Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Brown, R.
Danesh‐Naruie, G.
and
Hardy, J. P. L.
1976.
Topological groupoids: II. Covering morphisms and G‐spaces.
Mathematische Nachrichten,
Vol. 74,
Issue. 1,
p.
143.
Taylor, John
1988.
Quotients of groupoids by an action of a group.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 103,
Issue. 2,
p.
239.
Mucuk, Osman
and
Icen, Ilhan
2003.
Holonomy, extendibility, and the star universal cover of a topological groupoid.
Applied General Topology,
Vol. 4,
Issue. 1,
p.
79.
Mucuk, O.
Kılıçarslan, B.
Şahan, T.
and
Alemdar, N.
2011.
Group-groupoids and monodromy groupoids.
Topology and its Applications,
Vol. 158,
Issue. 15,
p.
2034.
Mucuk, Osman
Şahan, Tunçar
and
Alemdar, Nazmiye
2015.
Normality and Quotients in Crossed Modules and Group-groupoids.
Applied Categorical Structures,
Vol. 23,
Issue. 3,
p.
415.
Roberts, David Michael
2016.
A bigroupoid’s topology (or, Topologising the homotopy bigroupoid of a space).
Journal of Homotopy and Related Structures,
Vol. 11,
Issue. 4,
p.
923.
Virk, Žiga
and
Zastrow, Andreas
2017.
A new topology on the universal path space.
Topology and its Applications,
Vol. 231,
Issue. ,
p.
186.
AKIZ, H. Fulya
2018.
Covering Morphisms of Local Topological Group-Groupoids.
Proceedings of the National Academy of Sciences, India Section A: Physical Sciences,
Vol. 88,
Issue. 4,
p.
603.
Alemdar, Nazmiye
2019.
Topolojik R-Modül Grupoid Örtüleri.
Süleyman Demirel Üniversitesi Fen Bilimleri Enstitüsü Dergisi,
Vol. 23,
Issue. 1,
p.
272.
Mucuk, Osman
and
Şahan, Tunçar
2019.
Quotients of monodromy groupoids.
Vol. 2091,
Issue. ,
p.
030028.
Akız, H. Fulya
and
Mucuk, Osman
2019.
Topological aspect of monodromy groupoid for a topological internal groupoid.
Vol. 2183,
Issue. ,
p.
030005.
Mucuk, Osman
Demir, Serap
and
Şahan, Tunçar
2019.
Crossed module aspects of monodromy groupoids for topological internal groupoids.
Vol. 2183,
Issue. ,
p.
030012.
Mucuk, Osman
Demir, Serap
and
Şahan, Tunçar
2019.
Group-groupoids and crossed module aspects of monodromy groupoids.
Vol. 2091,
Issue. ,
p.
030027.
MUCUK, Osman
and
AKIZ, Hürmet Fulya
2020.
Covering morphisms of topological internal groupoids.
Hacettepe Journal of Mathematics and Statistics,
Vol. 49,
Issue. 3,
p.
1020.
Pakdaman, Ali
and
Shahini, Freshte
2021.
The fundamental groupoid as a topological groupoid: Lasso topology.
Topology and its Applications,
Vol. 302,
Issue. ,
p.
107838.
ÖZCAN, Abdullah Fatih
and
İÇEN, İlhan
2022.
Topological Group-Groupoids and Equivalent Categories.
Yüzüncü Yıl Üniversitesi Fen Bilimleri Enstitüsü Dergisi,
Vol. 27,
Issue. 3,
p.
667.
Chatterjee, Saikat
and
Koushik, Praphulla
2023.
Extension of Topological Groupoids and Hurewicz Morphisms.
Applied Categorical Structures,
Vol. 31,
Issue. 5,
PAKDAMAN, Ali
and
SHAHİNİ, Fereshteh
2023.
Topological fundamental groupoids: Brown's topology.
Hacettepe Journal of Mathematics and Statistics,
Vol. 52,
Issue. 4,
p.
896.
Pakdaman, Ali
2025.
On the topology of topological fundamental groupoids.
Applied General Topology,
Vol. 26,
Issue. 1,
p.
241.
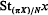 which is the fibre of the initial point map ∂′: (πX)/N→X over x in X, is the usual covering space
which is the fibre of the initial point map ∂′: (πX)/N→X over x in X, is the usual covering space  of X determined by the normal subgroup N{x} of the fundamental group π(X, x).
of X determined by the normal subgroup N{x} of the fundamental group π(X, x).

