Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-29T00:37:43.809Z Has data issue: false hasContentIssue false
  • English
  • Français

Examples of multiparameter CCR flows with non-trivial index

Part of: Selfadjoint operator algebras

Published online by Cambridge University Press:  27 September 2022

Piyasa Sarkar  and
S. Sundar
Piyasa Sarkar
Affiliation:
Institute of Mathematical Sciences, A CI of Homi Bhabha National Institute, CIT Campus, Taramani, Chennai 600113, India ([email protected]; [email protected])
S. Sundar
Affiliation:
Institute of Mathematical Sciences, A CI of Homi Bhabha National Institute, CIT Campus, Taramani, Chennai 600113, India ([email protected]; [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we construct uncountably many examples of multiparameter CCR flows, which are not pullbacks of $1$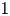-parameter CCR flows, with any given index. Moreover, the constructed CCR flows are type I in the sense that the associated product system is the smallest subsystem containing its units.

Keywords

E0-semigroupsCCR flowsIndex

MSC classification

Primary: 46L55: Noncommutative dynamical systems
Secondary: 46L99: None of the above, but in this section
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 65 , Issue 3 , August 2022 , pp. 799 - 832
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arjunan, A., Srinivasan, R. and Sundar, S., $E$-semigroups over closed convex cones, J. Operator Theory 84 (2) (2020), 289–322.CrossRefGoogle Scholar
Arjunan, A. and Sundar, S., CCR flows associated to closed convex cones, Münster J. Math. 13(2020), 115–143.Google Scholar
Arveson, W., Continuous analogues of Fock space, Mem. Amer. Math. Soc. 80 (409) (1989).Google Scholar
Arveson, W., Noncommutative dynamics and E-semigroups, Springer Monographs in Mathematics (Springer-Verlag, New York, 2003).CrossRefGoogle Scholar
Borel, A., Semisimple groups and Riemannian symmetric spaces, Texts and Readings in Mathematics, Volume 16 (Hindustan Book Agency, New Delhi, 1998).CrossRefGoogle Scholar
Faraut, J. and Korányi, A., Analysis on symmetric cones (Oxford Mathematical Monographs, 1994).Google Scholar
Hilgert, J., Hofmann, K. H. and Lawson, J. D., Lie groups, convex cones, and semigroups, Oxford Mathematical Monographs, (Oxford Science Publications, 1989).Google Scholar
Hilgert, J. and Neeb, K.-H., Lie semigroups and their applications, Lecture Notes in Mathematics, Volume 1552, Springer-Verlag, Berlin, 1993.CrossRefGoogle Scholar
Hilgert, J. and Neeb, K.-H., Wiener-Hopf operators on ordered homogeneous spaces. I, J. Funct. Anal. 132(1995), 86–118.CrossRefGoogle Scholar
Izumi, M. and Srinivasan, R., Generalized CCR flows, Comm. Math. Phys. 281 (2) (2008), 529–571.CrossRefGoogle Scholar
Izumi, M. and Srinivasan, R., Toeplitz CAR flows and type I factorizations, Kyoto J. Math. 50 (1) (2010), 1–32.CrossRefGoogle Scholar
Laca, M. and Raeburn, I., Extending multipliers from semigroups, Proc. Amer. Math. Soc. 123 (2) (1995), 355–362.CrossRefGoogle Scholar
Liebscher, V., Random sets and invariants for (type II) continuous tensor product systems of Hilbert spaces, Mem. Amer. Math. Soc. 199 (930) (2009).Google Scholar
Murugan, S.P. and Sundar, S., On the existence of $E_0$-semigroups – the multiparameter case, Infin. Dimens. Anal. Quantum Probab. Relat. Top. 21 (2) (2018), 185007–185027.CrossRefGoogle Scholar
Powers, R. T., A nonspatial continuous semigroup of $*$-endomorphisms of $B(H)$, Publ. Res. Inst. Math. Sci. 23 (6) (1987), 1053–1069.CrossRefGoogle Scholar
Powers, R. T., New examples of continuous spatial semigroups of $*$-endomorphisms of $B(H)$, Internat. J. Math. 10 (2) (1999), 215–288.CrossRefGoogle Scholar
Shalit, O. and Skeide, M. CP-semigroups and dilations, SubProduct systems and SuperProduct systems: The Multiparameter Case and Beyond, e-print https://arxiv/math.OA:2003.05166.Google Scholar
Shalit, O. M., $E_0$-dilation of strongly continuous $CP_0$-semigroups, J. Funct. Anal. 255 (1) (2008), 46–89.CrossRefGoogle Scholar
Shalit, O. M., What type of dynamics arise in $E_0$-dilations of commuting quantum Markov semigroups?, Infin. Dimens. Anal. Quantum. Probab. Relat. Top. 11 (3) (2008), 393–403.CrossRefGoogle Scholar
Shalit, O. M., $E$-dilation of strongly commuting $CP$-semigroups (the nonunital case), Houston J. Math. 37 (1) (2011), 203–232.Google Scholar
Shalit, O. M. and Solel, B., Subproduct systems, Doc. Math. 14(2009), 801–868.Google Scholar
Srinivasan, R., CCR and CAR flows over convex cones, e-print https://arxiv/math.OA:1908.00188v1.Google Scholar
Sundar, S., Notes on $E_0$-semigroups, available online at www.imsc.res.in/ssundar.Google Scholar
Sundar, S., $C^{*}$-algebras associated to topological ore semigroups, Münster J. Math. 9 (1) (2016), 155–185.Google Scholar
Sundar, S., Arveson's characterisation of CCR flows: the multiparameter case, J. Funct. Anal. 280 (1) (2021),108802–108846.CrossRefGoogle Scholar
Tsirelson, B., From random sets to continuous tensor products: answers to three questions of W. Arveson, e-print arxiv/math.FA:0001070.Google Scholar
Tsirelson, B., Non-isomorphic product systems. Advances in quantum dynamics,Contemp. Math., Amer. Math. Soc., Providence, RI 335 (2003) 273–328.Google Scholar
Williams, D. P., Crossed products of $C^{*}$-algebras, Mathematical Surveys and Monographs, Volume 134 (Amer. Math. Soc., Providence, RI, 2007).CrossRefGoogle Scholar