Article contents
The Equation of Telegraphy
Published online by Cambridge University Press: 20 January 2009
Extract
The equation of the propagation of electric signals along cables, generally known as the equation of telegraphy, may be written
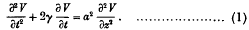
Particular solutions of this equation, adapted to various purposes have been found by Heaviside, Poincaré, A. G. Webster, T. W. Chaundy, § and others. The object of the present paper is to unify the theory of the equation by exhibiting the relations which these solutions bear to each other, and by obtaining them as particular cases of a general solution. The derivation of new particular solutions by the solution of integral equations is also discussed.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1923
References
* Electrical Papers, Vol, I., pp. 53 et seq. “On the Extra Current.”
† Comptes Rendus, 117 (1893), pp. 1027 et seq. “On the Propagation of Electricity.”
‡ “Electricity and Magnetism,” pp. 540 et seq.
§ Proc. London Math. Soc. (2) XXI. (1922), pp. 214–234.
* Cf. Riemann, Ges. Werke (1876), pp. 158 et seq.; or Darboux, Théorie Génerale des Surfaces, t. II., pp. 75 et seq.
* Nouveaux Mémoires de l'Acad. des Science, t. III.
- 1
- Cited by


