Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shulman, V. S.
1997.
Factorization of completely positive cocycles and the GNS construction of representations in pontryagin spaces.
Functional Analysis and Its Applications,
Vol. 31,
Issue. 3,
p.
221.
Шульман, Виктор Семенович
and
Shulman, Viktor Semenovich
1997.
Факторизация вполне положительных коциклов и ГНС-конструкция представлений в пространствах Понтрягина.
Функциональный анализ и его приложения,
Vol. 31,
Issue. 3,
p.
91.
Kissin, Edward
and
Shulman, Victor S
1998.
Differential Banach *-Algebras of Compact Operators Associated with Symmetric Operators.
Journal of Functional Analysis,
Vol. 156,
Issue. 1,
p.
1.
Badea, C.
1998.
The Stable Rank of Topological Algebras and a Problem of R. G. Swan.
Journal of Functional Analysis,
Vol. 160,
Issue. 1,
p.
42.
Kissin, Edward
and
Shulman, Victor S.
1999.
Dual spaces and isomorphisms of some differential Banach ∗-algebras of operators.
Pacific Journal of Mathematics,
Vol. 190,
Issue. 2,
p.
329.
Kissin, Edward
and
Shulman, Victor
2006.
LIPSCHITZ FUNCTIONS ON HERMITIAN BANACH *-ALGEBRAS.
The Quarterly Journal of Mathematics,
Vol. 57,
Issue. 2,
p.
215.
Kissin, Edward
Shulman, Victor S.
and
Turowska, Lyudmila B.
2007.
The Extended Field of Operator Theory.
Vol. 171,
Issue. ,
p.
225.
Gröchenig, Karlheinz
and
Klotz, Andreas
2010.
Noncommutative Approximation: Inverse-Closed Subalgebras and Off-Diagonal Decay of Matrices.
Constructive Approximation,
Vol. 32,
Issue. 3,
p.
429.
Klotz, Andreas
2012.
Spectral invariance of Besov–Bessel subalgebras.
Journal of Approximation Theory,
Vol. 164,
Issue. 2,
p.
268.
Shin, Chang Eon
and
Sun, Qi-yu
2013.
Wiener’s lemma: localization and various approaches.
Applied Mathematics-A Journal of Chinese Universities,
Vol. 28,
Issue. 4,
p.
465.
BHATT, SUBHASH J
KARIA, DINESH J
and
SHAH, MEETAL M
2013.
On a class of smooth Frechet subalgebras of C * -algebras.
Proceedings - Mathematical Sciences,
Vol. 123,
Issue. 3,
p.
393.
Sun, Qiyu
2014.
Localized nonlinear functional equations and two sampling problems in signal processing.
Advances in Computational Mathematics,
Vol. 40,
Issue. 2,
p.
415.
Motee, Nader
and
Sun, Qiyu
2014.
Sparsity measures for spatially decaying systems.
p.
5459.
BHATT, S. J.
and
SHAH, MEETAL M.
2016.
SECOND-ORDER NONCOMMUTATIVE DIFFERENTIAL AND LIPSCHITZ STRUCTURES DEFINED BY A CLOSED SYMMETRIC OPERATOR.
Journal of the Australian Mathematical Society,
Vol. 100,
Issue. 2,
p.
145.
BHATT, SUBHASH J
2016.
Smooth Frechet subalgebras of C ∗ -algebras defined by first order differential seminorms.
Proceedings - Mathematical Sciences,
Vol. 126,
Issue. 1,
p.
125.
Sun, Qiyu
and
Tang, Wai-Shing
2017.
Nonlinear Frames and Sparse Reconstructions in Banach Spaces.
Journal of Fourier Analysis and Applications,
Vol. 23,
Issue. 5,
p.
1118.
Motee, Nader
and
Sun, Qiyu
2017.
Sparsity and Spatial Localization Measures for Spatially Distributed Systems.
SIAM Journal on Control and Optimization,
Vol. 55,
Issue. 1,
p.
200.
Shin, Chang Eon
and
Sun, Qiyu
2019.
Polynomial control on stability, inversion and powers of matrices on simple graphs.
Journal of Functional Analysis,
Vol. 276,
Issue. 1,
p.
148.
Samei, Ebrahim
and
Shepelska, Varvara
2019.
Norm-Controlled Inversion in Weighted Convolution Algebras.
Journal of Fourier Analysis and Applications,
Vol. 25,
Issue. 6,
p.
3018.
Shin, Chang Eon
and
Sun, Qiyu
2020.
Operator Theory, Operator Algebras and Their Interactions with Geometry and Topology.
Vol. 278,
Issue. ,
p.
467.
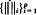 on B it defines
on B it defines 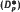 -subalgebras of A and establishes that they are locally normal Q*-subalgebras. If x = x* ∈ B and f(t) is a function on Sp(x), some sufficient conditions are given for f(x) to belong to B. For p = 1, in particular, it is shown that
-subalgebras of A and establishes that they are locally normal Q*-subalgebras. If x = x* ∈ B and f(t) is a function on Sp(x), some sufficient conditions are given for f(x) to belong to B. For p = 1, in particular, it is shown that 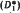 -subalgebras are closed under C∞-calculus. If δ is a closed derivation of A, the algebras D(δp) are
-subalgebras are closed under C∞-calculus. If δ is a closed derivation of A, the algebras D(δp) are 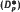 -subalgebras of A. In the case when δ is a generator of a one-parameter semigroup of automorphisms of A, it is proved that, in fact, D(δp) are
-subalgebras of A. In the case when δ is a generator of a one-parameter semigroup of automorphisms of A, it is proved that, in fact, D(δp) are 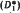 -subalgebras. The paper also characterizes those Banach *-algebras which are isomorphic to
-subalgebras. The paper also characterizes those Banach *-algebras which are isomorphic to 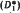 subalgebras of C*-algebras.
subalgebras of C*-algebras.

