Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-27T09:46:43.787Z Has data issue: false hasContentIssue false
  • English
  • Français

Defining Coarsenings of Valuations

Part of: Topological fields General field theory Connections with logic Model theory Arithmetic rings and other special rings

Published online by Cambridge University Press:  10 January 2017

Franziska Jahnke  and
Jochen Koenigsmann
Franziska Jahnke
Affiliation:
Institut für Mathematische Logik, Einsteinstrasse 62, 48149 Münster, Germany ([email protected])
Jochen Koenigsmann
Affiliation:
Mathematical Institute, Radcliffe Observatory Quarter, University of Oxford, Woodstock Road, Oxford OX2 6GG, UK ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the question of which Henselian fields admit definable Henselian valuations (with or without parameters). We show that every field that admits a Henselian valuation with non-divisible value group admits a parameter-definable (non-trivial) Henselian valuation. In equicharacteristic 0, we give a complete characterization of Henselian fields admitting a parameter-definable (non-trivial) Henselian valuation. We also obtain partial characterization results of fields admitting 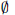-definable (non-trivial) Henselian valuations. We then draw some Galois-theoretic conclusions from our results.

Keywords

valuationsHenselian valued fieldsdefinable valuations

MSC classification

Secondary: 03C40: Interpolation, preservation, definability 12J10: Valued fields 03C60: Model-theoretic algebra 12L12: Model theory 12E30: Field arithmetic 13F30: Valuation rings
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 60 , Issue 3 , August 2017 , pp. 665 - 687
Copyright
Copyright © Edinburgh Mathematical Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Anscombe, W. and Koenigsmann, J., An existential -definition of Fq [[t]] in Fq ((t)), J. Symb. Logic 79(4) (2014), 1336–1343.CrossRefGoogle Scholar
2. Arason, J. K., Elman, R. and Jacob, B., Rigid elements, valuations, and realization of Witt rings, J. Alg. 110(2) (1987), 449–467.Google Scholar
3. Cherlin, G., van den Dries, L. and Macintyre, A., The elementary theory of regularly closed fields, Preprint (1980).Google Scholar
4. Cluckers, R. and Halupczok, I., Quantifier elimination in ordered abelian groups, Confluentes Math. 3(4) (2011), 587–615.Google Scholar
5. Cluckers, R., Derakhshan, J., Leenknegt, E. and Macintyre, A., Uniformly defining valuation rings in Henselian valued fields with finite or pseudo-finite residue fields, Annals Pure Appl. Logic 164(12) (2013), 1236–1246.Google Scholar
6. Delon, F. and Farré, R., Some model theory for almost real closed fields, J. Symb. Logic 61(4) (1996), 1121–1152.Google Scholar
7. Efrat, I., Valuations, orderings, and Milnor K-theory, Mathematical Surveys and Monographs, Volume 124 (American Mathematical Society, Providence, RI, 2006).Google Scholar
8. Engler, A. J. and Prestel, A., Valued fields, Springer Monographs in Mathematics (Springer, 2005).Google Scholar
9. Fehm, A., Existential -definability of Henselian valuation rings, J. Symb. Logic 80(1) (2015), 301–307.Google Scholar
10. Fehm, A. and Jahnke, F., On the quantifier complexity of definable canonical Henselian valuations, Math. Logic Q. 61(4–5) (2015), 347–361.Google Scholar
11. Giraudet, M., Cancellation and absorption of lexicographic powers of totally ordered abelian groups, Order 5(3) (1988), 275–287.Google Scholar
12. Hong, J., Definable non-divisible Henselian valuations, Bull. Lond. Math. Soc. 46(1) (2014), 14–18.Google Scholar
13. Jahnke, F. and Koenigsmann, J., Definable Henselian valuations, J. Symb. Logic 80(1) (2015), 85–99.Google Scholar
14. Jahnke, F. and Koenigsmann, J., Uniformly definining p-Henselian valuations, Annals Pure Appl. Logic 166(7–8) (2015), 741–754.CrossRefGoogle Scholar
15. Klingen, N., Elementar äquivalente Körper und ihre absolute Galoisgruppe, Arch. Math. 25 (1974), 604–612.Google Scholar
16. Koenigsmann, J., Definable valuations, Preprint (1994).Google Scholar
17. Koenigsmann, J., p-Henselian fields, Manuscr. Math. 87(1) (1995), 89–99.Google Scholar
18. Koenigsmann, J., Encoding valuations in absolute Galois groups, in Valuation theory and its applications Volume II, Fields Institute Communications, Volume 33, pp. 107–132 (American Mathematical Society, Providence, RI, 2003).Google Scholar
19. Koenigsmann, J., Elementary characterization of fields by their absolute Galois group, Sb. Adv. Math. 14(3) (2004), 16–42.Google Scholar
20. Marker, D., Model theory, Graduate Texts in Mathematics, Volume 217 (Springer, 2002).Google Scholar
21. Pas, J., Uniform p-adic cell decomposition and local zeta functions, J. Reine Angew. Math. 399 (1989), 137–172.Google Scholar
22. Prestel, A., Definable Henselian valuation rings, J. Symbolic Logic 80(4) (2015), 1260–1267.Google Scholar
23. Prestel, A. and Delzell, C. N., Mathematical logic and model theory: a brief introduction (expanded translation of the 1986 German original), Universitext (Springer, 2011).Google Scholar
24. Prestel, A. and Ziegler, M., Model-theoretic methods in the theory of topological fields, J. Reine Angew. Math. 299(300) (1978), 318–341.Google Scholar
25. Zakon, E., Generalized Archimedean groups, Trans. Am. Math. Soc. 99 (1961), 21–40.Google Scholar