Article contents
The closure of convergence sets for continued fractions are convergence sets
Published online by Cambridge University Press: 20 January 2009
Abstract
We prove that if Ω is a simple convergence set for continued fractions K(an/bn), then the closure  of Ω is also such a convergence set. Actually, we prove more: every continued fraction K(an/bn) has a “neighbourhood”
of Ω is also such a convergence set. Actually, we prove more: every continued fraction K(an/bn) has a “neighbourhood” 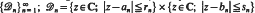 where rn>0 and sn>0, with the following property: Every continued fraction from {
where rn>0 and sn>0, with the following property: Every continued fraction from { n} converges if and only if K(an/bn) converges.
n} converges if and only if K(an/bn) converges.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 37 , Issue 1 , February 1994 , pp. 39 - 46
- Copyright
- Copyright © Edinburgh Mathematical Society 1994
References
REFERENCES
- 1
- Cited by


