Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-23T14:30:07.008Z Has data issue: false hasContentIssue false
  • English
  • Français

An example regarding Kalton's paper ‘isomorphisms between spaces of vector-valued continuous functions’

Part of: Topological linear spaces and related structures Linear function spaces and their duals

Published online by Cambridge University Press:  02 August 2021

Félix Cabello Sánchez
Félix Cabello Sánchez*
Affiliation:
Departamento de Matemáticas and IMUEx, Universidad de Extremadura, Avenida de Elvas, 06071Badajoz, Spain ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

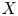The paper alluded to in the title contains the following striking result: Let $I$ be the unit interval and $\Delta$ the Cantor set. If $X$ is a quasi Banach space containing no copy of $c_{0}$ 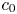which is isomorphic to a closed subspace of a space with a basis and $C(I,\,X)$ 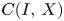is linearly homeomorphic to $C(\Delta ,\, X)$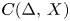, then $X$ 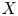is locally convex, i.e., a Banach space. We will show that Kalton result is sharp by exhibiting non-locally convex quasi Banach spaces $X$ 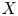with a basis for which $C(I,\,X)$ and $C(\Delta ,\, X)$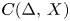 are isomorphic. Our examples are rather specific and actually, in all cases, $X$ is isomorphic to $C(K,\,X)$ if $K$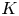 is a metric compactum of finite covering dimension.

Keywords

spaces of vector-valued continuous functionsquasi Banach spaces

MSC classification

Primary: 46A16: Not locally convex spaces (metrizable topological linear spaces, locally bounded spaces, quasi-Banach spaces, etc.)
Secondary: 46E10: Topological linear spaces of continuous, differentiable or analytic functions
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 64 , Issue 3 , August 2021 , pp. 615 - 619
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Cabello Sánchez, F., Castillo, J. M. F. and Moreno, Y., On the bounded approximation property on subspaces of $\ell _p$ when $0 < p < 1$ and related issues, Forum Math. 31 (2019), 1–24.CrossRefGoogle Scholar
Cabello Sánchez, F., Garbulińska-Wȩgrzyn, J. and Kubiś, W., Quasi-Banach spaces of almost universal disposition, J. Funct. Anal. 267 (2014), 744–771.CrossRefGoogle Scholar
Caponetti, D. and Lewicki, G., A note on the admissibility of modular function spaces, J. Math. Anal. Appl. 448 (2017), 1331–1342.10.1016/j.jmaa.2016.11.047CrossRefGoogle Scholar
Cauty, R., Un espace métrique linéaire qui n'est pas un rétracte absolu, Fund. Math. 146 (1994), 85–99.CrossRefGoogle Scholar
Ishii, J., On the admissibility of function spaces, J. Fac. Sci., Hokkaido Univ., Ser. 1 19 (1965), 49–55.Google Scholar
Kalton, N. J., Universal spaces and universal bases in metric linear spaces, Studia Math. 61 (1977), 161–191.CrossRefGoogle Scholar
Kalton, N. J., Transitivity and quotients of Orlicz spaces, Comment. Math. (1978), 159–172. (Special issue in honor of the 75th birthday of W. Orlicz)Google Scholar
Kalton, N. J., Isomorphisms between spaces of vector-valued continuous functions, Proc. Edinburgh Math. Soc. 26 (1983), 29–48.CrossRefGoogle Scholar
Kalton, N. J. and Dobrowolski, T., Cauty's space enhanced, Topology Appl. 159 (2012), 28–33.Google Scholar
Klee, V., Leray–Schauder theory without local convexity, Math. Ann. 141 (1960), 286–296.CrossRefGoogle Scholar
Michael, E., Continuous selections II, Ann. Math. (2) 64 (1956), 562–580.CrossRefGoogle Scholar
Shuchat, A. H., Approximation of vector-valued continuous functions, Proc. Amer. Math. Soc. 31 (1972), 97–103.CrossRefGoogle Scholar
Waelbroeck, L., Topological vector spaces, Summer school on topological vector spaces, Bruxelles 1972, Springer Lecture Notes in Mathematics, Volume 331, pp. 1–40 (Berlin-Heidelberg-New York, 1973).CrossRefGoogle Scholar