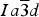Crystal structure of morimotoite from Ice River, Canada

Abstract

The crystal structure of a morimotoite garnet, ideally Ca₃(Ti⁴⁺Fe²⁺)Si₃O₁₂, from the Ice River alkaline complex, British Columbia, Canada was refined by the Rietveld method, space group $Ia\overline 3 d$ , and monochromatic synchrotron high-resolution powder X-ray diffraction (HRPXRD) data. Electron-microprobe analysis indicates a homogeneous sample with a formula {Ca_2.91Mg_0.05Mn²⁺ _0.03}_Σ3[Ti_1.09Fe³⁺ _0.46Fe²⁺ _0.37Mg_0.08]_Σ2(Si_2.36Fe³⁺ _0.51Al_0.14)_Σ3O₁₂. The HRPXRD data show a two-phase intergrowth. The reduced χ ² and overall R(F ²) Rietveld refinement values are 1.572 and 0.0544, respectively. The weight percentage, unit-cell parameter (Å), distances (Å), and site occupancy factors (sofs) for phase-1 are as follows: 76.5(1)%, a = 12.156 98(1) Å, average <Ca–O> = 2.4383, Ti–O = 2.011(1), Si–O = 1.693(1) Å, Ca(sof) = 0.943(2), Ti(sof) = 0.966(2), and Si(sof) = 1.095(3). The corresponding values for phase-2 are 23.5(1)%, a = 12.160 67(2) Å, average <Ca–O> = 2.452, Ti–O = 1.988(3), Si–O = 1.704(3) Å, Ca(sof) = 1.063(7), Ti(sof) = 1.187(7), and Si(sof) = 1.220(8). The two phases cause strain that arises from structural mismatch and gives rise to low optical anisotropy. Because the two phases are structurally quite similar, a refinement using a single-phase model with anisotropic displacement parameters shows no unusual displacement ellipsoid for the O atom that requires a “split O-atom position”, as was done in previous studies.

I. INTRODUCTION

Peterson et al. (1995) observed unusual and large anisotropic displacement parameters for the O atom on a single position for morimotoite garnet from Ice River. So, they modeled a split position for the O atom and used isotropic displacement parameters. The reason for these unusual displacement parameters was attributed to different size Si and Fe atoms on the tetrahedral site. Attempts were made to show that the SiO₄ and the O₄H₄ tetrahedra in hydrogarnets have different sizes by also using a “split O-atom position” model, which was also used to explain the unusual anisotropic displacement ellipsoid of the O atom that elongate along the “Si–O” bond instead of right angles to this bond direction (e.g. Figure 2 in Armbruster, 1995; Figure 2 in Ferro et al., 2003). These unusual features of the O-atom displacement ellipsoid may be the result of multi-phase intergrowths and are investigated in this study with regard to morimotoite and have implications for hydrogarnets.

Recently, we proposed that a multi-phase intergrowth of two or three cubic phases with slightly different structural (and chemical) parameters gives rise to strain arising from structural mismatch, and that this strain is the cause of the anisotropy in cubic garnets (Antao, 2013a, 2013b, 2013c; Antao and Klincker, 2013a, 2013b; Antao and Round, 2014). This explanation is tested further in this study for morimotoite because Ti-rich andradites are known to be birefringent, but their structures were refined in the cubic space group (e.g. Armbruster et al., 1998).

The crystal structure of many members of the garnet group have been refined (e.g. Novak and Gibbs, 1971; Lager et al., 1989; Armbruster and Geiger, 1993). The general formula for garnet is ^[8]X₃ ^[6]Y₂ ^[4]Z₃ ^[4]O₁₂, Z=8, space group $Ia\overline 3 d$ , where the eight-coordinated dodecahedral X site contains Mg²⁺, Ca²⁺, Mn²⁺, or Fe²⁺ cations, the six-coordinated octahedral Y site contains Al³⁺, Cr³⁺, Fe³⁺, Ti⁴⁺, or Zr⁴⁺ cations, and the four-coordinated tetrahedral Z site contains Si⁴⁺, Fe³⁺, or Al³⁺ cations, or (O₄H₄) (e.g. Armbruster et al., 1998). The structure of garnet consists of alternating ZO₄ tetrahedra and YO₆ octahedra with X atoms filling cavities to form XO₈ dodecahedra. The eight O atoms in the XO₈ dodecahedron occur at the corners of a distorted cube.

This study examines the crystal structure of a morimotoite, {Ca_2.91Mg_0.05Mn²⁺ _0.03}_Σ3[Ti_1.09Fe³⁺ _0.46Fe²⁺ _0.37Mg_0.08]_Σ2(Si_2.36Fe³⁺ _0.51Al_0.14)_Σ3O₁₂, from Ice River using high-resolution powder X-ray diffraction (HRPXRD) data that show a two-phase intergrowth that causes strain, which gives rise to low optical anisotropy. Moreover, a refinement of a single-phase model with anisotropic displacement parameters shows no unusual features for the O atom that requires a “split O-atom position” model.

II. EXPERIMENTAL

A. Sample characterization and electron-microprobe analysis (EMPA)

The black morimotoite sample is from the Ice River alkaline intrusive complex, Yoho National Park, British Columbia, Canada. The same sample was studied by Locock et al. (1995) and Peterson et al. (1995). A thin section of the sample shows weak optical anisotropy under cross-polarized light.

The morimotoite sample was analyzed with a JEOL JXA-8200 wavelength-dispersive (WD)–ED electron-microprobe analyzer. The JEOL operating program on a Solaris platform was used for ZAF (atomic number, absorption, fluorescence) correction and data reduction. The WD operating conditions were 15 kV, 20 nA, 5 μm beam diameter, and using various standards [almandine-pyrope (MgKα), grossular (CaKα), almandine (FeKα, AlKα, SiKα), rutile (TiKα), spessartine (MnKα), and chromite (CrKα)]. The sample appeared homogeneous based on optical observations and EMPA results of eight spots from different areas of a crystal with a diameter of about 2 mm. The EMPA data were analyzed using the spreadsheet developed by Locock (2008) and the average chemical composition is given (Table I).

Table I. EMPA results for morimotoite.

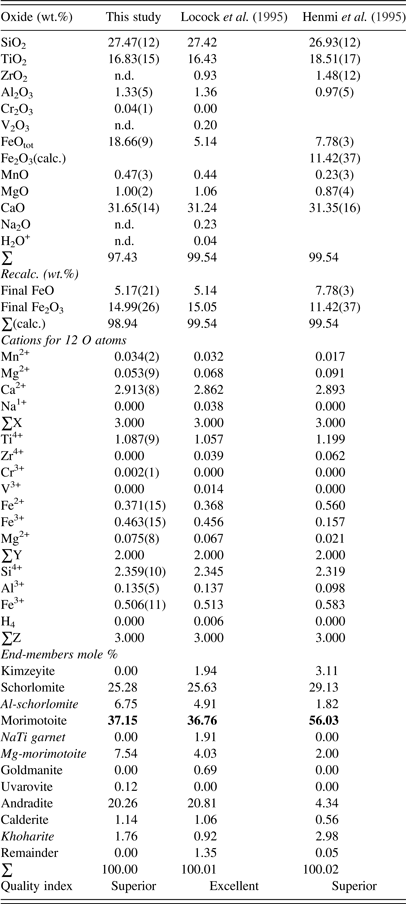
Numbers in bold indicate the dominant end-member morimotoite, ideally Ca₃(Ti⁴⁺Fe²⁺)Si₃O₁₂, so all three samples are called morimotoite.

B. Synchrotron HRPXRD

The morimotoite sample was studied by HRPXRD that was performed at beamline 11-BM, Advanced Photon Source, Argonne National Laboratory. A small fragment (about 2 mm in diameter) of the sample was crushed to a fine powder using an agate mortar and pestle. The crushed sample was loaded into a Kapton capillary (0.8 mm internal diameter) and rotated during the experiment at a rate of 90 rotations per second. The data were collected at 23 °C to a maximum 2θ of about 50 with a step size of 0.001° and a step time of 0.1 s per step. The HRPXRD trace was collected with 12 silicon (111) crystal analyzers that reduce the angular range to be scanned and allow rapid acquisition of data. A silicon (NIST 640c) and alumina (NIST 676a) standard (ratio of ⅓ Si:⅔ Al₂O₃ by weight) was used to calibrate the instrument and refine the monochromatic wavelength used in the experiment (see Table II). Additional details of the experimental setup are given elsewhere (Antao et al., 2008; Lee et al., 2008; Wang et al., 2008).

Table II. HRPXRD data and Rietveld refinement statistics for morimotoite.

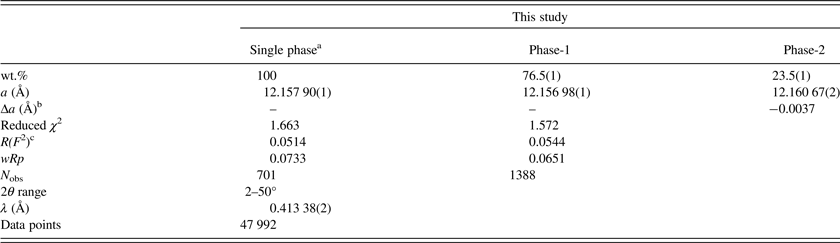
^aBased on anisotropic displacement parameters.

^bThe strain and birefringence are proportional to Δa = (a _{dominant phase}−a _{minor phase}) (Kitamura and Komatsu, 1978).

^c R(F ²) = overall R-structure factor based on observed and calculated structure amplitudes = [∑(F _o ² −F _c ²)/∑(F _o ²)]^1/2.

C. Rietveld structure refinements

The HRPXRD data were analyzed by the Rietveld (1969) method, as implemented in the GSAS program (Larson and Von Dreele, 2000), and using the EXPGUI interface (Toby, 2001). Scattering curves for neutral atoms were used. The starting atom coordinates, cell parameter, and space group, $Ia\overline 3 d$ , were taken from Peterson et al. (1995). The background was modeled using a Chebyschev polynomial (eight terms). The reflection-peak profiles were fitted using type-3 profile in the GSAS program. A full-matrix least-squares refinement was carried out by varying the parameters in the following sequence: a scale factor, unit-cell parameter, atom coordinates, and isotropic displacement parameters. Examination of the HRPXRD trace for morimotoite shows the presence of two separate phases with slightly different unit-cell parameters (Figures 1 and 2). Both the HRPXRD trace and the Rietveld refinement statistics indicate that the two-phase model is preferred over the single-phase model (Table II). The two separate phases were refined together with the site occupancy factors (sofs) in terms of the dominant Ca, Ti, and Si atoms in the corresponding X, Y, and Z sites. Toward the end of the refinement, all the parameters were allowed to vary simultaneously, and the refinement proceeded to convergence. A single-phase model was also refined using anisotropic displacement parameters to check for unusual O-atom features that were reported by Peterson et al. (1995).

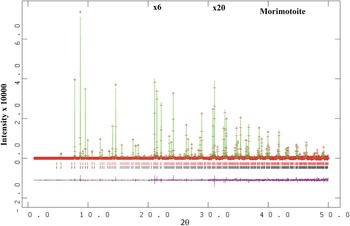
Figure 1. (Color online) HRPXRD trace for morimotoite (two-phase model) together with the calculated (continuous line) and observed (crosses) profiles. The difference curve (I _obs −I _calc) is shown at the bottom. The short vertical lines indicate allowed reflection positions. The intensities for the trace and difference curve that are above 20° and 30° 2θ are multiplied by factors of 6 and 20, respectively.

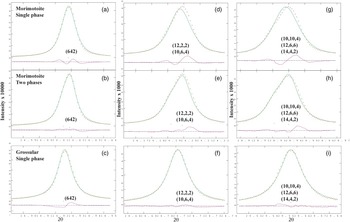
Figure 2. (Color online) A comparison of the same reflections in morimotoite and grossular. Parts (a), (d), and (g) are morimotoite data fitted using a single phase. Parts (b), (e), and (h) are morimotoite data fitted using two phases. Parts (c), (f), and (i) are grossular data fitted using a single phase for comparison (Antao, 2013a). The two phases for morimotoite fit the data best and matches the left shoulder on the high-angle peaks. The peaks in grossular are narrower than those in morimotoite.

The cell parameters and the Rietveld refinement statistics for the single- and two-phase models are listed in Table II. Atom coordinates, isotropic displacement parameters, and sofs are given in Table III. Anisotropic displacement parameters for the single-phase model are given in Table IV. Bond distances are given in Table V.

Table III. Atom coordinates ^a , isotropic displacement parameters (U, Ų ) ^b , and sofs for morimotoite.

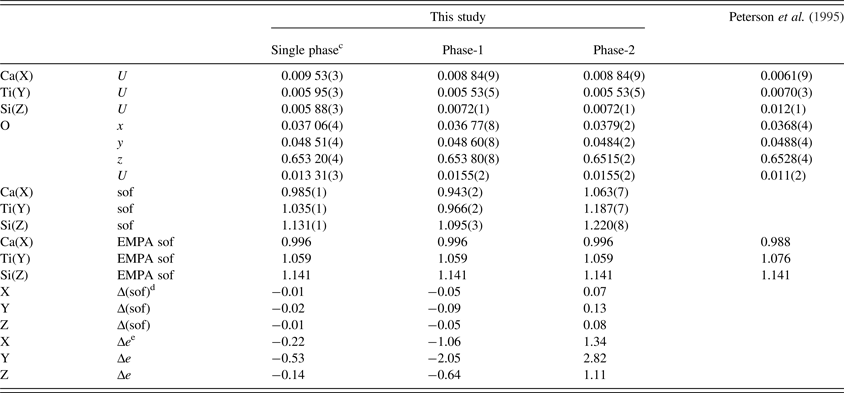
^a X at (0, ¼, ⅛), Y at (0, 0, 0), and Z at (⅜, 0, ¼). The O(sof) = 1 in all cases.

^b U parameter for the same site in phases 1 and 2 were constrained to be equal.

^cData are from a refinement using isotropic displacement parameters.

^dΔ(sof) = sof (HRPXRD refinement)−sof (EMPA).

^eΔe = electrons (HRPXRD refinement)−electrons (EMPA).

Table IV. Anisotropic displacement parameters (Ų) for single-phase morimotoite.

Table V. Selected distances (Å) and angle (°) for morimotoite.

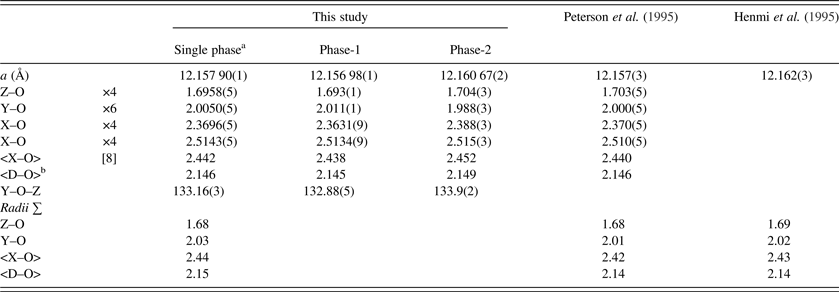
^aData are from a refinement using isotropic displacement parameters.

^b<D–O> = {(Z–O) + (Y–O) + (X–O) + (X′–O)}/4. For the calculated bond distances, the following radii from Shannon (1976) were used (X site: Mn²⁺ = 0.96, Mg²⁺ = 0.89 Å; Y site: Ti⁴⁺ = 0.605, Cr³⁺ = 0.615, Fe²⁺ = 0.78, Fe³⁺ = 0.645, Mg²⁺ = 0.72 Å; Z site: Si⁴⁺ = 0.26, Al³⁺ = 0.39, Fe³⁺ = 0.49 Å). Ca²⁺ = 1.06 Å instead of 1.12 Å; this gives more realistic <X–O> distances.

III. RESULTS AND DISCUSSION

The composition for the Ice River sample, {Ca_2.91Mg_0.05Mn²⁺ _0.03}_Σ3[Ti_1.09Fe³⁺ _0.46Fe²⁺ _0.37Mg_0.08]_Σ2(Si_2.36Fe³⁺ _0.51Al_0.14)_Σ3O₁₂, shows the distribution of the atoms in the three cation sites indicated by the general formula ^[8]X₃ ^[6]Y₂ ^[4]Z₃ ^[4]O₁₂ (Table I). Using the data from Locock et al. (1995) and recalculating the cation content using the spreadsheet from Locock (2008), their composition, {Ca_2.86Mg_0.07Na_0.04Mn²⁺ _0.03}_Σ3[Ti_1.06Fe³⁺ _0.46Fe²⁺ _0.37Mg_0.07Zr_0.04V_0.01]_Σ2(Si_2.35Fe³⁺ _0.51Al_0.14)_Σ3O₁₂, is similar to that obtained in this study, as expected (Table I). Similarly, the composition, {Ca_2.89Mg_0.09Mn²⁺ _0.02}_Σ3[Ti_1.20Fe³⁺ _0.16Fe²⁺ _0.56Mg_0.02Zr_0.06]_Σ2(Si_2.32Fe³⁺ _0.58Al_0.10)_Σ3O₁₂, was obtained for the type material from Japan (Henmi et al., 1995). The ideal end-member formula for morimotoite is Ca₃(Ti⁴⁺Fe²⁺)Si₃O₁₂, which is the dominant component in the chemical analysis, so all three samples are called morimotoite (Table I). The Ice River sample was previously called schorlomite (Peterson et al., 1995; Locock, 2008). However, the dominant component in Table I is morimotoite, which is the name used in this study and in Grew et al. (2013).

The reduced χ ² and overall R(F ²) Rietveld refinement values are 1.572 and 0.0544, respectively, for the two-phase model and they are similar to those for the single-phase model (Table II). The sofs obtained from the refinement are similar to those calculated from the EMPA results (Table III). The bond distances calculated from the atom radii are similar to those obtained from the Rietveld structure refinements (Table V).

The unit-cell parameter for the Ice River sample [a = 12.157 90(1) Å] is smaller than a = 12.162(3) (Å) for the type-material morimotoite from Japan (Henmi et al., 1995), for which no structural data are available. However, the bond distances calculated from the atom radii are similar to the other samples (Table V). The reason for the larger cell for the type material seems to be the larger amount of Fe²⁺ in the Y site and the slightly longer cation–O distances (Table V).

The single-crystal results from Peterson et al. (1995) using one O-atom position for the same morimotoite sample matches the single-phase HRPXRD results from this study (Table V). However, Peterson et al. (1995) observed unusual displacement parameters for the O atom with its displacement ellipsoid elongated along the “Si–O” bond. So, they modeled a split position for the O atom. Attempts were also made to show that the SiO₄ and the O₄H₄ tetrahedra in hydrogarnets have different sizes by using a “split O-atom position” model, which was used to explain the unusual displacement ellipsoid of the O atom that also elongates along the “Si–O” bond (e.g. Lager et al., 1987; Armbruster and Lager, 1989; Ferro et al., 2003).

A single-phase model for the Ice River sample was refined using anisotropic displacement parameters to test the “split O-atom position” hypothesis (Table IV). No unusual O-atom features were observed, as shown by the tetrahedral coordination of the Z site by O atoms (Figure 3). The displacement parameter for the Z site is about the same as that for the Y site and both are smaller than that for the X site (Table III). However, Peterson et al. (1995) obtained displacement parameters for the Z site that are larger than those of the X and Y sites, which is usual (Table III). It is possible that multi-phase intergrowths may give rise to unusual ellipsoids for the O atom, if the unit-cell parameters for the phases are quite different from each other (e.g. Koritnig et al., 1978; Antao, 2013c), which is not the case in this study.

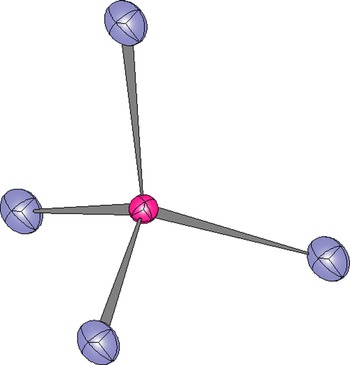
Figure 3. (Color online) Tetrahedral coordination of the Z site showing that the O atoms are not elongated along the “Si–O” bond direction, as was found by Peterson et al. (1995).

The sample used in this study may contain a two-phase intergrowth instead of a single phase. The same reflections are compared for: (1) a single-phase [Figures 2(a), 2(d), and 2(g)]; (2) a two-phase [Figures 2(b), 2(e), and 2(h)]; and (3) a single-phase grossular [Figures 2(c), 2(f), and 2(i); data from Antao, 2013a]. No significant differences are observed for the low-angle peak near 14.9°. However, from the two high-angle peaks [near 24° and 29° 2θ in Figures 2(e) and 2(h)], it appears that the Ice River sample is a two-phase intergrowth. The trace for grossular contains only one peak for each reflection [Figures 2(c), 2(f), and 2(i)]. The two phases in morimotoite have slightly different unit-cell parameters: a = 12.156 98(1) for phase-1 and a = 12.160 67(2) for phase-2. Although splitting of the reflections is difficult to observe in this study, they are easily observed in other studies (e.g. Koritnig et al., 1978; Antao, 2013c). The residuals in the difference curves probably indicate a small amount of a third morimotoite phase with a slightly smaller unit-cell parameter, which was not modeled (Figure 2).

Because the two phases are quite similar to each other, there are some correlations between the structural parameters in the two-phase refinement. Therefore, the isotropic displacement parameters for atoms in the same site were constrained to be equal to each other, and the profile parameters for both phases were also constrained to be the same. Because of the profile constraint, sample size and strain information could not be obtained for this sample. However, in other garnet samples, such information was obtained (Antao, 2013a, 2013c).

The crystal structure of morimotoite can be rationalized using bond-valence sums (BVS) calculated in valence units (v.u.) (Wills and Brown, 1999). For the isotropic refinement using a single phase (Table V), the BVS for the Ca atom at the X site is 2.26 v.u., so the Ca atom is a bit large for the X site. For Ti⁴⁺ at the Y site, the BVS is 3.59 v.u. and this site also contains Fe³⁺ and Fe²⁺ cations. The BVS for Si atom at the Z site is 3.44 v.u., which is reasonable because there are some Al and Fe²⁺ cations in this site (Table I). The BVS for the O atom is 1.95 instead of 2 v.u., which is reasonable.

It is possible that formation of a two-phase intergrowth of morimotoite in Si-deficient rocks may be related to changes in oxygen fugacity (f _O2), activity of SiO₂ (a _SiO2), etc., as the crystals grow at low temperature that prevents diffusion or homogenization of the cations. The two phases cause strain that arises from structural mismatch and gives rise to optical anisotropy, as was found in other garnets (Antao, 2013a, 2013b, 2013c; Antao and Klincker, 2013a, 2013b; Antao and Round, 2014). The two-phase intergrowth in morimotoite may be similar to epitaxial intergrowths because of the similarity of the structural and chemical parameters (Kitamura and Komatsu, 1978). However, the small differences between the two phases give rise to structural mismatch that results in strain and low optical anisotropy.