Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
van Douwen, Erik K.
1975.
Simultaneous linear extension of Continuous functions.
General Topology and its Applications,
Vol. 5,
Issue. 4,
p.
297.
Reed, G.M.
1975.
Studies in Topology.
p.
479.
Malykhin, V. I.
and
Ponomarev, V. I.
1977.
General topology (set-theoretic trend).
Journal of Soviet Mathematics,
Vol. 7,
Issue. 4,
p.
587.
Reed, G. M.
1986.
The intersection topology w.r.t. the real line and the countable ordinals.
Transactions of the American Mathematical Society,
Vol. 297,
Issue. 2,
p.
509.
Kholshchevnikova, N. N.
1991.
Representation 0f certain functions under martin's axiom.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 49,
Issue. 2,
p.
225.
Recław, Ireneusz
1993.
Restrictions to continuous functions and Boolean algebras.
Proceedings of the American Mathematical Society,
Vol. 118,
Issue. 3,
p.
791.
Hirschorn, James
2003.
Summable gaps.
Annals of Pure and Applied Logic,
Vol. 120,
Issue. 1-3,
p.
1.
Bartoszewicz, Artur
Gła̧b, Szymon
Pellegrino, Daniel
and
Seoane-Sepúlveda, Juan
2013.
Algebrability, non-linear properties, and special functions.
Proceedings of the American Mathematical Society,
Vol. 141,
Issue. 10,
p.
3391.
Gámez-Merino, José L.
and
Seoane-Sepúlveda, Juan B.
2013.
An undecidable case of lineability inRR.
Journal of Mathematical Analysis and Applications,
Vol. 401,
Issue. 2,
p.
959.
Bartoszewicz, Artur
Bienias, Marek
Filipczak, Małgorzata
and
Gła̧b, Szymon
2014.
Strongc-algebrability of strong Sierpiński–Zygmund, smooth nowhere analytic and other sets of functions.
Journal of Mathematical Analysis and Applications,
Vol. 412,
Issue. 2,
p.
620.
2015.
Lineability.
p.
229.
Ciesielski, K. C.
and
Seoane-Sepúlveda, J. B.
2019.
A century of Sierpiński–Zygmund functions.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 113,
Issue. 4,
p.
3863.
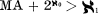 and three were independent of
and three were independent of 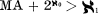 But the relation of the remaining eight propositions to
But the relation of the remaining eight propositions to 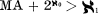 has been unsettled.
has been unsettled.

