1 Introduction
Let
![]() $G$
be a finite simple graph. We denote
$G$
be a finite simple graph. We denote
![]() $V=V(G)$
the vertex set of
$V=V(G)$
the vertex set of
![]() $G$
and
$G$
and
![]() $E(G)$
the edge set of
$E(G)$
the edge set of
![]() $G$
. Let
$G$
. Let
![]() $K$
be a field and
$K$
be a field and
![]() $S=K[V]$
the polynomial ring whose variables are identified with the vertices of
$S=K[V]$
the polynomial ring whose variables are identified with the vertices of
![]() $G$
. We consider the standard (multi-)grading on
$G$
. We consider the standard (multi-)grading on
![]() $K[V]$
. We can associate with
$K[V]$
. We can associate with
![]() $G$
the ideal of
$G$
the ideal of
![]() $S$
:
$S$
:
The ideal
![]() $I(G)$
is called the edge ideal of
$I(G)$
is called the edge ideal of
![]() $G$
. Let
$G$
. Let
![]() $J(G)$
be the Alexander dual ideal of
$J(G)$
be the Alexander dual ideal of
![]() $I(G)$
. Actually,
$I(G)$
. Actually,
![]() $J(G)$
is the cover ideal of
$J(G)$
is the cover ideal of
![]() $G$
, the ideal generated by all monomials which are products of the vertices of minimal vertex covers of
$G$
, the ideal generated by all monomials which are products of the vertices of minimal vertex covers of
![]() $G$
. The main theme of the study of these ideals is to investigate the relations between the ring properties of
$G$
. The main theme of the study of these ideals is to investigate the relations between the ring properties of
![]() $I(G)$
and
$I(G)$
and
![]() $J(G)$
and the combinatorics of
$J(G)$
and the combinatorics of
![]() $G$
. We are interested in characterizing homological invariants of these ideals. There are some results about this direction; see for example, [Reference Dochtermann and Engström3, Reference Hà and Van Tuyl5, Reference Katzman7–Reference Morey and Villarreal13, Reference Van Tuyl19, Reference Woodroofe21, Reference Zheng22] and the references therein.
$G$
. We are interested in characterizing homological invariants of these ideals. There are some results about this direction; see for example, [Reference Dochtermann and Engström3, Reference Hà and Van Tuyl5, Reference Katzman7–Reference Morey and Villarreal13, Reference Van Tuyl19, Reference Woodroofe21, Reference Zheng22] and the references therein.
A subset
![]() $C\subset V$
is called a vertex cover of
$C\subset V$
is called a vertex cover of
![]() $G$
if
$G$
if
![]() $C\cap e\neq \emptyset$
for each
$C\cap e\neq \emptyset$
for each
![]() $e\in E(G)$
. A vertex cover is said to be minimal if it has no proper subset which is also a vertex cover. A graph
$e\in E(G)$
. A vertex cover is said to be minimal if it has no proper subset which is also a vertex cover. A graph
![]() $G$
is called unmixed if all minimal vertex covers of
$G$
is called unmixed if all minimal vertex covers of
![]() $G$
have the same cardinality. When
$G$
have the same cardinality. When
![]() $G$
is unmixed and has no isolated vertex, it is known that
$G$
is unmixed and has no isolated vertex, it is known that
![]() $2\operatorname{height}I(G)\geqslant \#V$
. When the equality holds,
$2\operatorname{height}I(G)\geqslant \#V$
. When the equality holds,
![]() $G$
is called very well-covered. Note that the class of very well-covered graphs contains the class of unmixed bipartite graphs with no isolated vertex. Also the class of unmixed bipartite graphs contains the class of Cohen–Macaulay bipartite graphs. Here we say that a graph
$G$
is called very well-covered. Note that the class of very well-covered graphs contains the class of unmixed bipartite graphs with no isolated vertex. Also the class of unmixed bipartite graphs contains the class of Cohen–Macaulay bipartite graphs. Here we say that a graph
![]() $G$
is Cohen–Macaulay if the quotient ring
$G$
is Cohen–Macaulay if the quotient ring
![]() $S/I(G)$
is Cohen–Macaulay.
$S/I(G)$
is Cohen–Macaulay.
In general, it is hard to construct an explicit minimal free resolution of an ideal. But Herzog and Hibi [Reference Herzog and Hibi6] succeeded in constructing a resolution of
![]() $J(G)$
when
$J(G)$
when
![]() $G$
is a Cohen–Macaulay bipartite graph. Also Mohammadi and Moradi [Reference Mohammadi and Moradi12] investigated a resolution of
$G$
is a Cohen–Macaulay bipartite graph. Also Mohammadi and Moradi [Reference Mohammadi and Moradi12] investigated a resolution of
![]() $J(G)$
when
$J(G)$
when
![]() $G$
is an unmixed bipartite graph. The first main result of the present paper is a construction of an explicit minimal free resolution of
$G$
is an unmixed bipartite graph. The first main result of the present paper is a construction of an explicit minimal free resolution of
![]() $J(G)$
when
$J(G)$
when
![]() $G$
is a Cohen–Macaulay very well-covered graph (Theorem 3.2).
$G$
is a Cohen–Macaulay very well-covered graph (Theorem 3.2).
Using our first main result, we derive the characterization of the projective dimension of
![]() $S/I(G)$
over
$S/I(G)$
over
![]() $S$
, denoted by
$S$
, denoted by
![]() $\operatorname{pd}S/I(G)$
when
$\operatorname{pd}S/I(G)$
when
![]() $G$
is a very well-covered graph.
$G$
is a very well-covered graph.
Two edges
![]() $\{x_{1},y_{1}\},\{x_{2},y_{2}\}\in E(G)$
are said to be
$\{x_{1},y_{1}\},\{x_{2},y_{2}\}\in E(G)$
are said to be
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $G$
if there is no other edge in
$G$
if there is no other edge in
![]() $G$
between vertices
$G$
between vertices
![]() $x_{1},x_{2},y_{1},y_{2}$
. Let
$x_{1},x_{2},y_{1},y_{2}$
. Let
![]() ${\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}$
be a set of complete bipartite subgraphs of
${\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}$
be a set of complete bipartite subgraphs of
![]() $G$
. We set
$G$
. We set
![]() $V({\mathcal{B}})=V(B_{1})\cup \cdots \cup V(B_{r})$
. We say
$V({\mathcal{B}})=V(B_{1})\cup \cdots \cup V(B_{r})$
. We say
![]() ${\mathcal{B}}$
is pairwise
${\mathcal{B}}$
is pairwise
![]() $3$
-disjoint if
$3$
-disjoint if
![]() $V(B_{k})\cap V(B_{\ell })=\emptyset$
for any
$V(B_{k})\cap V(B_{\ell })=\emptyset$
for any
![]() $k\neq \ell$
and there exists
$k\neq \ell$
and there exists
![]() $e_{k}\in E(B_{k})$
for each
$e_{k}\in E(B_{k})$
for each
![]() $k=1,\ldots ,r$
such that
$k=1,\ldots ,r$
such that
![]() $e_{1},\ldots ,e_{r}$
are pairwise
$e_{1},\ldots ,e_{r}$
are pairwise
![]() $3$
-disjoint.
$3$
-disjoint.
Theorem 1.1. Let
![]() $G$
be a very well-covered graph. Then
$G$
be a very well-covered graph. Then
 $$\begin{eqnarray}\displaystyle & & \displaystyle \operatorname{pd}S/I(G)=\nonumber\\ \displaystyle & & \displaystyle \quad \max \left\{\#V({\mathcal{B}})-r:\begin{array}{@{}l@{}}{\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}\text{is a pairwise 3-disjoint set}\\ \text{of complete bipartite subgraphs of }G\end{array}\right\}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \operatorname{pd}S/I(G)=\nonumber\\ \displaystyle & & \displaystyle \quad \max \left\{\#V({\mathcal{B}})-r:\begin{array}{@{}l@{}}{\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}\text{is a pairwise 3-disjoint set}\\ \text{of complete bipartite subgraphs of }G\end{array}\right\}.\nonumber\end{eqnarray}$$
Herzog and Hibi [Reference Herzog and Hibi6] characterized Cohen–Macaulay bipartite graphs in terms of the original graph. Crupi et al. [Reference Crupi, Rinaldo and Terai2] expanded their result for very well-covered graphs. When
![]() $G$
is an unmixed bipartite graph, Kummini [Reference Kummini10, Proposition 3.2] gave a combinatorial characterization of
$G$
is an unmixed bipartite graph, Kummini [Reference Kummini10, Proposition 3.2] gave a combinatorial characterization of
![]() $\operatorname{pd}S/I(G)$
. Later Kimura[Reference Kimura9, Theorem 7.1] translated his result in terms of
$\operatorname{pd}S/I(G)$
. Later Kimura[Reference Kimura9, Theorem 7.1] translated his result in terms of
![]() $G$
and Theorem 1.1 is a generalization of it. The characterization for the regularity of
$G$
and Theorem 1.1 is a generalization of it. The characterization for the regularity of
![]() $I(G)$
, denoted by
$I(G)$
, denoted by
![]() $\operatorname{reg}I(G)$
has already been done; Kummini [Reference Kummini10] studied when
$\operatorname{reg}I(G)$
has already been done; Kummini [Reference Kummini10] studied when
![]() $G$
is an unmixed bipartite graph and later Mahmoudi et al. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11] generalized Kummini’s result for a very well-covered graph
$G$
is an unmixed bipartite graph and later Mahmoudi et al. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11] generalized Kummini’s result for a very well-covered graph
![]() $G$
. Theorem 1.1 stands the Alexander dual version of their generalization since
$G$
. Theorem 1.1 stands the Alexander dual version of their generalization since
![]() $\operatorname{pd}S/I(G)=\operatorname{reg}J(G)$
; see [Reference Terai18].
$\operatorname{pd}S/I(G)=\operatorname{reg}J(G)$
; see [Reference Terai18].
In this article we also treat the projective dimension of symbolic powers of the edge ideal of a very well-covered graph. The following problem is widely open:
Problem 1.2. Let
![]() $G$
be a graph. Then is it true that
$G$
be a graph. Then is it true that
for
![]() $i\geqslant 2$
?
$i\geqslant 2$
?
We give a partial affirmative answer for the case that
![]() $G$
is a very well-covered graph. We also point out that the corresponding result also holds for Stanley depth.
$G$
is a very well-covered graph. We also point out that the corresponding result also holds for Stanley depth.
Now we explain the organization of the paper. In Section 2, we recall the structure of a very well-covered graph which was given by Crupi et al. [Reference Crupi, Rinaldo and Terai2] and the association a very well-covered graph with semidirected graph by Mahmoudi et al. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11]. In Section 3, we construct an explicit minimal free resolution of
![]() $J(G)$
for a Cohen–Macaulay very well-covered graph
$J(G)$
for a Cohen–Macaulay very well-covered graph
![]() $G$
and in Section 4, we prove Theorem 1.1. In Section 5, we show that the projective dimension of symbolic powers of the edge ideal of a very well-covered graph is nondecreasing.
$G$
and in Section 4, we prove Theorem 1.1. In Section 5, we show that the projective dimension of symbolic powers of the edge ideal of a very well-covered graph is nondecreasing.
2 The structure of very well-covered graphs
In this section, we recall the structure of very well-covered graphs. Almost all of the results in this section are proved by Crupi et al. [Reference Crupi, Rinaldo and Terai2] and Mahmoudi et al. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11].
Let
![]() $G$
be a finite simple graph with no isolated vertex. If
$G$
be a finite simple graph with no isolated vertex. If
![]() $G$
is unmixed, then
$G$
is unmixed, then
![]() $2\operatorname{height}I(G)\geqslant \#V(G)$
is known. A graph
$2\operatorname{height}I(G)\geqslant \#V(G)$
is known. A graph
![]() $G$
is called very well-covered if
$G$
is called very well-covered if
![]() $G$
is unmixed with
$G$
is unmixed with
![]() $2\operatorname{height}I(G)=\#V(G)$
.
$2\operatorname{height}I(G)=\#V(G)$
.
A subset
![]() $W\subset V(G)$
is called an independent set if there is no edge of
$W\subset V(G)$
is called an independent set if there is no edge of
![]() $G$
between any two vertices in
$G$
between any two vertices in
![]() $W$
. An independent set is said to be maximal if it is maximal among independent sets of
$W$
. An independent set is said to be maximal if it is maximal among independent sets of
![]() $G$
.
$G$
.
A very well-covered graph has the following structure.
Theorem 2.1. [Reference Crupi, Rinaldo and Terai2, Proposition 2.3]
Let
![]() $G$
be a very well-covered graph with height
$G$
be a very well-covered graph with height
![]() $h$
. Then there is a relabeling of vertices
$h$
. Then there is a relabeling of vertices
![]() $V(G)=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
such that the following conditions are satisfied:
$V(G)=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
such that the following conditions are satisfied:
(i)
 $X=\{x_{1},\ldots ,x_{h}\}$
is a minimal vertex cover of
$X=\{x_{1},\ldots ,x_{h}\}$
is a minimal vertex cover of
 $G$
and
$G$
and
 $Y=\{y_{1},\ldots ,y_{h}\}$
is a maximal independent set of
$Y=\{y_{1},\ldots ,y_{h}\}$
is a maximal independent set of
 $G$
;
$G$
;(ii)
 $\{x_{k},y_{k}\}\in E(G)$
for
$\{x_{k},y_{k}\}\in E(G)$
for
 $k=1,\ldots ,h$
;
$k=1,\ldots ,h$
;(iii) if
 $\{z_{i},x_{j}\},\{y_{j},x_{k}\}\in E(G)$
, then
$\{z_{i},x_{j}\},\{y_{j},x_{k}\}\in E(G)$
, then
 $\{z_{i},x_{k}\}\in E(G)$
for distinct
$\{z_{i},x_{k}\}\in E(G)$
for distinct
 $i,j,k$
and for
$i,j,k$
and for
 $z_{i}\in \{x_{i},y_{i}\}$
;
$z_{i}\in \{x_{i},y_{i}\}$
;(iv) if
 $\{x_{i},y_{j}\}\in E(G)$
, then
$\{x_{i},y_{j}\}\in E(G)$
, then
 $\{x_{i},x_{j}\}\notin E(G)$
.
$\{x_{i},x_{j}\}\notin E(G)$
.
On the other hand, the graph
![]() $G$
on
$G$
on
![]() $V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with (i), (ii), (iii), (iv) in Theorem 2.1 is a very well-covered graph.
$V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with (i), (ii), (iii), (iv) in Theorem 2.1 is a very well-covered graph.
Cohen–Macaulay very well-covered graphs have the following additional property.
Theorem 2.2. [Reference Crupi, Rinaldo and Terai2, Theorem 3.6]
Let
![]() $G$
be a very well-covered graph with height
$G$
be a very well-covered graph with height
![]() $h$
. Then
$h$
. Then
![]() $G$
is Cohen–Macaulay if and only if there is a relabeling of vertices
$G$
is Cohen–Macaulay if and only if there is a relabeling of vertices
![]() $V(G)=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with (i), (ii), (iii), (iv) of Theorem 2.1 and the following property:
$V(G)=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with (i), (ii), (iii), (iv) of Theorem 2.1 and the following property:
(v) if
 $\{x_{i},y_{j}\}\in E(G)$
, then
$\{x_{i},y_{j}\}\in E(G)$
, then
 $i\leqslant j$
.
$i\leqslant j$
.
Now we associate a very well-covered graph
![]() $G$
with a semidirected graph
$G$
with a semidirected graph
![]() $\mathfrak{d}_{G}$
as in [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11]. We recall the notion of a semidirected graph. A semidirected graph
$\mathfrak{d}_{G}$
as in [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11]. We recall the notion of a semidirected graph. A semidirected graph
![]() $\mathfrak{d}$
consists of the vertex set
$\mathfrak{d}$
consists of the vertex set
![]() $V(\mathfrak{d})=\{p_{1},\ldots ,p_{h}\}$
, the set of undirected edges
$V(\mathfrak{d})=\{p_{1},\ldots ,p_{h}\}$
, the set of undirected edges
![]() $E_{u}(\mathfrak{d})$
, and the set of directed edges
$E_{u}(\mathfrak{d})$
, and the set of directed edges
![]() $E_{d}(\mathfrak{d})$
, where if
$E_{d}(\mathfrak{d})$
, where if
![]() $p_{i}p_{j}\in E_{d}(\mathfrak{d})$
, then
$p_{i}p_{j}\in E_{d}(\mathfrak{d})$
, then
![]() $\{p_{i},p_{j}\}\notin E_{u}(\mathfrak{d})$
. We say
$\{p_{i},p_{j}\}\notin E_{u}(\mathfrak{d})$
. We say
![]() $A\subset V(\mathfrak{d})$
is independent if
$A\subset V(\mathfrak{d})$
is independent if
![]() $\{p_{i},p_{j}\}\notin E_{u}(\mathfrak{d})$
and
$\{p_{i},p_{j}\}\notin E_{u}(\mathfrak{d})$
and
![]() $p_{i}p_{j},p_{j}p_{i}\notin E_{d}(\mathfrak{d})$
for any
$p_{i}p_{j},p_{j}p_{i}\notin E_{d}(\mathfrak{d})$
for any
![]() $p_{i},p_{j}\in A$
,
$p_{i},p_{j}\in A$
,
![]() $i\neq j$
. Let
$i\neq j$
. Let
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}}$
denote the set of all independent sets in
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}}$
denote the set of all independent sets in
![]() $\mathfrak{d}$
. Then
$\mathfrak{d}$
. Then
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}}$
is a simplicial complex on
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}}$
is a simplicial complex on
![]() $V(\mathfrak{d})$
and is called the independence complex of
$V(\mathfrak{d})$
and is called the independence complex of
![]() $\mathfrak{d}$
.
$\mathfrak{d}$
.
A semidirected graph
![]() $\mathfrak{d}$
is called acyclic if
$\mathfrak{d}$
is called acyclic if
![]() $\mathfrak{d}$
has no directed cycle and is called transitively closed if the following two properties are satisfied for any distinct
$\mathfrak{d}$
has no directed cycle and is called transitively closed if the following two properties are satisfied for any distinct
![]() $i,j,k$
:
$i,j,k$
:
(TC1) if
 $p_{i}p_{j}\in E_{d}(\mathfrak{d})$
and
$p_{i}p_{j}\in E_{d}(\mathfrak{d})$
and
 $p_{j}p_{k}\in E_{d}(\mathfrak{d})$
, then
$p_{j}p_{k}\in E_{d}(\mathfrak{d})$
, then
 $p_{i}p_{k}\in E_{d}(\mathfrak{d})$
;
$p_{i}p_{k}\in E_{d}(\mathfrak{d})$
;(TC2) if
 $p_{i}p_{j}\in E_{d}(\mathfrak{d})$
and
$p_{i}p_{j}\in E_{d}(\mathfrak{d})$
and
 $\{p_{j},p_{k}\}\in E_{u}(\mathfrak{d})$
, then
$\{p_{j},p_{k}\}\in E_{u}(\mathfrak{d})$
, then
 $\{p_{i},p_{k}\}\in E_{u}(\mathfrak{d})$
.
$\{p_{i},p_{k}\}\in E_{u}(\mathfrak{d})$
.
Let
![]() $\mathfrak{d}$
be an acyclic and transitively closed semidirected graph. We define
$\mathfrak{d}$
be an acyclic and transitively closed semidirected graph. We define
![]() $p_{j}\succ p_{i}$
if
$p_{j}\succ p_{i}$
if
![]() $p_{i}p_{j}\in E_{d}(\mathfrak{d})$
, and
$p_{i}p_{j}\in E_{d}(\mathfrak{d})$
, and
![]() $p_{j}\succcurlyeq p_{i}$
if
$p_{j}\succcurlyeq p_{i}$
if
![]() $p_{j}=p_{i}$
or
$p_{j}=p_{i}$
or
![]() $p_{j}\succ p_{i}$
. Then
$p_{j}\succ p_{i}$
. Then
![]() $\prec$
is a partial order on
$\prec$
is a partial order on
![]() $V(\mathfrak{d})$
. For a subset
$V(\mathfrak{d})$
. For a subset
![]() $A\subset V(\mathfrak{d})$
, we define
$A\subset V(\mathfrak{d})$
, we define
![]() $p_{j}\succcurlyeq A$
if
$p_{j}\succcurlyeq A$
if
![]() $p_{j}\succcurlyeq p_{i}$
for some
$p_{j}\succcurlyeq p_{i}$
for some
![]() $p_{i}\in A$
.
$p_{i}\in A$
.
Let
![]() $\mathfrak{d}$
be a transitively closed semidirected graph. Two vertices
$\mathfrak{d}$
be a transitively closed semidirected graph. Two vertices
![]() $p_{i},p_{j}\in V(\mathfrak{d})$
are called strongly connected if
$p_{i},p_{j}\in V(\mathfrak{d})$
are called strongly connected if
![]() $p_{i}p_{j},p_{j}p_{i}\in E_{d}(\mathfrak{d})$
. Let
$p_{i}p_{j},p_{j}p_{i}\in E_{d}(\mathfrak{d})$
. Let
![]() ${\mathcal{Z}}_{1},\ldots ,{\mathcal{Z}}_{t}$
be the strongly connected components of
${\mathcal{Z}}_{1},\ldots ,{\mathcal{Z}}_{t}$
be the strongly connected components of
![]() $\mathfrak{d}$
. Note that
$\mathfrak{d}$
. Note that
![]() $V(\mathfrak{d})$
can be decomposed as
$V(\mathfrak{d})$
can be decomposed as
![]() ${\mathcal{Z}}_{1}\sqcup \cdots \sqcup {\mathcal{Z}}_{t}$
. Then we define a new semidirected graph
${\mathcal{Z}}_{1}\sqcup \cdots \sqcup {\mathcal{Z}}_{t}$
. Then we define a new semidirected graph
![]() $\widehat{\mathfrak{d}}$
, called the acyclic reduction of
$\widehat{\mathfrak{d}}$
, called the acyclic reduction of
![]() $\mathfrak{d}$
, as follows:
$\mathfrak{d}$
, as follows:
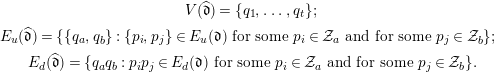 $$\begin{eqnarray}\displaystyle & \displaystyle V(\widehat{\mathfrak{d}})=\{q_{1},\ldots ,q_{t}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle \!\!E_{u}(\widehat{\mathfrak{d}})=\{\{q_{a},q_{b}\}:\{p_{i},p_{j}\}\in E_{u}(\mathfrak{d})\text{ for some }p_{i}\in {\mathcal{Z}}_{a}\text{ and for some }p_{j}\in {\mathcal{Z}}_{b}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{d}(\widehat{\mathfrak{d}})=\{q_{a}q_{b}:p_{i}p_{j}\in E_{d}(\mathfrak{d})\text{ for some }p_{i}\in {\mathcal{Z}}_{a}\text{ and for some }p_{j}\in {\mathcal{Z}}_{b}\}. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle V(\widehat{\mathfrak{d}})=\{q_{1},\ldots ,q_{t}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle \!\!E_{u}(\widehat{\mathfrak{d}})=\{\{q_{a},q_{b}\}:\{p_{i},p_{j}\}\in E_{u}(\mathfrak{d})\text{ for some }p_{i}\in {\mathcal{Z}}_{a}\text{ and for some }p_{j}\in {\mathcal{Z}}_{b}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{d}(\widehat{\mathfrak{d}})=\{q_{a}q_{b}:p_{i}p_{j}\in E_{d}(\mathfrak{d})\text{ for some }p_{i}\in {\mathcal{Z}}_{a}\text{ and for some }p_{j}\in {\mathcal{Z}}_{b}\}. & \displaystyle \nonumber\end{eqnarray}$$
Note that
![]() $\widehat{\mathfrak{d}}$
is acyclic. Also
$\widehat{\mathfrak{d}}$
is acyclic. Also
![]() $\widehat{\mathfrak{d}}$
is transitively closed since
$\widehat{\mathfrak{d}}$
is transitively closed since
![]() $\mathfrak{d}$
is transitively closed.
$\mathfrak{d}$
is transitively closed.
Let
![]() $G$
be a very well-covered graph with (i), (ii), (iii), (iv) of Theorem 2.1. Then we define the semidirected graph
$G$
be a very well-covered graph with (i), (ii), (iii), (iv) of Theorem 2.1. Then we define the semidirected graph
![]() $\mathfrak{d}_{G}$
as follows:
$\mathfrak{d}_{G}$
as follows:
 $$\begin{eqnarray}\displaystyle & \displaystyle V(\mathfrak{d}_{G})=\{p_{1},\ldots ,p_{h}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{u}(\mathfrak{d}_{G})=\{\{p_{i},p_{j}\}:\{x_{i},x_{j}\}\in E(G)\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{d}(\mathfrak{d}_{G})=\{p_{i}p_{j}:\{x_{i},y_{j}\}\in E(G)\}. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle V(\mathfrak{d}_{G})=\{p_{1},\ldots ,p_{h}\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{u}(\mathfrak{d}_{G})=\{\{p_{i},p_{j}\}:\{x_{i},x_{j}\}\in E(G)\}; & \displaystyle \nonumber\\ \displaystyle & \displaystyle E_{d}(\mathfrak{d}_{G})=\{p_{i}p_{j}:\{x_{i},y_{j}\}\in E(G)\}. & \displaystyle \nonumber\end{eqnarray}$$
Note that
![]() $\mathfrak{d}_{G}$
is transitively closed. Now let us consider the acyclic reduction
$\mathfrak{d}_{G}$
is transitively closed. Now let us consider the acyclic reduction
![]() $\widehat{\mathfrak{d}_{G}}$
. We define a new graph
$\widehat{\mathfrak{d}_{G}}$
. We define a new graph
![]() $\widehat{G}$
, called the acyclic reduction of
$\widehat{G}$
, called the acyclic reduction of
![]() $G$
as follows:
$G$
as follows:
 $$\begin{eqnarray}\displaystyle V(\widehat{G}) & = & \displaystyle \{u_{1},\ldots ,u_{t}\}\cup \{v_{1},\ldots ,v_{t}\};\nonumber\\ \displaystyle E(\widehat{G}) & = & \displaystyle \{\{u_{a},v_{a}\}:a=1,\ldots ,t\}\nonumber\\ \displaystyle & \cup & \displaystyle \{\{u_{a},u_{b}\}:\{q_{a},q_{b}\}\in E_{u}(\widehat{\mathfrak{d}_{G}})\}\cup \{\{u_{a},v_{b}\}:q_{a}q_{b}\in E_{d}(\widehat{\mathfrak{d}_{G}})\}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle V(\widehat{G}) & = & \displaystyle \{u_{1},\ldots ,u_{t}\}\cup \{v_{1},\ldots ,v_{t}\};\nonumber\\ \displaystyle E(\widehat{G}) & = & \displaystyle \{\{u_{a},v_{a}\}:a=1,\ldots ,t\}\nonumber\\ \displaystyle & \cup & \displaystyle \{\{u_{a},u_{b}\}:\{q_{a},q_{b}\}\in E_{u}(\widehat{\mathfrak{d}_{G}})\}\cup \{\{u_{a},v_{b}\}:q_{a}q_{b}\in E_{d}(\widehat{\mathfrak{d}_{G}})\}.\nonumber\end{eqnarray}$$
Then
![]() $\widehat{G}$
is a Cohen–Macaulay very well-covered graph [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Lemma 4.5]. Note that
$\widehat{G}$
is a Cohen–Macaulay very well-covered graph [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Lemma 4.5]. Note that
![]() $\mathfrak{d}_{\widehat{G}}=\widehat{\mathfrak{d}_{G}}$
and we denote it by
$\mathfrak{d}_{\widehat{G}}=\widehat{\mathfrak{d}_{G}}$
and we denote it by
![]() $\widehat{\mathfrak{d}}_{G}$
.
$\widehat{\mathfrak{d}}_{G}$
.
For
![]() $\emptyset \neq \widehat{A}\in \unicode[STIX]{x1D6E5}_{\widehat{\mathfrak{d}}_{G}}$
, we set
$\emptyset \neq \widehat{A}\in \unicode[STIX]{x1D6E5}_{\widehat{\mathfrak{d}}_{G}}$
, we set
![]() $\unicode[STIX]{x1D6FA}_{\widehat{A}}:=\bigcup _{q_{b}\succcurlyeq A}{\mathcal{Z}}_{b}$
and
$\unicode[STIX]{x1D6FA}_{\widehat{A}}:=\bigcup _{q_{b}\succcurlyeq A}{\mathcal{Z}}_{b}$
and
![]() $\unicode[STIX]{x1D6FA}_{\emptyset }:=\emptyset$
.
$\unicode[STIX]{x1D6FA}_{\emptyset }:=\emptyset$
.
Lemma 2.3. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Lemma 4.10]
Let
![]() $G$
be a very well-covered graph satisfying (i), (ii), (iii), (iv) of Theorem 2.1. Then
$G$
be a very well-covered graph satisfying (i), (ii), (iii), (iv) of Theorem 2.1. Then
Finally, we note the following lemma, which will be a key to the construction of a minimal free resolution of
![]() $J(G)$
when
$J(G)$
when
![]() $G$
is a Cohen–Macaulay very well-covered graph; see the next section.
$G$
is a Cohen–Macaulay very well-covered graph; see the next section.
Lemma 2.4. [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Lemma 4.9]
Let
![]() $G$
be a very well-covered graph satisfying (i), (ii), (iii), (iv) of Theorem 2.1. Then
$G$
be a very well-covered graph satisfying (i), (ii), (iii), (iv) of Theorem 2.1. Then
![]() $\unicode[STIX]{x1D6FA}_{\widehat{A}}$
does not contain any undirected edge in
$\unicode[STIX]{x1D6FA}_{\widehat{A}}$
does not contain any undirected edge in
![]() $\mathfrak{d}_{G}$
for any
$\mathfrak{d}_{G}$
for any
![]() $\widehat{A}\in \unicode[STIX]{x1D6E5}_{\widehat{\mathfrak{d}}_{G}}$
.
$\widehat{A}\in \unicode[STIX]{x1D6E5}_{\widehat{\mathfrak{d}}_{G}}$
.
3 A minimal free resolution of
 $J(G)$
where
$J(G)$
where
 $G$
is Cohen–Macaulay
$G$
is Cohen–Macaulay
The proof of Theorem 1.1 is inspired of that for the case of Cohen–Macaulay bipartite graphs in [Reference Kimura9]. This proof is based on an explicit minimal free resolution of the cover ideal
![]() $J(G)$
given by Herzog and Hibi [Reference Herzog and Hibi6]. In this section, we construct an explicit minimal free resolution of
$J(G)$
given by Herzog and Hibi [Reference Herzog and Hibi6]. In this section, we construct an explicit minimal free resolution of
![]() $J(G)$
for a Cohen–Macaulay very well-covered graph
$J(G)$
for a Cohen–Macaulay very well-covered graph
![]() $G$
. This is done by a similar construction to the one by Herzog and Hibi [Reference Herzog and Hibi6].
$G$
. This is done by a similar construction to the one by Herzog and Hibi [Reference Herzog and Hibi6].
Let
![]() $G$
be a Cohen–Macaulay very well-covered graph on
$G$
be a Cohen–Macaulay very well-covered graph on
![]() $V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) in Theorem 2.1 and (v) in Theorem 2.2. For
$V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) in Theorem 2.1 and (v) in Theorem 2.2. For
![]() $A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
, we denote
$A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
, we denote
By Lemma 2.3, we know
Remark 3.1. Our notation of
![]() $x_{i},y_{j}$
are converse to the one in Herzog and Hibi [Reference Herzog and Hibi6]. Also “minimal” in our construction corresponds to “maximal” in that of [Reference Herzog and Hibi6].
$x_{i},y_{j}$
are converse to the one in Herzog and Hibi [Reference Herzog and Hibi6]. Also “minimal” in our construction corresponds to “maximal” in that of [Reference Herzog and Hibi6].
We set
![]() ${\mathcal{L}}=\{\unicode[STIX]{x1D6FA}_{A}:A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}\}$
. For
${\mathcal{L}}=\{\unicode[STIX]{x1D6FA}_{A}:A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}\}$
. For
![]() $\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
, let
$\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
, let
![]() $A(\unicode[STIX]{x1D6FA})$
denote the minimal elements in
$A(\unicode[STIX]{x1D6FA})$
denote the minimal elements in
![]() $\unicode[STIX]{x1D6FA}$
with respect to
$\unicode[STIX]{x1D6FA}$
with respect to
![]() $\prec$
. Note that by Lemma 2.4, no two vertices in
$\prec$
. Note that by Lemma 2.4, no two vertices in
![]() $\unicode[STIX]{x1D6FA}$
form an undirected edge in
$\unicode[STIX]{x1D6FA}$
form an undirected edge in
![]() $\mathfrak{d}_{G}$
. It then follows that
$\mathfrak{d}_{G}$
. It then follows that
![]() $A(\unicode[STIX]{x1D6FA})$
is independent in
$A(\unicode[STIX]{x1D6FA})$
is independent in
![]() $\mathfrak{d}_{G}$
and the set
$\mathfrak{d}_{G}$
and the set
![]() $A(\unicode[STIX]{x1D6FA})$
is the unique face
$A(\unicode[STIX]{x1D6FA})$
is the unique face
![]() $A$
in
$A$
in
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
with
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
with
![]() $\unicode[STIX]{x1D6FA}=\unicode[STIX]{x1D6FA}_{A}$
. Hence there is a one-to-one correspondence between
$\unicode[STIX]{x1D6FA}=\unicode[STIX]{x1D6FA}_{A}$
. Hence there is a one-to-one correspondence between
![]() $\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
and
$\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
and
![]() $A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
.
$A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
.
Now we construct a (
![]() $\mathbb{N}^{2h}$
-graded) minimal free resolution
$\mathbb{N}^{2h}$
-graded) minimal free resolution
![]() ${\mathcal{F}}_{\bullet }$
of
${\mathcal{F}}_{\bullet }$
of
![]() $J(G)$
as follows. For all
$J(G)$
as follows. For all
![]() $i\geqslant 0$
, let
$i\geqslant 0$
, let
![]() ${\mathcal{F}}_{i}$
denote the free
${\mathcal{F}}_{i}$
denote the free
![]() $S$
-module with basis
$S$
-module with basis
![]() $e(\unicode[STIX]{x1D6FA},T)$
, where
$e(\unicode[STIX]{x1D6FA},T)$
, where
![]() $\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
and
$\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
and
![]() $T\subset V(\mathfrak{d}_{G})$
satisfying
$T\subset V(\mathfrak{d}_{G})$
satisfying
The degree of
![]() $e(\unicode[STIX]{x1D6FA},T)$
is defined by
$e(\unicode[STIX]{x1D6FA},T)$
is defined by
![]() $\deg u_{A(\unicode[STIX]{x1D6FA}),T}$
where we define
$\deg u_{A(\unicode[STIX]{x1D6FA}),T}$
where we define
for
![]() $A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. Also for all
$A\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. Also for all
![]() $i\geqslant 1$
, we define the differential
$i\geqslant 1$
, we define the differential
![]() $\unicode[STIX]{x2202}_{i}:{\mathcal{F}}_{i}\longrightarrow {\mathcal{F}}_{i-1}$
by
$\unicode[STIX]{x2202}_{i}:{\mathcal{F}}_{i}\longrightarrow {\mathcal{F}}_{i-1}$
by
where for
![]() $Q\subset V(\mathfrak{d}_{G})$
and
$Q\subset V(\mathfrak{d}_{G})$
and
![]() $p_{\ell }\in Q$
, we set
$p_{\ell }\in Q$
, we set
![]() $\unicode[STIX]{x1D70E}(Q,p_{\ell }):=\#\{p_{k}\in Q:k<\ell \}$
. Since
$\unicode[STIX]{x1D70E}(Q,p_{\ell }):=\#\{p_{k}\in Q:k<\ell \}$
. Since
![]() $\unicode[STIX]{x1D6FA}$
contains no undirected edge in
$\unicode[STIX]{x1D6FA}$
contains no undirected edge in
![]() $\mathfrak{d}_{G}$
, we have that
$\mathfrak{d}_{G}$
, we have that
![]() $\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\}=\unicode[STIX]{x1D6FA}_{A^{\prime }}\in {\mathcal{L}}$
where
$\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\}=\unicode[STIX]{x1D6FA}_{A^{\prime }}\in {\mathcal{L}}$
where
![]() $A^{\prime }$
is the set of minimal elements of
$A^{\prime }$
is the set of minimal elements of
![]() $\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\}$
with respect to
$\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\}$
with respect to
![]() $\prec$
and it is an independent set in
$\prec$
and it is an independent set in
![]() $\mathfrak{d}_{G}$
. Then it is easy to see that
$\mathfrak{d}_{G}$
. Then it is easy to see that
![]() $e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T),e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell }\})$
are free bases of
$e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T),e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell }\})$
are free bases of
![]() ${\mathcal{F}}_{i-1}$
and
${\mathcal{F}}_{i-1}$
and
![]() $\unicode[STIX]{x2202}_{i}$
possesses the multidegree.
$\unicode[STIX]{x2202}_{i}$
possesses the multidegree.
The following is the main result of this section.
Theorem 3.2.
![]() $({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is a
$({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is a
![]() $\mathbb{N}^{2h}$
-graded minimal free resolution of
$\mathbb{N}^{2h}$
-graded minimal free resolution of
![]() $J(G)$
.
$J(G)$
.
Before proving the theorem, we recall some notion on graphs. Let
![]() $G$
be a simple graph on the vertex set
$G$
be a simple graph on the vertex set
![]() $V$
. Take
$V$
. Take
![]() $W\subset V$
. The induced subgraph of
$W\subset V$
. The induced subgraph of
![]() $G$
on
$G$
on
![]() $W$
is the graph whose vertex set is
$W$
is the graph whose vertex set is
![]() $W$
and whose edge set is the set of all edges of
$W$
and whose edge set is the set of all edges of
![]() $G$
whose two end vertices are in
$G$
whose two end vertices are in
![]() $W$
. Also,
$W$
. Also,
![]() $G\setminus W$
denotes the induced subgraph
$G\setminus W$
denotes the induced subgraph
![]() $G_{V\setminus W}$
. For
$G_{V\setminus W}$
. For
![]() $x\in V$
, we denote by
$x\in V$
, we denote by
![]() $N_{G}(x)$
the set of neighbors of
$N_{G}(x)$
the set of neighbors of
![]() $x$
in
$x$
in
![]() $G$
:
$G$
:
![]() $N_{G}(x):=\{y\in V:\{x,y\}\in E(G)\}$
.
$N_{G}(x):=\{y\in V:\{x,y\}\in E(G)\}$
.
Proof of Theorem 3.2.
We use arguments similar to those of Herzog and Hibi [Reference Herzog and Hibi6, Theorem 2.1].
Set
![]() $V^{\prime }:=V(\mathfrak{d}_{G})$
. The free basis
$V^{\prime }:=V(\mathfrak{d}_{G})$
. The free basis
![]() $e(\unicode[STIX]{x1D6FA},T)$
of
$e(\unicode[STIX]{x1D6FA},T)$
of
![]() ${\mathcal{F}}_{0}$
satisfies
${\mathcal{F}}_{0}$
satisfies
![]() $\unicode[STIX]{x1D6FA}\cap T=\emptyset$
and
$\unicode[STIX]{x1D6FA}\cap T=\emptyset$
and
![]() $\unicode[STIX]{x1D6FA}\cup T=V^{\prime }$
. Thus
$\unicode[STIX]{x1D6FA}\cup T=V^{\prime }$
. Thus
![]() $T$
is uniquely determined by
$T$
is uniquely determined by
![]() $\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
:
$\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
:
![]() $T=V^{\prime }\setminus \unicode[STIX]{x1D6FA}$
. Also
$T=V^{\prime }\setminus \unicode[STIX]{x1D6FA}$
. Also
![]() $\deg e(\unicode[STIX]{x1D6FA},T)=\deg u_{\unicode[STIX]{x1D6FA},T}=\deg u_{A(\unicode[STIX]{x1D6FA})}$
. Define the augmentation
$\deg e(\unicode[STIX]{x1D6FA},T)=\deg u_{\unicode[STIX]{x1D6FA},T}=\deg u_{A(\unicode[STIX]{x1D6FA})}$
. Define the augmentation
![]() $\unicode[STIX]{x1D700}:{\mathcal{F}}_{0}\longrightarrow J(G)$
by
$\unicode[STIX]{x1D700}:{\mathcal{F}}_{0}\longrightarrow J(G)$
by
![]() $e(\unicode[STIX]{x1D6FA},T)\mapsto u_{A(\unicode[STIX]{x1D6FA})}$
.
$e(\unicode[STIX]{x1D6FA},T)\mapsto u_{A(\unicode[STIX]{x1D6FA})}$
.
Claim 1.
![]() ${\mathcal{F}}_{1}\stackrel{\unicode[STIX]{x2202}_{1}}{\longrightarrow }{\mathcal{F}}_{0}\stackrel{\unicode[STIX]{x1D700}}{\longrightarrow }J(G)\longrightarrow 0$
is a complex.
${\mathcal{F}}_{1}\stackrel{\unicode[STIX]{x2202}_{1}}{\longrightarrow }{\mathcal{F}}_{0}\stackrel{\unicode[STIX]{x1D700}}{\longrightarrow }J(G)\longrightarrow 0$
is a complex.
Take a free basis
![]() $e(\unicode[STIX]{x1D6FA},T)$
of
$e(\unicode[STIX]{x1D6FA},T)$
of
![]() ${\mathcal{F}}_{1}$
. Note that
${\mathcal{F}}_{1}$
. Note that
![]() $\#(\unicode[STIX]{x1D6FA}\cap T)=1$
and set
$\#(\unicode[STIX]{x1D6FA}\cap T)=1$
and set
![]() $\unicode[STIX]{x1D6FA}\cap T=\{p_{\ell }\}$
. Then
$\unicode[STIX]{x1D6FA}\cap T=\{p_{\ell }\}$
. Then
as desired.
Claim 2.
![]() $\unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}=0$
.
$\unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}=0$
.
Note that
 $$\begin{eqnarray}\displaystyle & & \displaystyle \unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}(e(\unicode[STIX]{x1D6FA},T))\nonumber\\ \displaystyle & & \displaystyle \quad =\unicode[STIX]{x2202}_{i}\left(\mathop{\sum }_{p_{\ell }\in \unicode[STIX]{x1D6FA}\cap T}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap T,p_{\ell })}(y_{\ell }e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T)-x_{\ell }e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell }\}))\right)\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{p_{\ell }\in \unicode[STIX]{x1D6FA}\cap T}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap T,p_{\ell })}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \left[y_{\ell }\mathop{\sum }_{\substack{ p_{k}\in \unicode[STIX]{x1D6FA}\cap T \\ k\neq \ell }}(-1)^{\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})}\right.\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad \times \,(y_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell },p_{k}\},T)-x_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T\setminus \{p_{k}\}))\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad -\,x_{\ell }\mathop{\sum }_{\substack{ p_{k}\in \unicode[STIX]{x1D6FA}\cap T \\ k\neq \ell }}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap (T\setminus \{p_{\ell }\}),p_{k})}\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad \times \left.(y_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{k}\},T\setminus \{p_{\ell }\})-x_{k}e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell },p_{k}\}))\right].\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}(e(\unicode[STIX]{x1D6FA},T))\nonumber\\ \displaystyle & & \displaystyle \quad =\unicode[STIX]{x2202}_{i}\left(\mathop{\sum }_{p_{\ell }\in \unicode[STIX]{x1D6FA}\cap T}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap T,p_{\ell })}(y_{\ell }e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T)-x_{\ell }e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell }\}))\right)\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{p_{\ell }\in \unicode[STIX]{x1D6FA}\cap T}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap T,p_{\ell })}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \left[y_{\ell }\mathop{\sum }_{\substack{ p_{k}\in \unicode[STIX]{x1D6FA}\cap T \\ k\neq \ell }}(-1)^{\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})}\right.\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad \times \,(y_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell },p_{k}\},T)-x_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T\setminus \{p_{k}\}))\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad -\,x_{\ell }\mathop{\sum }_{\substack{ p_{k}\in \unicode[STIX]{x1D6FA}\cap T \\ k\neq \ell }}(-1)^{\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap (T\setminus \{p_{\ell }\}),p_{k})}\nonumber\\ \displaystyle & & \displaystyle \qquad \qquad \times \left.(y_{k}e(\unicode[STIX]{x1D6FA}\setminus \{p_{k}\},T\setminus \{p_{\ell }\})-x_{k}e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell },p_{k}\}))\right].\nonumber\end{eqnarray}$$
Then the coefficient of
![]() $e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell },p_{k}\},T)$
,
$e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell },p_{k}\},T)$
,
![]() $k\neq \ell$
vanishes since the differences of
$k\neq \ell$
vanishes since the differences of
![]() $\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})$
and
$\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})$
and
![]() $\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{k}\})\cap T,p_{\ell })$
is just
$\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{k}\})\cap T,p_{\ell })$
is just
![]() $1$
. Similarly, the coefficient of
$1$
. Similarly, the coefficient of
![]() $e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell },p_{k}\})$
,
$e(\unicode[STIX]{x1D6FA},T\setminus \{p_{\ell },p_{k}\})$
,
![]() $k\neq \ell$
vanishes. Finally we check the coefficient of
$k\neq \ell$
vanishes. Finally we check the coefficient of
![]() $e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T\setminus \{p_{k}\})$
. In this case,
$e(\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\},T\setminus \{p_{k}\})$
. In this case,
![]() $\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})$
and
$\unicode[STIX]{x1D70E}((\unicode[STIX]{x1D6FA}\setminus \{p_{\ell }\})\cap T,p_{k})$
and
![]() $\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap (T\setminus \{p_{k}\}),p_{\ell })$
also differ just by
$\unicode[STIX]{x1D70E}(\unicode[STIX]{x1D6FA}\cap (T\setminus \{p_{k}\}),p_{\ell })$
also differ just by
![]() $1$
. Therefore, we have
$1$
. Therefore, we have
![]() $\unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}(e(\unicode[STIX]{x1D6FA},T))=0$
.
$\unicode[STIX]{x2202}_{i}\circ \unicode[STIX]{x2202}_{i-1}(e(\unicode[STIX]{x1D6FA},T))=0$
.
Claim 3.
![]() ${\mathcal{F}}_{1}\stackrel{\unicode[STIX]{x2202}_{1}}{\longrightarrow }{\mathcal{F}}_{0}\stackrel{\unicode[STIX]{x1D700}}{\longrightarrow }J(G)\longrightarrow 0$
is exact.
${\mathcal{F}}_{1}\stackrel{\unicode[STIX]{x2202}_{1}}{\longrightarrow }{\mathcal{F}}_{0}\stackrel{\unicode[STIX]{x1D700}}{\longrightarrow }J(G)\longrightarrow 0$
is exact.
We first note that the first syzygy module of
![]() $J(G)$
is generated by
$J(G)$
is generated by
where for
![]() $\unicode[STIX]{x1D6FA}\subset V^{\prime }$
, we denote
$\unicode[STIX]{x1D6FA}\subset V^{\prime }$
, we denote
For
![]() $\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
, the intersection
$\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
, the intersection
![]() $\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}$
is in
$\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}$
is in
![]() ${\mathcal{L}}$
because
${\mathcal{L}}$
because
![]() $\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}_{A^{\prime }}$
where
$\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}_{A^{\prime }}$
where
![]() $A^{\prime }$
is the set of minimal elements of
$A^{\prime }$
is the set of minimal elements of
![]() $\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}$
with respect to
$\unicode[STIX]{x1D6FA}\cap \unicode[STIX]{x1D6E9}$
with respect to
![]() $\prec$
. Also we can easily check that
$\prec$
. Also we can easily check that
Therefore, in order to prove the claim, it is sufficient to prove that
![]() $r_{\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}}\in \unicode[STIX]{x2202}_{1}({\mathcal{F}}_{1})$
for
$r_{\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}}\in \unicode[STIX]{x2202}_{1}({\mathcal{F}}_{1})$
for
![]() $\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
with
$\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
with
![]() $\unicode[STIX]{x1D6E9}\subset \unicode[STIX]{x1D6FA}$
; let
$\unicode[STIX]{x1D6E9}\subset \unicode[STIX]{x1D6FA}$
; let
![]() $\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
be such a pair. Set
$\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}\in {\mathcal{L}}$
be such a pair. Set
![]() $\unicode[STIX]{x1D6FA}\setminus \unicode[STIX]{x1D6E9}=\{p_{k_{1}},\ldots ,p_{k_{m}}\}$
, where
$\unicode[STIX]{x1D6FA}\setminus \unicode[STIX]{x1D6E9}=\{p_{k_{1}},\ldots ,p_{k_{m}}\}$
, where
![]() $k_{1}>\cdots >k_{m}$
, and
$k_{1}>\cdots >k_{m}$
, and
We show that
![]() $\unicode[STIX]{x1D6FA}_{j}\in {\mathcal{L}}$
for each
$\unicode[STIX]{x1D6FA}_{j}\in {\mathcal{L}}$
for each
![]() $j$
.
$j$
.
Let
![]() $A_{j}$
denote the set of minimal elements of
$A_{j}$
denote the set of minimal elements of
![]() $\unicode[STIX]{x1D6FA}_{j}$
. We prove
$\unicode[STIX]{x1D6FA}_{j}$
. We prove
![]() $\unicode[STIX]{x1D6FA}_{j}=\unicode[STIX]{x1D6FA}_{A_{j}}$
. It is enough to show that
$\unicode[STIX]{x1D6FA}_{j}=\unicode[STIX]{x1D6FA}_{A_{j}}$
. It is enough to show that
![]() $\unicode[STIX]{x1D6FA}_{j}\supset \unicode[STIX]{x1D6FA}_{A_{j}}$
. Take
$\unicode[STIX]{x1D6FA}_{j}\supset \unicode[STIX]{x1D6FA}_{A_{j}}$
. Take
![]() $p_{\ell }\in \unicode[STIX]{x1D6FA}_{A_{j}}$
. Then there exists
$p_{\ell }\in \unicode[STIX]{x1D6FA}_{A_{j}}$
. Then there exists
![]() $p_{k}\in A_{j}$
with
$p_{k}\in A_{j}$
with
![]() $p_{k}\preccurlyeq p_{\ell }$
. We note that
$p_{k}\preccurlyeq p_{\ell }$
. We note that
![]() $k\leqslant \ell$
by the property (v) of Theorem 2.2. We also note that
$k\leqslant \ell$
by the property (v) of Theorem 2.2. We also note that
![]() $p_{k}\in A_{j}\subset \unicode[STIX]{x1D6FA}_{j}\subset \unicode[STIX]{x1D6FA}$
, and combining this with
$p_{k}\in A_{j}\subset \unicode[STIX]{x1D6FA}_{j}\subset \unicode[STIX]{x1D6FA}$
, and combining this with
![]() $\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
, we have
$\unicode[STIX]{x1D6FA}\in {\mathcal{L}}$
, we have
![]() $p_{\ell }\in \unicode[STIX]{x1D6FA}$
. By the same reason, when
$p_{\ell }\in \unicode[STIX]{x1D6FA}$
. By the same reason, when
![]() $p_{k}\in \unicode[STIX]{x1D6E9}$
, we have
$p_{k}\in \unicode[STIX]{x1D6E9}$
, we have
![]() $p_{\ell }\in \unicode[STIX]{x1D6E9}$
. Since
$p_{\ell }\in \unicode[STIX]{x1D6E9}$
. Since
![]() $\unicode[STIX]{x1D6E9}\subset \unicode[STIX]{x1D6FA}_{j}$
, we have done for the case
$\unicode[STIX]{x1D6E9}\subset \unicode[STIX]{x1D6FA}_{j}$
, we have done for the case
![]() $p_{k}\in \unicode[STIX]{x1D6E9}$
. Thus we assume
$p_{k}\in \unicode[STIX]{x1D6E9}$
. Thus we assume
![]() $p_{k}\notin \unicode[STIX]{x1D6E9}$
. If
$p_{k}\notin \unicode[STIX]{x1D6E9}$
. If
![]() $p_{\ell }\in \unicode[STIX]{x1D6E9}$
, then
$p_{\ell }\in \unicode[STIX]{x1D6E9}$
, then
![]() $p_{\ell }\in \unicode[STIX]{x1D6FA}_{j}$
is obvious. If
$p_{\ell }\in \unicode[STIX]{x1D6FA}_{j}$
is obvious. If
![]() $p_{\ell }\notin \unicode[STIX]{x1D6E9}$
, it follows that
$p_{\ell }\notin \unicode[STIX]{x1D6E9}$
, it follows that
![]() $p_{k},p_{\ell }\in \unicode[STIX]{x1D6FA}\setminus \unicode[STIX]{x1D6E9}$
. Since
$p_{k},p_{\ell }\in \unicode[STIX]{x1D6FA}\setminus \unicode[STIX]{x1D6E9}$
. Since
![]() $\ell \geqslant k$
and
$\ell \geqslant k$
and
![]() $p_{k}\in \unicode[STIX]{x1D6FA}_{j}$
, we conclude that
$p_{k}\in \unicode[STIX]{x1D6FA}_{j}$
, we conclude that
![]() $p_{\ell }\in \unicode[STIX]{x1D6FA}_{j}$
by the construction of
$p_{\ell }\in \unicode[STIX]{x1D6FA}_{j}$
by the construction of
![]() $\unicode[STIX]{x1D6FA}_{j}$
.
$\unicode[STIX]{x1D6FA}_{j}$
.
Note that
![]() $p_{k_{j}}$
must be a minimal element of
$p_{k_{j}}$
must be a minimal element of
![]() $\unicode[STIX]{x1D6FA}_{j}$
and
$\unicode[STIX]{x1D6FA}_{j}$
and
By the easy calculation, we have
 $$\begin{eqnarray}\displaystyle & \displaystyle r_{\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}}=\mathop{\sum }_{j=1}^{m}\left(\mathop{\prod }_{s=j+1}^{m}y_{k_{s}}\mathop{\prod }_{s=1}^{j-1}x_{k_{s}}\right)r_{\unicode[STIX]{x1D6FA}_{j},\unicode[STIX]{x1D6FA}_{j-1}}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle r_{\unicode[STIX]{x1D6FA}_{j},\unicode[STIX]{x1D6FA}_{j-1}}=-\unicode[STIX]{x2202}(e(\unicode[STIX]{x1D6FA}_{j},V^{\prime }\setminus \unicode[STIX]{x1D6FA}_{j-1})),\quad j=1,\ldots ,m. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle r_{\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6E9}}=\mathop{\sum }_{j=1}^{m}\left(\mathop{\prod }_{s=j+1}^{m}y_{k_{s}}\mathop{\prod }_{s=1}^{j-1}x_{k_{s}}\right)r_{\unicode[STIX]{x1D6FA}_{j},\unicode[STIX]{x1D6FA}_{j-1}}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle r_{\unicode[STIX]{x1D6FA}_{j},\unicode[STIX]{x1D6FA}_{j-1}}=-\unicode[STIX]{x2202}(e(\unicode[STIX]{x1D6FA}_{j},V^{\prime }\setminus \unicode[STIX]{x1D6FA}_{j-1})),\quad j=1,\ldots ,m. & \displaystyle \nonumber\end{eqnarray}$$
Therefore, the claim follows.
Claim 4.
![]() $({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is acyclic.
$({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is acyclic.
We proceed by induction on
![]() $h$
.
$h$
.
If
![]() $h=1$
, the cover ideal
$h=1$
, the cover ideal
![]() $J(G)=(x_{1},y_{1})$
. Then
$J(G)=(x_{1},y_{1})$
. Then
![]() $({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is just the Koszul complex associated with
$({\mathcal{F}}_{\bullet },\unicode[STIX]{x2202}_{\bullet })$
is just the Koszul complex associated with
![]() $\{x_{1},y_{1}\}$
and thus it is acyclic.
$\{x_{1},y_{1}\}$
and thus it is acyclic.
Now we assume
![]() $h>1$
. The induced subgraph
$h>1$
. The induced subgraph
![]() $G^{\prime }:=G_{V\setminus \{x_{1},y_{1}\}}$
is also a Cohen–Macaulay very well-covered graph. Note that
$G^{\prime }:=G_{V\setminus \{x_{1},y_{1}\}}$
is also a Cohen–Macaulay very well-covered graph. Note that
![]() $G^{\prime }$
satisfies the properties (i), (ii), (iii), (iv) of Theorem 2.1 and (v) of Theorem 2.2 with respect to the induced labeling from
$G^{\prime }$
satisfies the properties (i), (ii), (iii), (iv) of Theorem 2.1 and (v) of Theorem 2.2 with respect to the induced labeling from
![]() $G$
. Let
$G$
. Let
![]() $({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
denote the complex corresponding to
$({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
denote the complex corresponding to
![]() $G^{\prime }$
. Then by inductive hypothesis,
$G^{\prime }$
. Then by inductive hypothesis,
![]() $({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
is acyclic. Since the inclusion
$({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
is acyclic. Since the inclusion
![]() $K[V(G^{\prime })]\longrightarrow K[V(G)]$
is a flat extension, by tensoring
$K[V(G^{\prime })]\longrightarrow K[V(G)]$
is a flat extension, by tensoring
![]() $K[V(G)]$
to
$K[V(G)]$
to
![]() $({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
, we obtain the acyclic complex over
$({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
, we obtain the acyclic complex over
![]() $K[V(G)]$
. We define the map
$K[V(G)]$
. We define the map
![]() $\unicode[STIX]{x1D719}:{\mathcal{F}}^{\prime }\longrightarrow {\mathcal{F}}$
by
$\unicode[STIX]{x1D719}:{\mathcal{F}}^{\prime }\longrightarrow {\mathcal{F}}$
by
![]() $e(\unicode[STIX]{x1D6FA},T)\mapsto e(\unicode[STIX]{x1D6FA},T\cup \{p_{1}\})$
. Then
$e(\unicode[STIX]{x1D6FA},T)\mapsto e(\unicode[STIX]{x1D6FA},T\cup \{p_{1}\})$
. Then
![]() $\unicode[STIX]{x1D719}$
is an injective map of complexes. Also the induced map
$\unicode[STIX]{x1D719}$
is an injective map of complexes. Also the induced map
![]() $J(G^{\prime })=H_{0}({\mathcal{F}}^{\prime })\longrightarrow H_{0}({\mathcal{F}})=J(G)$
is a multiplication by
$J(G^{\prime })=H_{0}({\mathcal{F}}^{\prime })\longrightarrow H_{0}({\mathcal{F}})=J(G)$
is a multiplication by
![]() $x_{1}$
. Let
$x_{1}$
. Let
![]() ${\mathcal{G}}_{\bullet }$
be the quotient complex
${\mathcal{G}}_{\bullet }$
be the quotient complex
![]() ${\mathcal{F}}/{\mathcal{F}}^{\prime }$
. Then the short exact sequence of complexes
${\mathcal{F}}/{\mathcal{F}}^{\prime }$
. Then the short exact sequence of complexes
induces the long exact sequence
By inductive hypothesis,
![]() ${\mathcal{F}}^{\prime }$
is acyclic, that is,
${\mathcal{F}}^{\prime }$
is acyclic, that is,
![]() $H_{i}({\mathcal{F}}^{\prime })=0$
for all
$H_{i}({\mathcal{F}}^{\prime })=0$
for all
![]() $i>0$
. Also the multiplication map is injective. It then follows that
$i>0$
. Also the multiplication map is injective. It then follows that
![]() $H_{i}({\mathcal{F}})\cong H_{i}({\mathcal{G}})$
for all
$H_{i}({\mathcal{F}})\cong H_{i}({\mathcal{G}})$
for all
![]() $i>0$
. We prove
$i>0$
. We prove
![]() $H_{i}({\mathcal{G}})=0$
for all
$H_{i}({\mathcal{G}})=0$
for all
![]() $i>0$
.
$i>0$
.
We consider the induced subgraph
![]() $G^{\prime \prime }:=G_{V\setminus (\{x_{1}\}\cup N_{G}(x_{1}))}$
. Let
$G^{\prime \prime }:=G_{V\setminus (\{x_{1}\}\cup N_{G}(x_{1}))}$
. Let
![]() $G_{0}^{\prime \prime }:=G^{\prime \prime }\setminus \{\text{isolated vertices of }G^{\prime \prime }\}$
. It was proved by Mahmoudi et al. in [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Proof of Theorem 3.2] that
$G_{0}^{\prime \prime }:=G^{\prime \prime }\setminus \{\text{isolated vertices of }G^{\prime \prime }\}$
. It was proved by Mahmoudi et al. in [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Proof of Theorem 3.2] that
![]() $G_{0}^{\prime \prime }$
is also a Cohen–Macaulay very well-covered graph with respect to the induced labeling of the vertices from
$G_{0}^{\prime \prime }$
is also a Cohen–Macaulay very well-covered graph with respect to the induced labeling of the vertices from
![]() $G$
. Let
$G$
. Let
![]() $({\mathcal{F}}^{\prime \prime },\unicode[STIX]{x2202}^{\prime \prime })$
denote the complex corresponding to
$({\mathcal{F}}^{\prime \prime },\unicode[STIX]{x2202}^{\prime \prime })$
denote the complex corresponding to
![]() $G_{0}^{\prime \prime }$
. Similarly to the case of
$G_{0}^{\prime \prime }$
. Similarly to the case of
![]() $({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
, we do not distinguish
$({\mathcal{F}}^{\prime },\unicode[STIX]{x2202}^{\prime })$
, we do not distinguish
![]() $({\mathcal{F}}^{\prime \prime },\unicode[STIX]{x2202}^{\prime \prime })$
with
$({\mathcal{F}}^{\prime \prime },\unicode[STIX]{x2202}^{\prime \prime })$
with
![]() $({\mathcal{F}}^{\prime \prime }\otimes K[V(G)],\unicode[STIX]{x2202}^{\prime \prime }\otimes 1)$
. Let
$({\mathcal{F}}^{\prime \prime }\otimes K[V(G)],\unicode[STIX]{x2202}^{\prime \prime }\otimes 1)$
. Let
![]() ${\mathcal{C}}_{\bullet }$
denote the mapping cone of the complex homomorphism
${\mathcal{C}}_{\bullet }$
denote the mapping cone of the complex homomorphism
Then we have a short exact sequence of complexes
where
![]() $({\mathcal{F}}^{\prime \prime }[-1])_{i}:={\mathcal{F}}_{i-1}^{\prime \prime }$
. This induces the long exact sequence
$({\mathcal{F}}^{\prime \prime }[-1])_{i}:={\mathcal{F}}_{i-1}^{\prime \prime }$
. This induces the long exact sequence
 $$\begin{eqnarray}\displaystyle & \displaystyle \cdots \longrightarrow H_{2}({\mathcal{C}})\longrightarrow H_{1}({\mathcal{F}}^{\prime \prime }) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \stackrel{\cdot -x_{1}}{\longrightarrow }H_{1}({\mathcal{F}}^{\prime \prime })\longrightarrow H_{1}({\mathcal{C}})\longrightarrow H_{0}({\mathcal{F}}^{\prime \prime }) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \stackrel{\cdot -x_{1}}{\longrightarrow }H_{0}({\mathcal{F}}^{\prime \prime })\longrightarrow H_{0}({\mathcal{C}})\longrightarrow 0. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \cdots \longrightarrow H_{2}({\mathcal{C}})\longrightarrow H_{1}({\mathcal{F}}^{\prime \prime }) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \stackrel{\cdot -x_{1}}{\longrightarrow }H_{1}({\mathcal{F}}^{\prime \prime })\longrightarrow H_{1}({\mathcal{C}})\longrightarrow H_{0}({\mathcal{F}}^{\prime \prime }) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \stackrel{\cdot -x_{1}}{\longrightarrow }H_{0}({\mathcal{F}}^{\prime \prime })\longrightarrow H_{0}({\mathcal{C}})\longrightarrow 0. & \displaystyle \nonumber\end{eqnarray}$$
By inductive hypothesis, the complex
![]() ${\mathcal{F}}^{\prime \prime }$
is also acyclic, and the multiplication map
${\mathcal{F}}^{\prime \prime }$
is also acyclic, and the multiplication map
![]() $J(G^{\prime \prime })=H_{0}({\mathcal{F}}^{\prime \prime })\stackrel{\cdot -x_{1}}{\longrightarrow }H_{0}({\mathcal{F}}^{\prime \prime })=J(G^{\prime \prime })$
is injective, we have
$J(G^{\prime \prime })=H_{0}({\mathcal{F}}^{\prime \prime })\stackrel{\cdot -x_{1}}{\longrightarrow }H_{0}({\mathcal{F}}^{\prime \prime })=J(G^{\prime \prime })$
is injective, we have
![]() $H_{i}({\mathcal{C}})=0$
for
$H_{i}({\mathcal{C}})=0$
for
![]() $i\geqslant 1$
.
$i\geqslant 1$
.
Finally we prove that
![]() ${\mathcal{C}}\cong {\mathcal{G}}$
. Note that
${\mathcal{C}}\cong {\mathcal{G}}$
. Note that
where
![]() ${{\mathcal{F}}^{\prime \prime }}_{-1}=0$
. Set
${{\mathcal{F}}^{\prime \prime }}_{-1}=0$
. Set
![]() ${\mathcal{L}}^{\prime \prime }=\{\unicode[STIX]{x1D6FA}_{A^{\prime \prime }}^{\prime \prime }:A^{\prime \prime }\in \mathfrak{d}_{G_{0}^{\prime \prime }}\}$
. Then the free basis of
${\mathcal{L}}^{\prime \prime }=\{\unicode[STIX]{x1D6FA}_{A^{\prime \prime }}^{\prime \prime }:A^{\prime \prime }\in \mathfrak{d}_{G_{0}^{\prime \prime }}\}$
. Then the free basis of
![]() ${\mathcal{C}}_{\ell }$
is
${\mathcal{C}}_{\ell }$
is
![]() $\mathfrak{C}_{\ell }=\mathfrak{B}_{\ell -1}\cup \mathfrak{B}_{\ell }$
where
$\mathfrak{C}_{\ell }=\mathfrak{B}_{\ell -1}\cup \mathfrak{B}_{\ell }$
where
On the other hand, the free basis of
![]() ${\mathcal{G}}_{\ell }$
is
${\mathcal{G}}_{\ell }$
is
We define
![]() $K[V(G)]$
-linear homomorphism
$K[V(G)]$
-linear homomorphism
![]() $\unicode[STIX]{x1D713}_{\ell }:{\mathcal{C}}_{\ell }\longrightarrow {\mathcal{G}}_{\ell }$
by
$\unicode[STIX]{x1D713}_{\ell }:{\mathcal{C}}_{\ell }\longrightarrow {\mathcal{G}}_{\ell }$
by
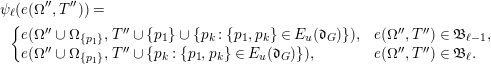 $$\begin{eqnarray}\displaystyle & & \displaystyle \!\!\!\unicode[STIX]{x1D713}_{\ell }(e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime }))=\nonumber\\ \displaystyle & & \displaystyle \left\{\begin{array}{@{}ll@{}}e(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}},T^{\prime \prime }\cup \{p_{1}\}\cup \{p_{k}:\{p_{1},p_{k}\}\in E_{u}(\mathfrak{d}_{G})\}), & e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime })\in \mathfrak{B}_{\ell -1},\\ e(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}},T^{\prime \prime }\cup \{p_{k}:\{p_{1},p_{k}\}\in E_{u}(\mathfrak{d}_{G})\}), & e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime })\in \mathfrak{B}_{\ell }.\end{array}\right.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \!\!\!\unicode[STIX]{x1D713}_{\ell }(e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime }))=\nonumber\\ \displaystyle & & \displaystyle \left\{\begin{array}{@{}ll@{}}e(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}},T^{\prime \prime }\cup \{p_{1}\}\cup \{p_{k}:\{p_{1},p_{k}\}\in E_{u}(\mathfrak{d}_{G})\}), & e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime })\in \mathfrak{B}_{\ell -1},\\ e(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}},T^{\prime \prime }\cup \{p_{k}:\{p_{1},p_{k}\}\in E_{u}(\mathfrak{d}_{G})\}), & e(\unicode[STIX]{x1D6FA}^{\prime \prime },T^{\prime \prime })\in \mathfrak{B}_{\ell }.\end{array}\right.\nonumber\end{eqnarray}$$
Then
![]() $\unicode[STIX]{x1D713}_{\ell }$
is well-defined. Note that
$\unicode[STIX]{x1D713}_{\ell }$
is well-defined. Note that
![]() $A(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}})=A(\unicode[STIX]{x1D6FA}^{\prime \prime })\cup \{p_{1}\}$
and
$A(\unicode[STIX]{x1D6FA}^{\prime \prime }\cup \unicode[STIX]{x1D6FA}_{\{p_{1}\}})=A(\unicode[STIX]{x1D6FA}^{\prime \prime })\cup \{p_{1}\}$
and
![]() $T^{\prime \prime }\cap \{p_{1}\}=\emptyset$
. It is easy to see that all
$T^{\prime \prime }\cap \{p_{1}\}=\emptyset$
. It is easy to see that all
![]() $\unicode[STIX]{x1D713}_{\ell }$
are bijective and
$\unicode[STIX]{x1D713}_{\ell }$
are bijective and
![]() $(\unicode[STIX]{x1D713}_{\ell })$
induces an isomorphism of complexes.◻
$(\unicode[STIX]{x1D713}_{\ell })$
induces an isomorphism of complexes.◻
Remark 3.3. In [Reference Mahmoudi, Mousivand, Crupi, Rinaldo, Terai and Yassemi11, Lemma 3.4], Mahmoudi et al. characterized the regularity of a Cohen–Macaulay very well-covered graph by using
![]() $G^{\prime }$
and
$G^{\prime }$
and
![]() $G^{\prime \prime }$
(the notations are
$G^{\prime \prime }$
(the notations are
![]() $G_{2}$
,
$G_{2}$
,
![]() $G_{1}$
, respectively).
$G_{1}$
, respectively).
By the above explicit minimal free resolution of
![]() $J(G)$
, we obtain the nonzero extremal Betti numbers of
$J(G)$
, we obtain the nonzero extremal Betti numbers of
![]() $J(G)$
. Recall that
$J(G)$
. Recall that
![]() $\unicode[STIX]{x1D6FD}_{i,\unicode[STIX]{x1D70E}}(J(G))$
is extremal if
$\unicode[STIX]{x1D6FD}_{i,\unicode[STIX]{x1D70E}}(J(G))$
is extremal if
![]() $\unicode[STIX]{x1D6FD}_{j,\unicode[STIX]{x1D70F}}(J(G))=0$
for all
$\unicode[STIX]{x1D6FD}_{j,\unicode[STIX]{x1D70F}}(J(G))=0$
for all
![]() $j\geqslant i$
and
$j\geqslant i$
and
![]() $\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
with
$\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
with
![]() $|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant j-i$
, where
$|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant j-i$
, where
![]() $|\unicode[STIX]{x1D70E}|=\unicode[STIX]{x1D70E}_{1}+\cdots +\unicode[STIX]{x1D70E}_{n}$
for
$|\unicode[STIX]{x1D70E}|=\unicode[STIX]{x1D70E}_{1}+\cdots +\unicode[STIX]{x1D70E}_{n}$
for
![]() $\unicode[STIX]{x1D70E}\in \mathbb{N}^{n}$
.
$\unicode[STIX]{x1D70E}\in \mathbb{N}^{n}$
.
Corollary 3.4. The free basis
![]() $e(\unicode[STIX]{x1D6FA},T)$
corresponds to an extremal Betti number of
$e(\unicode[STIX]{x1D6FA},T)$
corresponds to an extremal Betti number of
![]() $J(G)$
if and only if the following two conditions are satisfied:
$J(G)$
if and only if the following two conditions are satisfied:
(i)
 $A(\unicode[STIX]{x1D6FA})$
is a facet of
$A(\unicode[STIX]{x1D6FA})$
is a facet of
 $\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
;
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
;(ii)
 $\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
.
$\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
.
Proof. We use the same notation as above.
We first assume that
![]() $e(\unicode[STIX]{x1D6FA},T)$
corresponds to an extremal Betti number of
$e(\unicode[STIX]{x1D6FA},T)$
corresponds to an extremal Betti number of
![]() $J(G)$
. Suppose
$J(G)$
. Suppose
![]() $\unicode[STIX]{x1D6FA}\cap T\neq A(\unicode[STIX]{x1D6FA})$
. We set
$\unicode[STIX]{x1D6FA}\cap T\neq A(\unicode[STIX]{x1D6FA})$
. We set
![]() $A^{\prime }:=A(\unicode[STIX]{x1D6FA})\setminus (\unicode[STIX]{x1D6FA}\cap T)$
and
$A^{\prime }:=A(\unicode[STIX]{x1D6FA})\setminus (\unicode[STIX]{x1D6FA}\cap T)$
and
![]() $S:=T\cup A^{\prime }$
. Then the degree of
$S:=T\cup A^{\prime }$
. Then the degree of
![]() $e(\unicode[STIX]{x1D6FA},S)$
is
$e(\unicode[STIX]{x1D6FA},S)$
is
 $$\begin{eqnarray}\displaystyle \deg e(\unicode[STIX]{x1D6FA},S) & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap S}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}\mathop{\prod }_{p_{i}\in A^{\prime }}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg e(\unicode[STIX]{x1D6FA},T)+\deg \mathop{\prod }_{p_{i}\in A^{\prime }}x_{i}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \deg e(\unicode[STIX]{x1D6FA},S) & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap S}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}\mathop{\prod }_{p_{i}\in A^{\prime }}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg e(\unicode[STIX]{x1D6FA},T)+\deg \mathop{\prod }_{p_{i}\in A^{\prime }}x_{i}.\nonumber\end{eqnarray}$$
Hence
![]() $e(\unicode[STIX]{x1D6FA},T)$
does not correspond to an extremal Betti number, a contradiction. Therefore, we assume
$e(\unicode[STIX]{x1D6FA},T)$
does not correspond to an extremal Betti number, a contradiction. Therefore, we assume
![]() $\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
. Suppose that
$\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
. Suppose that
![]() $A(\unicode[STIX]{x1D6FA})$
is not a facet. Then there exists
$A(\unicode[STIX]{x1D6FA})$
is not a facet. Then there exists
![]() $p_{i}\in V(\mathfrak{d}_{G})$
with
$p_{i}\in V(\mathfrak{d}_{G})$
with
![]() $A(\unicode[STIX]{x1D6FA})\cup \{p_{i}\}\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. Let
$A(\unicode[STIX]{x1D6FA})\cup \{p_{i}\}\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. Let
![]() $p_{i_{0}}$
be such a vertex with maximum index. Set
$p_{i_{0}}$
be such a vertex with maximum index. Set
![]() $\unicode[STIX]{x1D6E9}:=\unicode[STIX]{x1D6FA}_{A(\unicode[STIX]{x1D6FA})\cup \{p_{i_{0}}\}}$
and
$\unicode[STIX]{x1D6E9}:=\unicode[STIX]{x1D6FA}_{A(\unicode[STIX]{x1D6FA})\cup \{p_{i_{0}}\}}$
and
![]() $S=T\cup \{p_{i_{0}}\}$
. We claim
$S=T\cup \{p_{i_{0}}\}$
. We claim
![]() $\unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}\cup \{p_{i_{0}}\}$
. Indeed,
$\unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}\cup \{p_{i_{0}}\}$
. Indeed,
![]() $\unicode[STIX]{x1D6E9}\supset \unicode[STIX]{x1D6FA}\cup \{p_{i_{0}}\}$
is obvious. Conversely, assume
$\unicode[STIX]{x1D6E9}\supset \unicode[STIX]{x1D6FA}\cup \{p_{i_{0}}\}$
is obvious. Conversely, assume
![]() $p_{k}\in \unicode[STIX]{x1D6E9}$
. If
$p_{k}\in \unicode[STIX]{x1D6E9}$
. If
![]() $p_{k}\succcurlyeq p_{\ell }$
for some
$p_{k}\succcurlyeq p_{\ell }$
for some
![]() $p_{\ell }\in A(\unicode[STIX]{x1D6FA})$
, then
$p_{\ell }\in A(\unicode[STIX]{x1D6FA})$
, then
![]() $p_{k}\in \unicode[STIX]{x1D6FA}$
. Otherwise, by applying Lemma 2.4 to
$p_{k}\in \unicode[STIX]{x1D6FA}$
. Otherwise, by applying Lemma 2.4 to
![]() $\unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}_{A(\unicode[STIX]{x1D6FA})\cup \{p_{i_{0}}\}}$
, we have
$\unicode[STIX]{x1D6E9}=\unicode[STIX]{x1D6FA}_{A(\unicode[STIX]{x1D6FA})\cup \{p_{i_{0}}\}}$
, we have
![]() $\{p_{k}\}\cup A(\unicode[STIX]{x1D6FA})\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. On the other hand,
$\{p_{k}\}\cup A(\unicode[STIX]{x1D6FA})\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
. On the other hand,
![]() $p_{k}\succcurlyeq p_{i_{0}}$
also satisfied. In particular
$p_{k}\succcurlyeq p_{i_{0}}$
also satisfied. In particular
![]() $i_{0}\leqslant k$
. Then the maximality of
$i_{0}\leqslant k$
. Then the maximality of
![]() $i_{0}$
implies that
$i_{0}$
implies that
![]() $p_{i_{0}}=p_{k}$
, as desired. Hence
$p_{i_{0}}=p_{k}$
, as desired. Hence
 $$\begin{eqnarray}\displaystyle \deg e(\unicode[STIX]{x1D6E9},S) & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6E9}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}\cap S}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg y_{i_{0}}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg e(\unicode[STIX]{x1D6FA},T)+\deg y_{i_{0}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \deg e(\unicode[STIX]{x1D6E9},S) & = & \displaystyle \deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6E9}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}\cap S}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg y_{i_{0}}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}\nonumber\\ \displaystyle & = & \displaystyle \deg e(\unicode[STIX]{x1D6FA},T)+\deg y_{i_{0}}.\nonumber\end{eqnarray}$$
This is also a contradiction.
Next, let
![]() $e(\unicode[STIX]{x1D6FA},T)$
be a free basis of
$e(\unicode[STIX]{x1D6FA},T)$
be a free basis of
![]() ${\mathcal{F}}_{\ell }$
of degree
${\mathcal{F}}_{\ell }$
of degree
![]() $\unicode[STIX]{x1D70E}$
satisfying the conditions (i), (ii). Suppose that there is a free basis
$\unicode[STIX]{x1D70E}$
satisfying the conditions (i), (ii). Suppose that there is a free basis
![]() $e(\unicode[STIX]{x1D6E9},S)$
of
$e(\unicode[STIX]{x1D6E9},S)$
of
![]() ${\mathcal{F}}_{k}$
of degree
${\mathcal{F}}_{k}$
of degree
![]() $\unicode[STIX]{x1D70F}$
with
$\unicode[STIX]{x1D70F}$
with
![]() $k\geqslant \ell$
,
$k\geqslant \ell$
,
![]() $\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
, and
$\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
, and
![]() $|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant k-\ell$
. Note that
$|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant k-\ell$
. Note that
![]() $J(G)$
has a linear resolution, and thus
$J(G)$
has a linear resolution, and thus
![]() $|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|=k-\ell$
. Since
$|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|=k-\ell$
. Since
 $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70E}=\deg e(\unicode[STIX]{x1D6FA},T)=\deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D70F}=\deg e(\unicode[STIX]{x1D6E9},S)=\deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6E9}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}\cap S}x_{i} & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70E}=\deg e(\unicode[STIX]{x1D6FA},T)=\deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6FA}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6FA}\cap T}x_{i}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D70F}=\deg e(\unicode[STIX]{x1D6E9},S)=\deg \mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}}y_{i}\mathop{\prod }_{p_{i}\notin \unicode[STIX]{x1D6E9}}x_{i}\mathop{\prod }_{p_{i}\in \unicode[STIX]{x1D6E9}\cap S}x_{i} & \displaystyle \nonumber\end{eqnarray}$$
and
![]() $\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
, it follows that
$\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
, it follows that
By
![]() $\unicode[STIX]{x1D6FA}\subset \unicode[STIX]{x1D6E9}$
, we have
$\unicode[STIX]{x1D6FA}\subset \unicode[STIX]{x1D6E9}$
, we have
![]() $(V(\mathfrak{d}_{G})\setminus \unicode[STIX]{x1D6E9})\cap \unicode[STIX]{x1D6FA}=\emptyset$
. Hence
$(V(\mathfrak{d}_{G})\setminus \unicode[STIX]{x1D6E9})\cap \unicode[STIX]{x1D6FA}=\emptyset$
. Hence
![]() $\unicode[STIX]{x1D6FA}\cap T\subset \unicode[STIX]{x1D6E9}\cap S$
. Then
$\unicode[STIX]{x1D6FA}\cap T\subset \unicode[STIX]{x1D6E9}\cap S$
. Then
![]() $A(\unicode[STIX]{x1D6E9})\supset \unicode[STIX]{x1D6E9}\cap S\supset \unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
, where the last equality follows from the condition (ii). Since
$A(\unicode[STIX]{x1D6E9})\supset \unicode[STIX]{x1D6E9}\cap S\supset \unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
, where the last equality follows from the condition (ii). Since
![]() $A(\unicode[STIX]{x1D6E9}),A(\unicode[STIX]{x1D6FA})\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
$A(\unicode[STIX]{x1D6E9}),A(\unicode[STIX]{x1D6FA})\in \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
![]() $A(\unicode[STIX]{x1D6FA})$
is a facet of
$A(\unicode[STIX]{x1D6FA})$
is a facet of
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
by (i), we have
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
by (i), we have
![]() $A(\unicode[STIX]{x1D6FA})=A(\unicode[STIX]{x1D6E9})$
. Then it also follows that
$A(\unicode[STIX]{x1D6FA})=A(\unicode[STIX]{x1D6E9})$
. Then it also follows that
![]() $\unicode[STIX]{x1D6FA}=\unicode[STIX]{x1D6E9}$
. Again by (ii), this contradicts to
$\unicode[STIX]{x1D6FA}=\unicode[STIX]{x1D6E9}$
. Again by (ii), this contradicts to
![]() $\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
.◻
$\unicode[STIX]{x1D70F}\succ \unicode[STIX]{x1D70E}$
.◻
Now we can prove Theorem 1.1 for a Cohen–Macaulay very well-covered graph by showing the following proposition and using the Alexander duality:
![]() $\operatorname{pd}S/I(G)=\operatorname{reg}J(G)$
given by Terai[Reference Terai18].
$\operatorname{pd}S/I(G)=\operatorname{reg}J(G)$
given by Terai[Reference Terai18].
Proposition 3.5. Let
![]() $G$
be a very well-covered graph. If
$G$
be a very well-covered graph. If
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(G))\neq 0$
is extremal, then there exists a pairwise
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(G))\neq 0$
is extremal, then there exists a pairwise
![]() $3$
-disjoint set
$3$
-disjoint set
![]() ${\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}$
of complete bipartite subgraph of
${\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}$
of complete bipartite subgraph of
![]() $G$
with
$G$
with
![]() $V({\mathcal{B}})=\unicode[STIX]{x1D70E}$
.
$V({\mathcal{B}})=\unicode[STIX]{x1D70E}$
.
Corollary 3.6. Let
![]() $G$
be a Cohen–Macaulay very well-covered graph. Then
$G$
be a Cohen–Macaulay very well-covered graph. Then
 $$\begin{eqnarray}\displaystyle & & \displaystyle \operatorname{pd}S/I(G)=\operatorname{height}I(G)\nonumber\\ \displaystyle & & \displaystyle \quad =\max \left\{\#V({\mathcal{B}})-r:\begin{array}{@{}l@{}}{\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}\text{is a pairwise 3-disjoint set}\\ \text{of complete bipartite subgraphs of }G\end{array}\right\}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \operatorname{pd}S/I(G)=\operatorname{height}I(G)\nonumber\\ \displaystyle & & \displaystyle \quad =\max \left\{\#V({\mathcal{B}})-r:\begin{array}{@{}l@{}}{\mathcal{B}}=\{B_{1},\ldots ,B_{r}\}\text{is a pairwise 3-disjoint set}\\ \text{of complete bipartite subgraphs of }G\end{array}\right\}.\nonumber\end{eqnarray}$$
Since
![]() $J(G)$
is squarefree, we only need to consider the case where the degree
$J(G)$
is squarefree, we only need to consider the case where the degree
![]() $\unicode[STIX]{x1D70E}$
is a
$\unicode[STIX]{x1D70E}$
is a
![]() $(0,1)$
-vector because otherwise
$(0,1)$
-vector because otherwise
![]() $\unicode[STIX]{x1D6FD}_{i,\unicode[STIX]{x1D70E}}(J(G))=0$
. Hence we sometimes identify the degree
$\unicode[STIX]{x1D6FD}_{i,\unicode[STIX]{x1D70E}}(J(G))=0$
. Hence we sometimes identify the degree
![]() $\unicode[STIX]{x1D70E}$
with a subset of
$\unicode[STIX]{x1D70E}$
with a subset of
![]() $V(G)$
.
$V(G)$
.
Proof of Proposition 3.5.
Assume that
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(G))\neq 0$
is extremal. Then there exists a free basis
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(G))\neq 0$
is extremal. Then there exists a free basis
![]() $e(\unicode[STIX]{x1D6FA},T)$
with
$e(\unicode[STIX]{x1D6FA},T)$
with
![]() $\#(\unicode[STIX]{x1D6FA}\cap T)=r$
and
$\#(\unicode[STIX]{x1D6FA}\cap T)=r$
and
![]() $\deg e(\unicode[STIX]{x1D6FA},T)=\unicode[STIX]{x1D70E}$
. Also by Corollary 3.4,
$\deg e(\unicode[STIX]{x1D6FA},T)=\unicode[STIX]{x1D70E}$
. Also by Corollary 3.4,
![]() $A(\unicode[STIX]{x1D6FA})$
is a facet of
$A(\unicode[STIX]{x1D6FA})$
is a facet of
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
![]() $\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
holds. Note that
$\unicode[STIX]{x1D6FA}\cap T=A(\unicode[STIX]{x1D6FA})$
holds. Note that
Set
![]() $A(\unicode[STIX]{x1D6FA}):=\{p_{\ell _{1}},\ldots ,p_{\ell _{r}}\}$
. Since
$A(\unicode[STIX]{x1D6FA}):=\{p_{\ell _{1}},\ldots ,p_{\ell _{r}}\}$
. Since
![]() $A(\unicode[STIX]{x1D6FA})$
is independent in
$A(\unicode[STIX]{x1D6FA})$
is independent in
![]() $\mathfrak{d}_{G}$
, it follows that
$\mathfrak{d}_{G}$
, it follows that
![]() $\{x_{\ell _{1}},y_{\ell _{1}}\},\ldots ,\{x_{\ell _{r}},y_{\ell _{r}}\}$
are pairwise
$\{x_{\ell _{1}},y_{\ell _{1}}\},\ldots ,\{x_{\ell _{r}},y_{\ell _{r}}\}$
are pairwise
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $G$
. We define
$G$
. We define
![]() $V_{1}$
to be the set of
$V_{1}$
to be the set of
![]() $z\in V(G)$
which divides
$z\in V(G)$
which divides
![]() $u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
$u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
![]() $\{z,x_{\ell _{1}}\},\{z,y_{\ell _{1}}\}$
is an edge of
$\{z,x_{\ell _{1}}\},\{z,y_{\ell _{1}}\}$
is an edge of
![]() $G$
. Next we define
$G$
. Next we define
![]() $V_{2}$
to be the set of
$V_{2}$
to be the set of
![]() $z\in V(G)\setminus V_{1}$
which divides
$z\in V(G)\setminus V_{1}$
which divides
![]() $u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
$u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
![]() $\{z,x_{\ell _{2}}\},\{z,y_{\ell _{2}}\}$
is an edge of
$\{z,x_{\ell _{2}}\},\{z,y_{\ell _{2}}\}$
is an edge of
![]() $G$
. Similarly, we define
$G$
. Similarly, we define
![]() $V_{k}$
to be the set of
$V_{k}$
to be the set of
![]() $z\in V(G)\setminus (V_{1}\cup \cdots \cup V_{k-1})$
which divides
$z\in V(G)\setminus (V_{1}\cup \cdots \cup V_{k-1})$
which divides
![]() $u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
$u_{A(\unicode[STIX]{x1D6FA}),T}$
and one of
![]() $\{z,x_{\ell _{k}}\},\{z,y_{\ell _{k}}\}$
is an edge of
$\{z,x_{\ell _{k}}\},\{z,y_{\ell _{k}}\}$
is an edge of
![]() $G$
. As a result, we obtain
$G$
. As a result, we obtain
![]() $V_{1},\ldots ,V_{r}$
. Note that
$V_{1},\ldots ,V_{r}$
. Note that
![]() $x_{\ell _{k}},y_{\ell _{k}}\in V_{k}$
.
$x_{\ell _{k}},y_{\ell _{k}}\in V_{k}$
.
We prove the following
![]() $2$
claims which derive the proposition:
$2$
claims which derive the proposition:
Claim 1.
![]() $\unicode[STIX]{x1D70E}=V_{1}\sqcup \cdots \sqcup V_{r}$
.
$\unicode[STIX]{x1D70E}=V_{1}\sqcup \cdots \sqcup V_{r}$
.
Claim 2.
![]() $G_{V_{k}}$
contains a complete bipartite graph as a spanning subgraph.
$G_{V_{k}}$
contains a complete bipartite graph as a spanning subgraph.
Proof of Claim 1. It is clear that
![]() $V_{\ell }\cap V_{k}\neq \emptyset$
if
$V_{\ell }\cap V_{k}\neq \emptyset$
if
![]() $k\neq \ell$
. Also
$k\neq \ell$
. Also
We set
![]() $\unicode[STIX]{x1D70E}_{0}:=\unicode[STIX]{x1D70E}\setminus \{x_{\ell _{1}},\ldots ,x_{\ell _{r}},y_{\ell _{1}},\ldots ,y_{\ell _{r}}\}$
. We prove
$\unicode[STIX]{x1D70E}_{0}:=\unicode[STIX]{x1D70E}\setminus \{x_{\ell _{1}},\ldots ,x_{\ell _{r}},y_{\ell _{1}},\ldots ,y_{\ell _{r}}\}$
. We prove
![]() $\unicode[STIX]{x1D70E}_{0}\subset V_{1}\cup \cdots \cup V_{r}$
. Then Claim 1 follows.
$\unicode[STIX]{x1D70E}_{0}\subset V_{1}\cup \cdots \cup V_{r}$
. Then Claim 1 follows.
If
![]() $y_{\ell }\in \unicode[STIX]{x1D70E}_{0}$
, then
$y_{\ell }\in \unicode[STIX]{x1D70E}_{0}$
, then
![]() $p_{\ell }\in \unicode[STIX]{x1D6FA}$
. Therefore,
$p_{\ell }\in \unicode[STIX]{x1D6FA}$
. Therefore,
![]() $p_{\ell }\succ p_{\ell _{k}}$
for some
$p_{\ell }\succ p_{\ell _{k}}$
for some
![]() $p_{\ell _{k}}\in A(\unicode[STIX]{x1D6FA})$
. This implies
$p_{\ell _{k}}\in A(\unicode[STIX]{x1D6FA})$
. This implies
![]() $\{x_{\ell _{k}},y_{\ell }\}\in E(G)$
. Therefore,
$\{x_{\ell _{k}},y_{\ell }\}\in E(G)$
. Therefore,
![]() $y_{\ell }\in V_{1}\cup \cdots \cup V_{k}$
.
$y_{\ell }\in V_{1}\cup \cdots \cup V_{k}$
.
If
![]() $x_{\ell }\in \unicode[STIX]{x1D70E}_{0}$
, then
$x_{\ell }\in \unicode[STIX]{x1D70E}_{0}$
, then
![]() $p_{\ell }\notin \unicode[STIX]{x1D6FA}$
. Note that
$p_{\ell }\notin \unicode[STIX]{x1D6FA}$
. Note that
![]() $p_{\ell }\notin A(\unicode[STIX]{x1D6FA})$
. Since
$p_{\ell }\notin A(\unicode[STIX]{x1D6FA})$
. Since
![]() $A(\unicode[STIX]{x1D6FA})$
is a facet of
$A(\unicode[STIX]{x1D6FA})$
is a facet of
![]() $\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
$\unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
and
![]() $A(\unicode[STIX]{x1D6FA})\subsetneq A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}$
, it follows that
$A(\unicode[STIX]{x1D6FA})\subsetneq A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}$
, it follows that
![]() $A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}\notin \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
, in other words,
$A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}\notin \unicode[STIX]{x1D6E5}_{\mathfrak{d}_{G}}$
, in other words,
![]() $A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}$
is not independent in
$A(\unicode[STIX]{x1D6FA})\cup \{p_{\ell }\}$
is not independent in
![]() $\mathfrak{d}_{G}$
. Since
$\mathfrak{d}_{G}$
. Since
![]() $p_{\ell }\notin \unicode[STIX]{x1D6FA}$
, it follows that
$p_{\ell }\notin \unicode[STIX]{x1D6FA}$
, it follows that
![]() $p_{\ell }p_{\ell _{k}}\in E_{d}(\mathfrak{d}_{G})$
for some
$p_{\ell }p_{\ell _{k}}\in E_{d}(\mathfrak{d}_{G})$
for some
![]() $k$
or
$k$
or
![]() $\{p_{\ell },p_{\ell _{k}}\}\in E_{u}(\mathfrak{d}_{G})$
for some
$\{p_{\ell },p_{\ell _{k}}\}\in E_{u}(\mathfrak{d}_{G})$
for some
![]() $k$
. In the former case, we have
$k$
. In the former case, we have
![]() $\{x_{\ell },y_{\ell _{k}}\}\in E(G)$
, and the latter case, we have
$\{x_{\ell },y_{\ell _{k}}\}\in E(G)$
, and the latter case, we have
![]() $\{x_{\ell },x_{\ell _{k}}\}\in E(G)$
. Thus
$\{x_{\ell },x_{\ell _{k}}\}\in E(G)$
. Thus
![]() $x_{\ell }\in V_{1}\cup \cdots \cup V_{k}$
.
$x_{\ell }\in V_{1}\cup \cdots \cup V_{k}$
.
Proof of Claim 2. For each
![]() $k$
, we set
$k$
, we set
Note that
![]() $V_{1k}\cap V_{2k}=\emptyset$
by the condition (iv) of Theorem 2.1. Also
$V_{1k}\cap V_{2k}=\emptyset$
by the condition (iv) of Theorem 2.1. Also
![]() $V_{1k}\cup V_{2k}=V_{k}$
holds. Take
$V_{1k}\cup V_{2k}=V_{k}$
holds. Take
![]() $z\in V_{1k}$
and
$z\in V_{1k}$
and
![]() $x_{\ell }\in V_{2k}$
. Since
$x_{\ell }\in V_{2k}$
. Since
![]() $\{z,x_{\ell _{k}}\},\{x_{\ell },y_{\ell _{k}}\}\in E(G)$
, we have
$\{z,x_{\ell _{k}}\},\{x_{\ell },y_{\ell _{k}}\}\in E(G)$
, we have
![]() $\{z,x_{\ell }\}\in E(G)$
by the condition (iii) of Theorem 2.1.◻
$\{z,x_{\ell }\}\in E(G)$
by the condition (iii) of Theorem 2.1.◻
4 Proof of the main theorem
In this section we prove Theorem 1.1. We use arguments similar to that of [Reference Kimura9, Theorem 7.1].
First, we recall some notation. Let
![]() $G$
be a very well-covered graph on
$G$
be a very well-covered graph on
![]() $V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) of Theorem 2.1. Then we obtain the transitively closed semidirected graph
$V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) of Theorem 2.1. Then we obtain the transitively closed semidirected graph
![]() $\mathfrak{d}_{G}$
on the vertex set
$\mathfrak{d}_{G}$
on the vertex set
![]() $\{p_{1},\ldots ,p_{h}\}$
. Let
$\{p_{1},\ldots ,p_{h}\}$
. Let
![]() ${\mathcal{Z}}_{1},\ldots ,{\mathcal{Z}}_{t}$
be all strongly connected components of
${\mathcal{Z}}_{1},\ldots ,{\mathcal{Z}}_{t}$
be all strongly connected components of
![]() $\mathfrak{d}_{G}$
. Then we obtain the acyclic reduction
$\mathfrak{d}_{G}$
. Then we obtain the acyclic reduction
![]() $\widehat{\mathfrak{d}}_{G}$
of
$\widehat{\mathfrak{d}}_{G}$
of
![]() $\mathfrak{d}_{G}$
, whose vertex set is
$\mathfrak{d}_{G}$
, whose vertex set is
![]() $\{q_{1},\ldots ,q_{t}\}$
. The semidirected graph
$\{q_{1},\ldots ,q_{t}\}$
. The semidirected graph
![]() $\widehat{\mathfrak{d}}_{G}$
corresponds to the acyclic reduction
$\widehat{\mathfrak{d}}_{G}$
corresponds to the acyclic reduction
![]() $\widehat{G}$
of
$\widehat{G}$
of
![]() $G$
. Note that
$G$
. Note that
![]() $\widehat{G}$
is a Cohen–Macaulay very well-covered graph and we may assume that the vertex set of
$\widehat{G}$
is a Cohen–Macaulay very well-covered graph and we may assume that the vertex set of
![]() $\widehat{G}$
is
$\widehat{G}$
is
![]() $\{u_{1},\ldots ,u_{t},v_{1},\ldots ,v_{t}\}$
satisfying (i), (ii), (iii), (iv) of Theorem 2.1 and (v) of Theorem 2.2 (though we need to replace notation
$\{u_{1},\ldots ,u_{t},v_{1},\ldots ,v_{t}\}$
satisfying (i), (ii), (iii), (iv) of Theorem 2.1 and (v) of Theorem 2.2 (though we need to replace notation
![]() $x_{i},y_{j},z_{k}$
by
$x_{i},y_{j},z_{k}$
by
![]() $u_{i},v_{j},w_{k}$
, respectively). Also we set
$u_{i},v_{j},w_{k}$
, respectively). Also we set
![]() $\unicode[STIX]{x1D701}_{a}=\#{\mathcal{Z}}_{a}$
. Moreover, for
$\unicode[STIX]{x1D701}_{a}=\#{\mathcal{Z}}_{a}$
. Moreover, for
![]() $\unicode[STIX]{x1D70E}=\prod _{a}u_{a}^{s_{a}}\prod _{b}v_{b}^{r_{b}}$
, we set
$\unicode[STIX]{x1D70E}=\prod _{a}u_{a}^{s_{a}}\prod _{b}v_{b}^{r_{b}}$
, we set
![]() $\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}=\prod _{a}u_{a}^{s_{a}\unicode[STIX]{x1D701}_{a}}\prod _{b}v_{b}^{r_{b}\unicode[STIX]{x1D701}_{b}}$
.
$\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}=\prod _{a}u_{a}^{s_{a}\unicode[STIX]{x1D701}_{a}}\prod _{b}v_{b}^{r_{b}\unicode[STIX]{x1D701}_{b}}$
.
The following result is an extension of the result for unmixed bipartite graphs by Kummini [Reference Kummini10, Proposition 3.2] to very well-covered graphs.
Proposition 4.1. Let
![]() $G$
be a very well-covered graph. Using the above notation we have
$G$
be a very well-covered graph. Using the above notation we have
One can prove this proposition by a similar argument due to Kummini [Reference Kummini10]. We omit the proof.
Now we prove Theorem 1.1.
Proof of Theorem 1.1.
We use the same notation as above. Let
![]() $G$
be a very well-covered graph on
$G$
be a very well-covered graph on
![]() $V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) of Theorem 2.1.
$V=\{x_{1},\ldots ,x_{h},y_{1},\ldots ,y_{h}\}$
with the properties (i), (ii), (iii), (iv) of Theorem 2.1.
Take
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))\neq 0$
which gives
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))\neq 0$
which gives
![]() $\operatorname{pd}S/I(G)$
. We may assume that
$\operatorname{pd}S/I(G)$
. We may assume that
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
is extremal. Indeed, suppose that
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
is extremal. Indeed, suppose that
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
is not extremal. Then there exist
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
is not extremal. Then there exist
![]() $s\geqslant r$
and
$s\geqslant r$
and
![]() $\unicode[STIX]{x1D70F}\supsetneq \unicode[STIX]{x1D70E}$
with
$\unicode[STIX]{x1D70F}\supsetneq \unicode[STIX]{x1D70E}$
with
![]() $|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant s-r$
satisfying
$|\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|\geqslant s-r$
satisfying
![]() $\unicode[STIX]{x1D6FD}_{s,\unicode[STIX]{x1D70F}}(J(\widehat{G}))\neq 0$
. In this case,
$\unicode[STIX]{x1D6FD}_{s,\unicode[STIX]{x1D70F}}(J(\widehat{G}))\neq 0$
. In this case,
 $$\begin{eqnarray}\displaystyle (|\unicode[STIX]{x1D70F}^{\unicode[STIX]{x1D701}}|-s)-(|\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}|-r) & = & \displaystyle \mathop{\sum }_{u_{a}\in \unicode[STIX]{x1D70F}\setminus \unicode[STIX]{x1D70E}}\unicode[STIX]{x1D701}_{a}+\mathop{\sum }_{v_{b}\in \unicode[STIX]{x1D70F}\setminus \unicode[STIX]{x1D70E}}\unicode[STIX]{x1D701}_{b}-(s-r)\nonumber\\ \displaystyle & {\geqslant} & \displaystyle |\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|-(s-r)\geqslant 0.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle (|\unicode[STIX]{x1D70F}^{\unicode[STIX]{x1D701}}|-s)-(|\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}|-r) & = & \displaystyle \mathop{\sum }_{u_{a}\in \unicode[STIX]{x1D70F}\setminus \unicode[STIX]{x1D70E}}\unicode[STIX]{x1D701}_{a}+\mathop{\sum }_{v_{b}\in \unicode[STIX]{x1D70F}\setminus \unicode[STIX]{x1D70E}}\unicode[STIX]{x1D701}_{b}-(s-r)\nonumber\\ \displaystyle & {\geqslant} & \displaystyle |\unicode[STIX]{x1D70F}|-|\unicode[STIX]{x1D70E}|-(s-r)\geqslant 0.\nonumber\end{eqnarray}$$
Therefore, we can replace
![]() $\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
by
$\unicode[STIX]{x1D6FD}_{r,\unicode[STIX]{x1D70E}}(J(\widehat{G}))$
by
![]() $\unicode[STIX]{x1D6FD}_{s,\unicode[STIX]{x1D70F}}(J(\widehat{G}))$
.
$\unicode[STIX]{x1D6FD}_{s,\unicode[STIX]{x1D70F}}(J(\widehat{G}))$
.
Since
![]() $\widehat{G}$
is Cohen–Macaulay, we can take a pairwise
$\widehat{G}$
is Cohen–Macaulay, we can take a pairwise
![]() $3$
-disjoint set
$3$
-disjoint set
![]() $\widehat{{\mathcal{B}}}=\{\widehat{B}_{1},\ldots ,\widehat{B}_{r}\}$
of complete bipartite subgraphs of
$\widehat{{\mathcal{B}}}=\{\widehat{B}_{1},\ldots ,\widehat{B}_{r}\}$
of complete bipartite subgraphs of
![]() $\widehat{G}$
with
$\widehat{G}$
with
![]() $V(\widehat{{\mathcal{B}}})=\unicode[STIX]{x1D70E}$
as constructed in Proposition 3.5. We can assume that
$V(\widehat{{\mathcal{B}}})=\unicode[STIX]{x1D70E}$
as constructed in Proposition 3.5. We can assume that
![]() $\{u_{a_{k}},v_{a_{k}}\}\in E(\widehat{B}_{k})$
and
$\{u_{a_{k}},v_{a_{k}}\}\in E(\widehat{B}_{k})$
and
![]() $\{u_{a_{1}},v_{a_{1}}\},\ldots ,\{u_{a_{r}},v_{a_{r}}\}$
are pairwise
$\{u_{a_{1}},v_{a_{1}}\},\ldots ,\{u_{a_{r}},v_{a_{r}}\}$
are pairwise
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $\widehat{G}$
. Moreover, we can assume that
$\widehat{G}$
. Moreover, we can assume that
![]() $\widehat{B}_{k}$
is the complete bipartite subgraph of
$\widehat{B}_{k}$
is the complete bipartite subgraph of
![]() $\widehat{G}$
on
$\widehat{G}$
on
![]() $\widehat{V}_{k}:=V(\widehat{B}_{k})$
which is decomposed as
$\widehat{V}_{k}:=V(\widehat{B}_{k})$
which is decomposed as
![]() $\widehat{V}_{1k}\sqcup \widehat{V}_{2k}$
, where
$\widehat{V}_{1k}\sqcup \widehat{V}_{2k}$
, where
Set
 $$\begin{eqnarray}\displaystyle & \displaystyle V_{1k}:=\left(\mathop{\bigcup }_{u_{b}\in \widehat{V}_{1k}}\{x_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}\right)\cup \left(\mathop{\bigcup }_{v_{b}\in \widehat{V}_{1k}}\{y_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}\right), & \displaystyle \nonumber\\ \displaystyle & \displaystyle V_{2k}:=\mathop{\bigcup }_{u_{b}\in \widehat{V}_{2k}}\{x_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle V_{1k}:=\left(\mathop{\bigcup }_{u_{b}\in \widehat{V}_{1k}}\{x_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}\right)\cup \left(\mathop{\bigcup }_{v_{b}\in \widehat{V}_{1k}}\{y_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}\right), & \displaystyle \nonumber\\ \displaystyle & \displaystyle V_{2k}:=\mathop{\bigcup }_{u_{b}\in \widehat{V}_{2k}}\{x_{\ell }:p_{\ell }\in {\mathcal{Z}}_{b}\}. & \displaystyle \nonumber\end{eqnarray}$$
Since
![]() $\widehat{V}_{1k}\cap \widehat{V}_{2k}=\emptyset$
, we have
$\widehat{V}_{1k}\cap \widehat{V}_{2k}=\emptyset$
, we have
![]() $V_{1k}\cap V_{2k}=\emptyset$
. Note that for each
$V_{1k}\cap V_{2k}=\emptyset$
. Note that for each
![]() $b$
, at least one of
$b$
, at least one of
![]() $u_{b}$
and
$u_{b}$
and
![]() $v_{b}$
is not contained in
$v_{b}$
is not contained in
![]() $\widehat{V}_{1k}$
. Let
$\widehat{V}_{1k}$
. Let
![]() $B_{k}$
be the complete bipartite graph with the bipartition
$B_{k}$
be the complete bipartite graph with the bipartition
![]() $V_{1k}\sqcup V_{2k}$
. Set
$V_{1k}\sqcup V_{2k}$
. Set
![]() ${\mathcal{B}}:=\{B_{1},\ldots ,B_{r}\}$
. It is clear that
${\mathcal{B}}:=\{B_{1},\ldots ,B_{r}\}$
. It is clear that
![]() $V(B_{k})\cap V(B_{\ell })=\emptyset$
for
$V(B_{k})\cap V(B_{\ell })=\emptyset$
for
![]() $k\neq \ell$
and
$k\neq \ell$
and
![]() $\#V({\mathcal{B}})=|\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}|$
. We prove the following
$\#V({\mathcal{B}})=|\unicode[STIX]{x1D70E}^{\unicode[STIX]{x1D701}}|$
. We prove the following
![]() $2$
claims which derive the theorem.
$2$
claims which derive the theorem.
Claim 1.
![]() $B_{k}$
is a subgraph of
$B_{k}$
is a subgraph of
![]() $G$
.
$G$
.
Claim 2.
![]() ${\mathcal{B}}$
is pairwise
${\mathcal{B}}$
is pairwise
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $G$
.
$G$
.
Proof of Claim 1. Take
![]() $\{z_{\ell },x_{m}\}\in E(B_{k})$
, where
$\{z_{\ell },x_{m}\}\in E(B_{k})$
, where
![]() $z_{\ell }\in V_{1k}$
and
$z_{\ell }\in V_{1k}$
and
![]() $x_{m}\in V_{2k}$
. Then there exist
$x_{m}\in V_{2k}$
. Then there exist
![]() $w_{a}\in \widehat{V}_{1k}$
and
$w_{a}\in \widehat{V}_{1k}$
and
![]() $u_{b}\in \widehat{V}_{2k}$
such that
$u_{b}\in \widehat{V}_{2k}$
such that
![]() $p_{\ell }\in {\mathcal{Z}}_{a}$
and
$p_{\ell }\in {\mathcal{Z}}_{a}$
and
![]() $p_{m}\in {\mathcal{Z}}_{b}$
.
$p_{m}\in {\mathcal{Z}}_{b}$
.
We first assume
![]() $a=b$
. Since
$a=b$
. Since
![]() $w_{a}\in \widehat{V}_{1k}$
,
$w_{a}\in \widehat{V}_{1k}$
,
![]() $u_{a}\in \widehat{V}_{2k}$
, and
$u_{a}\in \widehat{V}_{2k}$
, and
![]() $\widehat{V}_{1k}\cap \widehat{V}_{2k}=\emptyset$
, it follows that
$\widehat{V}_{1k}\cap \widehat{V}_{2k}=\emptyset$
, it follows that
![]() $w_{a}=v_{a}$
and thus
$w_{a}=v_{a}$
and thus
![]() $z_{\ell }=y_{\ell }$
. On the other hand,
$z_{\ell }=y_{\ell }$
. On the other hand,
![]() $p_{\ell },p_{m}\in {\mathcal{Z}}_{a}$
. In particular,
$p_{\ell },p_{m}\in {\mathcal{Z}}_{a}$
. In particular,
![]() $p_{m}p_{\ell }\in E_{d}(\mathfrak{d}_{G})$
. This means
$p_{m}p_{\ell }\in E_{d}(\mathfrak{d}_{G})$
. This means
![]() $\{x_{m},y_{\ell }\}\in E(G)$
as desired.
$\{x_{m},y_{\ell }\}\in E(G)$
as desired.
We next assume
![]() $a\neq b$
. If
$a\neq b$
. If
![]() $z_{\ell }=x_{\ell }$
, then
$z_{\ell }=x_{\ell }$
, then
![]() $w_{a}=u_{a}$
and we have
$w_{a}=u_{a}$
and we have
![]() $\{q_{a},q_{b}\}\in E_{u}(\widehat{\mathfrak{d}}_{G})$
since
$\{q_{a},q_{b}\}\in E_{u}(\widehat{\mathfrak{d}}_{G})$
since
![]() $\{u_{a},u_{b}\}\in E(\widehat{B}_{k})\subset E(\widehat{G})$
. Therefore,
$\{u_{a},u_{b}\}\in E(\widehat{B}_{k})\subset E(\widehat{G})$
. Therefore,
![]() $\{p_{\ell },p_{m}\}\in E_{u}(\mathfrak{d}_{G})$
. This means that
$\{p_{\ell },p_{m}\}\in E_{u}(\mathfrak{d}_{G})$
. This means that
![]() $\{z_{\ell },x_{m}\}=\{x_{\ell },x_{m}\}\in E(G)$
. If
$\{z_{\ell },x_{m}\}=\{x_{\ell },x_{m}\}\in E(G)$
. If
![]() $z_{\ell }=y_{\ell }$
, then
$z_{\ell }=y_{\ell }$
, then
![]() $w_{a}=v_{a}$
and we have
$w_{a}=v_{a}$
and we have
![]() $q_{b}q_{a}\in E_{d}(\widehat{\mathfrak{d}}_{G})$
since
$q_{b}q_{a}\in E_{d}(\widehat{\mathfrak{d}}_{G})$
since
![]() $\{v_{a},u_{b}\}\in E(\widehat{B}_{k})\subset E(\widehat{G})$
. Therefore,
$\{v_{a},u_{b}\}\in E(\widehat{B}_{k})\subset E(\widehat{G})$
. Therefore,
![]() $p_{m}p_{\ell }\in E_{d}(\mathfrak{d}_{G})$
. This means that
$p_{m}p_{\ell }\in E_{d}(\mathfrak{d}_{G})$
. This means that
![]() $\{z_{\ell },x_{m}\}=\{y_{\ell },x_{m}\}\in E(G)$
.
$\{z_{\ell },x_{m}\}=\{y_{\ell },x_{m}\}\in E(G)$
.
Proof of Claim 2. Recall that
![]() $\{u_{a_{1}},v_{a_{1}}\},\ldots ,\{u_{a_{r}},v_{a_{r}}\}$
are pairwise
$\{u_{a_{1}},v_{a_{1}}\},\ldots ,\{u_{a_{r}},v_{a_{r}}\}$
are pairwise
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $\widehat{G}$
. Let
$\widehat{G}$
. Let
![]() $p_{\ell _{s}}\in {\mathcal{Z}}_{a_{s}}$
. Then
$p_{\ell _{s}}\in {\mathcal{Z}}_{a_{s}}$
. Then
![]() $\{x_{\ell _{1}},y_{\ell _{1}}\},\ldots ,\{x_{\ell _{r}},y_{\ell _{r}}\}$
are pairwise
$\{x_{\ell _{1}},y_{\ell _{1}}\},\ldots ,\{x_{\ell _{r}},y_{\ell _{r}}\}$
are pairwise
![]() $3$
-disjoint in
$3$
-disjoint in
![]() $G$
.◻
$G$
.◻
5 Projective dimension of symbolic powers of the edge ideal of a very well-covered graph
In this section we show that the projective dimension of symbolic powers of the edge ideal of a very well-covered graph is nondecreasing.
We recall the definition of a symbolic power of an ideal.
Let
![]() $I$
be a radical ideal of a polynomial ring
$I$
be a radical ideal of a polynomial ring
![]() $S$
. Let
$S$
. Let
![]() $\operatorname{Min}_{S}(S/I)=\{P_{1},\ldots ,P_{r}\}$
be the set of the minimal prime ideals of
$\operatorname{Min}_{S}(S/I)=\{P_{1},\ldots ,P_{r}\}$
be the set of the minimal prime ideals of
![]() $I$
, and put
$I$
, and put
![]() $W=S\setminus \bigcup _{i=1}^{r}P_{i}$
. Given an integer
$W=S\setminus \bigcup _{i=1}^{r}P_{i}$
. Given an integer
![]() $\ell \geqslant 1$
, the
$\ell \geqslant 1$
, the
![]() $\ell$
th symbolic power of
$\ell$
th symbolic power of
![]() $I$
is defined to be the ideal
$I$
is defined to be the ideal
In particular, if
![]() $I$
is a squarefree monomial ideal of
$I$
is a squarefree monomial ideal of
![]() $S$
, then one has
$S$
, then one has
We use the following lemma:
Lemma 5.1. [Reference Rauf14, Corollary 1.3]
Let
![]() $I$
be a monomial ideal in a polynomial ring
$I$
be a monomial ideal in a polynomial ring
![]() $S$
. Take a monomial
$S$
. Take a monomial
![]() $m$
such that
$m$
such that
![]() $m\not \in I$
. Then
$m\not \in I$
. Then
Now we state the main result in this section.
Theorem 5.2. Let
![]() $G$
be a very well-covered graph or a graph with a leaf. Then for
$G$
be a very well-covered graph or a graph with a leaf. Then for
![]() $i\geqslant 2$
,
$i\geqslant 2$
,
Proof. Let
![]() $P$
be a minimal prime ideal of
$P$
be a minimal prime ideal of
![]() $I(G)$
. We first assume that
$I(G)$
. We first assume that
![]() $G$
is a very well-covered graph whose vertices are labeled as in Theorem 2.1. Then
$G$
is a very well-covered graph whose vertices are labeled as in Theorem 2.1. Then
![]() $P$
is of the form
$P$
is of the form
where
![]() $z_{i}=x_{i}$
or
$z_{i}=x_{i}$
or
![]() $z_{i}=y_{i}$
for
$z_{i}=y_{i}$
for
![]() $i=1,2,\ldots ,h$
by [Reference Crupi, Rinaldo and Terai2, Corollary 2.2].
$i=1,2,\ldots ,h$
by [Reference Crupi, Rinaldo and Terai2, Corollary 2.2].
Next we assume that
![]() $G$
has a leaf
$G$
has a leaf
![]() $x_{1}$
. And we assume that
$x_{1}$
. And we assume that
![]() $\{x_{1},y_{1}\}\in E(G)$
. Then just either one of
$\{x_{1},y_{1}\}\in E(G)$
. Then just either one of
![]() $x_{1}$
and
$x_{1}$
and
![]() $y_{1}$
is contained in
$y_{1}$
is contained in
![]() $P$
.
$P$
.
In either case we show that
Take a minimal monomial generator
![]() $m$
of
$m$
of
![]() $P^{i}$
. Then
$P^{i}$
. Then
![]() $m$
is not divided by
$m$
is not divided by
![]() $x_{1}y_{1}$
and
$x_{1}y_{1}$
and
![]() $x_{1}y_{1}\in P$
. Hence
$x_{1}y_{1}\in P$
. Hence
![]() $(m):x_{1}y_{1}\subset P^{i-1}$
. Hence
$(m):x_{1}y_{1}\subset P^{i-1}$
. Hence
![]() $P^{i}:x_{1}y_{1}\subset P^{i-1}$
. Conversely, take a minimal monomial generator
$P^{i}:x_{1}y_{1}\subset P^{i-1}$
. Conversely, take a minimal monomial generator
![]() $m$
of
$m$
of
![]() $P^{i-1}$
. Since we have
$P^{i-1}$
. Since we have
![]() $x_{1}\in P$
or
$x_{1}\in P$
or
![]() $y_{1}\in P$
, we have
$y_{1}\in P$
, we have
![]() $mx_{1}y_{1}\in P^{i}$
. Then we have
$mx_{1}y_{1}\in P^{i}$
. Then we have
![]() $P^{i-1}\subset P^{i}:x_{1}y_{1}$
.
$P^{i-1}\subset P^{i}:x_{1}y_{1}$
.
Now we have
 $$\begin{eqnarray}\displaystyle I(G)^{(i)}:x_{1}y_{1} & = & \displaystyle \left(\mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}P^{i}\right):x_{1}y_{1}\nonumber\\ \displaystyle & = & \displaystyle \mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}(P^{i}:x_{1}y_{1})\nonumber\\ \displaystyle & = & \displaystyle \mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}P^{i-1}\nonumber\\ \displaystyle & = & \displaystyle I(G)^{(i-1)}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle I(G)^{(i)}:x_{1}y_{1} & = & \displaystyle \left(\mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}P^{i}\right):x_{1}y_{1}\nonumber\\ \displaystyle & = & \displaystyle \mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}(P^{i}:x_{1}y_{1})\nonumber\\ \displaystyle & = & \displaystyle \mathop{\bigcap }_{P\in \operatorname{Min}_{S}S/I(G)}P^{i-1}\nonumber\\ \displaystyle & = & \displaystyle I(G)^{(i-1)}.\nonumber\end{eqnarray}$$
By Lemma 5.1 we have
As an application we show that the projective dimension of the ordinary powers of the edge ideal is also nondecreasing for certain bipartite graphs.
Corollary 5.3. Let
![]() $G$
be an unmixed or sequentially Cohen–Macaulay bipartite graph. Then for
$G$
be an unmixed or sequentially Cohen–Macaulay bipartite graph. Then for
![]() $i\geqslant 2$
,
$i\geqslant 2$
,
The above corollary follows from the next facts:
Lemma 5.4. [Reference Rinaldo, Terai and Yoshida15, Lemma 3.10], [Reference Simis, Vasconcelos and Villarreal16, Lemma 5.8, Theorem 5.9]
Let
![]() $I(G)$
be the edge ideal of a graph
$I(G)$
be the edge ideal of a graph
![]() $G$
. Let
$G$
. Let
![]() $t\geqslant 2$
be an integer. Then
$t\geqslant 2$
be an integer. Then
![]() $I(G)^{(t)}=I(G)^{t}$
holds if and only if
$I(G)^{(t)}=I(G)^{t}$
holds if and only if
![]() $G$
contains no odd cycles of length
$G$
contains no odd cycles of length
![]() $2s-1$
for any
$2s-1$
for any
![]() $2\leqslant s\leqslant t$
.
$2\leqslant s\leqslant t$
.
Lemma 5.5. [Reference Van Tuyl and Villarreal20, Lemma 2.8]
Let
![]() $G$
be a sequentially Cohen–Macaulay bipartite graph. Then
$G$
be a sequentially Cohen–Macaulay bipartite graph. Then
![]() $G$
has a leaf.
$G$
has a leaf.
Remark 5.6. Using the corresponding result to Lemma 5.1 for Stanley depth
![]() $\operatorname{sdepth}$
instead of depth in [Reference Cimpoeaş1], we can prove the following nonincreasing property of Stanley depth, similarly:
$\operatorname{sdepth}$
instead of depth in [Reference Cimpoeaş1], we can prove the following nonincreasing property of Stanley depth, similarly:
(1)
 $\operatorname{sdepth}S/I(G)^{(i)}\leqslant \operatorname{sdepth}S/I(G)^{(i-1)}$
for a very well-covered graph
$\operatorname{sdepth}S/I(G)^{(i)}\leqslant \operatorname{sdepth}S/I(G)^{(i-1)}$
for a very well-covered graph
 $G$
with
$G$
with
 $i\geqslant 2$
.
$i\geqslant 2$
.(2)
 $\operatorname{sdepth}S/I(G)^{i}\leqslant \operatorname{sdepth}S/I(G)^{i-1}$
for an unmixed or sequentially Cohen–Macaulay bipartite graph
$\operatorname{sdepth}S/I(G)^{i}\leqslant \operatorname{sdepth}S/I(G)^{i-1}$
for an unmixed or sequentially Cohen–Macaulay bipartite graph
 $G$
with
$G$
with
 $i\geqslant 2$
.
$i\geqslant 2$
.
Acknowledgments
Kimura was partially supported by JSPS Grant-in-Aid for Young Scientists (B) 24740008/15K17507. Terai was partially supported by JSPS Grant-in-Aid (C) 26400049 and thanks the American Institute of Mathematics for giving the chance to participate in SQuaRE “Ordinary powers and symbolic powers.” Yassemi was partially supported by a grant from University of Tehran. The authors thank the referee for many comments.



