Hostname: page-component-5cf477f64f-2wr7h Total loading time: 0 Render date: 2025-04-06T01:23:58.495Z Has data issue: false hasContentIssue false
  • English
  • Français

On defect relations of moving hyperplanes

Published online by Cambridge University Press:  22 January 2016

Manabu Shirosaki
Manabu Shirosaki*
Affiliation:
Department of Mathematics, Faculty of Science, Kanazawa University, Marunouchi, Kanazawa 920, Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The defect relation 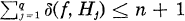gives the best-possible estimate, where f is a linearly non-degenerate holomorphic curve in Pn(C) and H1, …, Hq are hyperplanes in Pn(C) which are in general position. However, the case of moving hyperplanes has ever got only n(n + 1) instead of n + 1 (Stoll [4]) and it has not yet been known whether this bound is best-possible or not. In this paper we shall give some particular cases which have the bound n + 1.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 120 , December 1990 , pp. 103 - 112
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1990

References

[1] Griffiths, P. and King, J., Nevanlinna theory and holomorphic mappings between algebraic varieties, Acta Math., 130 (1973), 145–220.Google Scholar
[2] Mori, S., Remarks on holomorphic mappings, Contemporary Math. Vol. 25, 1983, 101–113.CrossRefGoogle Scholar
[3] V, B.. Shabat, Distribution of values of holomorphic mappings, Amer. Math. Soc., Providence, R.I., 1985.Google Scholar
[4] Stoll, W., An extension of the theorem of Steinmetz-Nevanlinna to holomorphic curves, Math. Ann., 282 (1988), 185–222.Google Scholar
[5] Stoll, W., Value distribution theory for meromorphic maps, Aspects of Mathematics, E7, Vieweg, 1985.Google Scholar