Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-24T16:33:29.512Z Has data issue: false hasContentIssue false
  • English
  • Français

Lp extension of holomorphic functions from submanifolds to strictly pseudoconvex domains with non-smooth boundary

Published online by Cambridge University Press:  22 January 2016

Kenzō Adachi
Kenzō Adachi*
Affiliation:
Department of Mathematics, Nagasaki University, Nagasaki, 852-8521, Japan, [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let D be a bounded strictly pseudoconvex domain in ℂn (with not necessarily smooth boundary) and let X be a submanifold in a neighborhood of 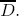. Then any Lp (1 ≥ p < ∞) holomorphic function in X ∩ D can be extended to an Lp holomorphic function in D.

Keywords

32A2632D15
Type
Research Article
Information
Nagoya Mathematical Journal , Volume 172 , 2003 , pp. 103 - 110
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 2003

References

[1] Beatrous, F., Lp estimates for extensions of holomorphic functions, Michigan Math. J., 32 (1985), 361–380.Google Scholar
[2] Cho, H. R., A counterexample to the Lp extension of holomorphic functions from subvarieties to pseudoconvex domains, Complex Variables, 35 (1998), 89–91.Google Scholar
[3] Cumenge, A., Extension dan des classes de Hardy de fonctions holomorphes et estimations de type “mesures de Carleson” pour l’equation ∂, Ann. Inst. Fourier, 33 (1983), 59–97.Google Scholar
[4] Henkin, G. M., Continuation of bounded holomorphic functions from submanifolds in general position in a strictly pseudoconvex domain, Math. USSR Izv., 6 (1972), 536–563.Google Scholar
[5] Henkin, G. M. and Leiterer, J., Theory of functions on complex manifolds, Birkhäuser, 1984.Google Scholar
[6] Ohsawa, T. and Takegoshi, K., On the extension of L2 holomorphic functions, Math. Z., 195 (1987), 197–204.Google Scholar
[7] Schmalz, G., Solution of the -equation with uniform estimates on strictly q-convex domains with non-smooth boundary, Math. Z., 202 (1989), 409–430.Google Scholar