Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-02T22:51:02.363Z Has data issue: false hasContentIssue false
  • English
  • Français

The law of the iterated logarithm on subsequences-characterizations

Published online by Cambridge University Press:  22 January 2016

Michel Weber
Michel Weber*
Affiliation:
Université Louis Pasteur, Uer de Mathématiques et Informatique, 7, rue René Descartes, 67084 Strasbourg Cedex, France
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

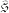Let be any increasing sequence of integers and M> 1; we connect to them in a very simply way, an increasing unbounded function φ: 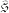→ R+. Let also X1, X2, · · · be a sequence of i.i.d. random vectors with value in euclidian space Rm. We prove that the cluster set of the sequence 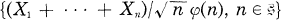almost surely coincides with the unit ball of Rm, if, and only if, the covariance matrix of X1 is the identity matrix of Rm and EX1 is the zero vector of Rm. We define a functional A on the set of increasing sequences of integers as follows:

.

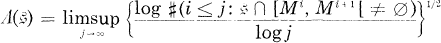Type
Research Article
Information
Nagoya Mathematical Journal , Volume 118 , June 1990 , pp. 65 - 97
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1990

References

[1] Borell, C., The Brunn-Minkowski inequality in Gauss space, Invent. Math., 30 (1975), 207–216.CrossRefGoogle Scholar
[2] Breiman, L., Probability. Addison Wesley, 1968.Google Scholar
[3] Chung, K. L., Erdos, P. and Sirao, T., On the Lipschitz’s conditions for Brownian motion, J. Math. Soc. Japan, 11 (1959), 263–274.CrossRefGoogle Scholar
[4] Fernique, X., Régularité des trajectoires des fonctions aléatoires gaussiennes, Ecole d’été de Probabilités de Saint-Flour 1974, Lecture Notes in Math., 480 (1975), 1–96. Springer, Berlin.Google Scholar
[5] Finkelstein, H., The law of the iterated logarithm for empirical distributions, Ann. of Math. Stat., 42 (1971), 607–615.CrossRefGoogle Scholar
[6] Gut, A., The law of the iterated on subsequences, Publ. Uppsala University, 1983.Google Scholar
[7] Hartman, P. and Wintner, A., On the law of the iterated logarithm, Amer. J. Math., 63 (1941), 169–176.CrossRefGoogle Scholar
[8] Hoffman-Jørgensen, J., Sums of independent Banach space valued random variables, Studia Math., 52 (1974), 159–186.CrossRefGoogle Scholar
[9] Kuelbs, J., The law of the iterated logarithm and related strong convergence theorems for Banach space valued random variables, Ecole d’été de Probabilité de Saint-Flour 1975. Lecture Notes in Math., 539 (1976), 224–314. Springer, Berlin.Google Scholar
[10] Ledoux, M. and Talagrand, M., Characterization of the law of the iterated logarithm in Banach spaces, Ann. of Prob., 16, (1988), 1242–1264.CrossRefGoogle Scholar
[11] Strassen, V., An invariance principle for the law of the iterated logarithm, Z. Wahrsch. verw. Geb., 3 (1964), 211–226.CrossRefGoogle Scholar
[12] Strassen, V., A converse to the law of the iterated logarithm, Z. Wahrsch. verw. Geb., 4 (1966), 265–268.CrossRefGoogle Scholar
[13] Talagrand, M., Regularity of gaussian processes, Acta Math., 159 (1987), 99–149.CrossRefGoogle Scholar
[14] Torrang, I., The law of the iterated logarithm — cluster points of deterministic and random subsequences, Prob. Math. Stat., 8 (1987), 133–141.Google Scholar
[15] Vaughan, R. C., The Hardy-Littlewood method, Cambridge University Press, 1981.Google Scholar
[16] Weber, M., La loi du logarithme itéré sur les sous-suites, Comptes Rendus Acad. Sci. Paris, 303 Sér. 1 (1986), 77–80.Google Scholar
[17] Weber, M., La loi du logarithme itéré sur toute sous-suite, caractérisations, C.R. Acad. Paris, 305 (1987), 835–840.Google Scholar
[18] Weber, M., The law of the iterated logarithm for subsequences in Banach spaces, Prob. in Banach spaces VII, (1988), to appear in Birkhäuser ed., Progress in Prob. and Stat.Google Scholar
[19] Yurinskii, V. V., Exponential bound for large deviations, Theor. Prob., Appl., 19 (1974),154–155.CrossRefGoogle Scholar