Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T05:50:25.427Z Has data issue: false hasContentIssue false
  • English
  • Français

L2 Extension for jets of holomorphic sections of a Hermitian line Bundle

Published online by Cambridge University Press:  11 January 2016

Dan Popovici
Dan Popovici*
Affiliation:
Mathematics Institute University of Warwick CoventryCV4 7ALUnited [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let (X, ω) be a weakly pseudoconvex Kähler manifold, Y ⊂ X a closed submanifold defined by some holomorphic section of a vector bundle over X, and L a Hermitian line bundle satisfying certain positivity conditions. We prove that for any integer k > 0, any section of the jet sheaf 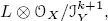which satisfies a certain L2 condition, can be extended into a global holomorphic section of L over X whose L2 growth on an arbitrary compact subset of X is under control. In particular, if Y is merely a point, this gives the existence of a global holomorphic function with an L2 norm under control and with prescribed values for all its derivatives up to order k at that point. This result generalizes the L2 extension theorems of Ohsawa-Takegoshi and of Manivel to the case of jets of sections of a line bundle. A technical difficulty is to achieve uniformity in the constant appearing in the final estimate. To this end, we make use of the exponential map and of a Rauch-type comparison theorem for complete Riemannian manifolds.

Keywords

53C5553C0732A1032U05
Type
Research Article
Information
Nagoya Mathematical Journal , Volume 180 , 2005 , pp. 1 - 34
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 2005

References

[AS95] Angehrn, U. and Siu, Y. T., Effective freeness and point separation for adjoint bundles, Invent. Math., 122 (1995), no. 2, 291–308.CrossRefGoogle Scholar
[Agm65] Agmon, S., Lectures on Elliptic Boundary Value Problems, Van Nostrand, Princeton, 1965.Google Scholar
[BC64] Bishop, R. and Crittenden, R. J., Geometry of Manifolds, Academic Press, 1964.Google Scholar
[Dem82] Demailly, J.-P., Estimations L2 pour l’opérateur ∂ d’un fibré vectoriel holomor-phe semi-positif au-dessus d’une variété kählérienne complète, Ann. Sci. École Norm. Sup., 15 (1982), 457–511.CrossRefGoogle Scholar
[Dem92] Demailly, J.-P., Regularization of Closed Positive Currents and Intersection Theory, J. Alg. Geom., 1 (1992), 361–409.Google Scholar
[Dem96] Demailly, J.-P., Effective Bounds for Very Ample Line Bundles, Invent. Math., 124 (1996), 243–261.CrossRefGoogle Scholar
[Dem00] Demailly, J.-P., On the Ohsawa-Takegoshi-Manivel L2 Extension Theorem, Article en l’honneur de Pierre Lelong à l’occasion de son 85ème anniversaire, Complex Analysis and Geometry (Paris, 1997), Progr. Math., 188, Birkh¨auser, Basel (2000), pp. 47–82.CrossRefGoogle Scholar
[DEL00] Demailly, J.-P., Ein, L. and Lazarsfeld, R., A Subadditivity Property of Multiplier Ideals, Michigan Math. J., 48 (2000), 137–156.CrossRefGoogle Scholar
[DK01] Demailly, J.-P. and Kollär, J., Semicontinuity of Complex Singularity Exponents and Kähler-Einstein Metrics on Fano Orbifolds, Ann. Scient. Ecole Norm. Sup. (4), 34 (2001), 525–556.CrossRefGoogle Scholar
[DM00] Diederich, K. and Mazzilli, E., A Remark on the Theorem of Ohsawa-Takegoshi, Nagoya Math. J., 158 (2000), 185–189.CrossRefGoogle Scholar
[HL84] Henkin, G. and Leiterer, J., Theory of Functions on Complex Manifolds, Birkhäuser Verlag, Basel, Boston, Stuttgart, 1984.Google Scholar
[Hör65] Hörmander, L., L2 Estimates and Existence Theorems for the ∂ Operator, Acta Math., 113 (1965), 89–152.CrossRefGoogle Scholar
[Hör66] Hörmander, L., An Introduction to Complex Analysis in Several Variables, 1st edition, Elsevier Science Pub., New York, 1966, 3rd revised edition, North-Holland math. library, Vol. 7, Amsterdam, 1990.Google Scholar
[Kol97] Kolláar, J., Singularities of Pairs, Algebraic Geometry, Santa Cruz 1995, Proc. Sympos. Pure Math., 62, Part 1, Amer. Math. Soc., Providence, RI (1997), pp. 221–287.Google Scholar
[Man93] Manivel, L., Un théorème de prolongement L2 de sections holomorphes d’un fibré hermitien, Math. Zeitschrift, 212 (1993), 107–122.CrossRefGoogle Scholar
[OT87] Ohsawa, T. and Takegoshi, K., On The Extension of L2 Holomorphic Functions, Math. Zeitschrift, 195 (1987), 197–204.CrossRefGoogle Scholar
[Ohs88] Ohsawa, T., On the Extension of L2 Holomorphic Functions, II, Publ. RIMS, Kyoto Univ., 24 (1988), 265–275.CrossRefGoogle Scholar
[Ohs94] Ohsawa, T., On the Extension of L2 Holomorphic Functions, IV: A New Density Conept, Geometry and Analysis on Complex Manifolds (Mabuchi, T. et al., eds.), Festschrift for Professor S. Kobayashi’s 60th birthday, Singapore:World Scientific (1994), pp. 157–170.CrossRefGoogle Scholar
[Ohs95] Ohsawa, T., On the Extension of L2 Holomorphic Functions, III: Negligible Weights, Math. Zeitschrift, 219 (1995), 215–225.CrossRefGoogle Scholar
[Ohs02] Ohsawa, T., A Precise L2 Division Theorem, Complex Geometry (Göttingen, 2000), Springer, Berlin (2002), pp. 185–191.Google Scholar
[Ohs04] Ohsawa, T., Generalization of a Precise L2 Division Theorem, Complex Analysis in Several Variables, Memorial Conference of Kiyoshi Oka’s Centennial Birthday, Adv. Stud. Pure Math., 42, Math. Soc. Japan, Tokyo (2004), pp. 249–261.Google Scholar
[Siu93] Siu, Y. T., An Effective Matsusaka Big Theorem, Ann. Inst. Fourier, 43 (1993), 1387–1405.CrossRefGoogle Scholar
[Siu98] Siu, Y. T., Invariance of Plurigenera, Invent. Math., 134 (1998), 661–673.CrossRefGoogle Scholar
[Siu02] Siu, Y. T., Extension of Twisted Pluricanonical Sections with Plurisubharmonic Weight and Invariance of Semipositively Twisted Plurigenera for Manifolds Not Necessarily of General Type, Complex Geometry (Göttingen, 2000), Springer, Berlin (2002), pp. 223–277.Google Scholar
[Sko78] Skoda, H., Morphismes surjectifs de fibrés vectoriels semi-positifs, Ann. Sci. École Norm. Sup. (4), 11 (1978), no. 4, 577–611.CrossRefGoogle Scholar