Hostname: page-component-f554764f5-wjqwx Total loading time: 0 Render date: 2025-04-10T22:38:33.702Z Has data issue: false hasContentIssue false
  • English
  • Français

A Generalization of Suspension Theorems

Published online by Cambridge University Press:  22 January 2016

Yasutoshi Nomura
Yasutoshi Nomura*
Affiliation:
Department of Mathematics, Shizuoha University, Shizuoka, Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Our purpose in this note is to establish a classification theorem for fiberings with a loop-space as fibre. This is deduced by applying a generalized suspension theorem which will be proved in § 1. As a by-product we obtain a proposition concerning fiberings with a loop-space as the total. Throughout this note we shall denote by 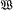the category of spaces having the based homotopy type of a CW-complex.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 19 , October 1961 , pp. 159 - 167
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1961

References

[1] Berstein, I. and Hilton, P. J., Category and generalized Hopf invariants, Illinois J. of Math. 4 (1960), 437–451.CrossRefGoogle Scholar
[2] Blakers, A. L. and Massey, W. S., The homotopy groups of a triad I, Ann. of Math. 33 (1951), 161–205.CrossRefGoogle Scholar
[3] Ganea, T., Fibrations and Cocategory, Comment. Math. Helv. 35 (1961), 15–24.CrossRefGoogle Scholar
[4] Milnor, J., The geometric realization of a semi-simplicial complex, Ann. of Math. 65 (1957), 357–362.CrossRefGoogle Scholar
[5] Milnor, J., On spaces having the homotopy type of a CW-complex , Trans. Amer. Math. Soc. 90 (1957), 272–280.Google Scholar
[6] Nomura, Y., On mapping sequences, Nagoya Math. J. 17 (1960), 111–145.Google Scholar
[7] Stasheff, J., On the space-of-loops isomorphism, Proc. Amer. Math. Soc. 10 (1959), 987–993.Google Scholar
[8] Whitehead, J. H. C., Combinatorial homotopy I, Bull. Amer. Math. Soc. 55 (1949), 245–277.Google Scholar