Hostname: page-component-745bb68f8f-d8cs5 Total loading time: 0 Render date: 2025-02-11T17:37:10.269Z Has data issue: false hasContentIssue false
  • English
  • Français

ENRIQUES INVOLUTIONS AND BRAUER CLASSES

Part of: Surfaces and higher-dimensional varieties (Co)homology theory

Published online by Cambridge University Press:  13 December 2022

A. N. SKOROBOGATOV Open the ORCID record for A. N. SKOROBOGATOV [Opens in a new window]  and
D. VALLONI Open the ORCID record for D. VALLONI [Opens in a new window]
A. N. SKOROBOGATOV*
Affiliation:
Department of Mathematics South Kensington Campus Imperial College London SW7 2BZ London United Kingdom - and - Institute for the Information Transmission Problems Russian Academy of Sciences 19 Bolshoi Karetnyi Moscow 127994 Russia
D. VALLONI
Affiliation:
Leibniz Universität Hannover Riemann Center for Geometry and Physics Welfengarten 1 30167 Hannover Germany [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that every element of order 2 in the Brauer group of a complex Kummer surface X descends to an Enriques quotient of X. In generic cases, this gives a bijection between the set ${\mathcal Enr}(X)$ of Enriques quotients of X up to isomorphism and the set of Brauer classes of X of order 2. For some K3 surfaces of Picard rank 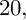$20,$ we prove that the fibers of 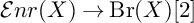${\mathcal Enr}(X)\to \mathrm {{Br}}(X)[2]$ above the nonzero points have the same cardinality.

MSC classification

Primary: 14J28: $K3$ surfaces and Enriques surfaces 14F22: Brauer groups of schemes
Type
Article
Information
Nagoya Mathematical Journal , Volume 251 , September 2023 , pp. 606 - 621
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

D.V. was funded by the European Research Council under EU Horizon 2020 research and innovation program grant agreement no. 948066.

References

Balestrieri, F., Berg, J., Manes, M., Park, J., and Viray, B., “ Insufficiency of the Brauer – Manin obstruction for Enriques surfaces ” in Eischen, E.E., Long, L., Pries, R., and Stange, K. (eds.), Directions in number theory, Women Math. Series 3, Springer, Cham, 2016, 1–31.Google Scholar
Beauville, A., On the Brauer group of Enriques surfaces , Math. Res. Lett. 16 (2009), 927–934.CrossRefGoogle Scholar
Brandhorst, S., Sonel, S., and Veniani, D.C., Idoneal genera and K3 surfaces covering an Enriques surface, preprint, arXiv:2003.08914 Google Scholar
Colliot-Thélène, J.-L. and Skorobogatov, A.N., The Brauer–Grothendieck Group, Ergeb. Math. Grenzgeb. (3) 71, Cham: Springer, 2021.CrossRefGoogle Scholar
Garbagnati, A. and Schütt, M., Enriques surfaces: Brauer groups and Kummer structures , Michigan Math. J. 61 (2012) 297–330.CrossRefGoogle Scholar
Harari, D. and Skorobogatov, A.N., Non-abelian descent and the arithmetic of Enriques surfaces , Int. Math. Res. Notices 52 (2005), 3203–3228.CrossRefGoogle Scholar
Horikawa, E., On the periods of Enriques surfaces. I, II , Math. Ann. 234 (1978), 217–246.CrossRefGoogle Scholar
Hulek, K. and Schütt, M., Enriques surfaces and Jacobian elliptic K3 surfaces , Math. Z. 268 (2011), 1025–1056.CrossRefGoogle Scholar
Huybrechts, D., Lectures on K3 Surfaces, Cambridge Stud. Adv. Math. 158, Cambridge: Cambridge University Press, 2016.10.1017/CBO9781316594193CrossRefGoogle Scholar
Keum, J.H., Every algebraic Kummer surface is the K3-cover of an Enriques surface , Nagoya Math. J. 118 (1990), 99–110.CrossRefGoogle Scholar
Nikulin, V.V., Integer symmetric bilinear forms and some of their geometric applications , Izv. Akad. Nauk SSSR Ser. Mat. 43 (1979), 111–177 (in Russian). English translation: Math. USSR-Izv. 14 (1980), 103–167.Google Scholar
Ohashi, H., On the number of Enriques quotients of a K3 surface , Publ. Res. Inst. Math. Sci. 43 (2007), 181–200.10.2977/prims/1199403814CrossRefGoogle Scholar
Ohashi, H., Enriques surfaces covered by Jacobian Kummer surfaces , Nagoya Math. J. 195 (2009), 165–186.CrossRefGoogle Scholar
Sertöz, A.S., Which singular K3 surfaces cover an Enriques surface , Proc. Amer. Math. Soc. 133 (2005), 43–50.CrossRefGoogle Scholar
Shimada, I. and Veniani, D.C., Enriques involutions on singular K3 surfaces of small discriminants , Ann. Sc. Norm. Super. Pisa Cl. Sci. 21 (2020), 1667–1701.Google Scholar
Valloni, D., Complex multiplication and Brauer groups of K3 surfaces , Adv. Math. 385 (2021), art. ID 107772.10.1016/j.aim.2021.107772CrossRefGoogle Scholar
Várilly-Alvarado, A. and Viray, B., Failure of the Hasse principle for Enriques surfaces , Adv. Math. 226 (2011), 4884–4901.CrossRefGoogle Scholar