Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Pouzancre, Agnès
1998.
Résidus d'ordre supérieur pour une famille d'actions sur un fibre vectoriel holomorphe.
Comptes Rendus de l'Académie des Sciences - Series I - Mathematics,
Vol. 327,
Issue. 12,
p.
969.
LEHMANN, DANIEL
and
SUWA, TATSUO
1999.
GENERALIZATION OF VARIATIONS AND BAUM–BOTT RESIDUES FOR HOLOMORPHIC FOLIATIONS ON SINGULAR VARIETIES.
International Journal of Mathematics,
Vol. 10,
Issue. 03,
p.
367.


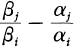 soient également non nulles pour
soient également non nulles pour 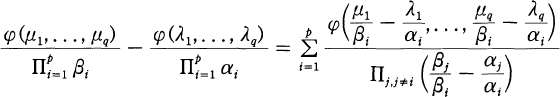 .
.