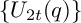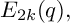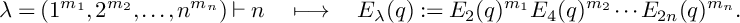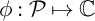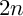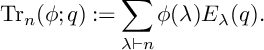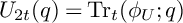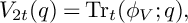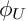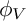1 Introduction and statement of results
In his “lost notebook”, Ramanujan considered the [Reference Ramanujan12, p. 369] two sequences of q-series:
 $$ \begin{align} U_{2t}(q)=\frac{1^{2t+1}-3^{2t+1}q+5^{2t+1}q^3-7^{2t+1}q^6+\cdots} {1-3q+5q^3-7q^6+\cdots} =\frac{\sum_{n\geq0}(-1)^n(2n+1)^{2t+1}q^{\frac{n(n+1)}2}}{\sum_{n\geq0}(-1)^n(2n+1)q^{\frac{n(n+1)}2}}, \end{align} $$
$$ \begin{align} U_{2t}(q)=\frac{1^{2t+1}-3^{2t+1}q+5^{2t+1}q^3-7^{2t+1}q^6+\cdots} {1-3q+5q^3-7q^6+\cdots} =\frac{\sum_{n\geq0}(-1)^n(2n+1)^{2t+1}q^{\frac{n(n+1)}2}}{\sum_{n\geq0}(-1)^n(2n+1)q^{\frac{n(n+1)}2}}, \end{align} $$
 $$ \begin{align} V_{2t}(q)=\frac{1^{2t}-5^{2t}q-7^{2t}q^2+11^{2t}q^5+\cdots} {1-q-q^2+q^5+\cdots} =\frac{\sum_{n=-\infty}^{\infty}(-1)^n(6n+1)^{2t}q^{\frac{n(3n+1)}2}}{\sum_{n=-\infty}^{\infty}(-1)^n q^{\frac{n(3n+1)}2}}, \end{align} $$
$$ \begin{align} V_{2t}(q)=\frac{1^{2t}-5^{2t}q-7^{2t}q^2+11^{2t}q^5+\cdots} {1-q-q^2+q^5+\cdots} =\frac{\sum_{n=-\infty}^{\infty}(-1)^n(6n+1)^{2t}q^{\frac{n(3n+1)}2}}{\sum_{n=-\infty}^{\infty}(-1)^n q^{\frac{n(3n+1)}2}}, \end{align} $$
and he offered identities such as
 $$ \begin{align*} &U_0=1,\ \ U_2=E_2,\ \ U_4=\frac13(5E_2^2-2E_4),\ \ U_6=\frac19(35E_2^3-42E_2E_4+16E_6),\dots\\ &V_0=1,\ \ V_2=E_2,\ \ V_4=3E_2^2-2E_4,\ \qquad V_6=15E_2^3-30E_2E_4+16E_6,\dots \end{align*} $$
$$ \begin{align*} &U_0=1,\ \ U_2=E_2,\ \ U_4=\frac13(5E_2^2-2E_4),\ \ U_6=\frac19(35E_2^3-42E_2E_4+16E_6),\dots\\ &V_0=1,\ \ V_2=E_2,\ \ V_4=3E_2^2-2E_4,\ \qquad V_6=15E_2^3-30E_2E_4+16E_6,\dots \end{align*} $$
where
![]() $E_2(q), E_4(q),$
and
$E_2(q), E_4(q),$
and
![]() $E_6(q)$
are the usual Eisenstein series
$E_6(q)$
are the usual Eisenstein series
 $$ \begin{align*} \begin{aligned} E_2:=1-24\sum_{n=1}^{\infty} \sigma_1(n)q^n,\ \ E_4:=1+240\sum_{n=1}^{\infty}\sigma_3(n)q^n, \ \ \text{and}\ \ E_6:=1-504\sum_{n=1}^{\infty} \sigma_5(n)q^n, \end{aligned} \end{align*} $$
$$ \begin{align*} \begin{aligned} E_2:=1-24\sum_{n=1}^{\infty} \sigma_1(n)q^n,\ \ E_4:=1+240\sum_{n=1}^{\infty}\sigma_3(n)q^n, \ \ \text{and}\ \ E_6:=1-504\sum_{n=1}^{\infty} \sigma_5(n)q^n, \end{aligned} \end{align*} $$
and where
![]() $\sigma _v(n):=\sum _{d\mid n}d^v.$
He made the following claim:
$\sigma _v(n):=\sum _{d\mid n}d^v.$
He made the following claim:
Berndt, Chan, Liu, Yee, and Yesilyurt [Reference Berndt, Chan, Liu and Yesilyurt5], [Reference Berndt and Yee6] proved this claim using Ramanujan’s identities [Reference Ramanujan13]
 $$ \begin{align} D(E_2) =\frac{E_2^2-E_4}{12},\ \ \ D(E_4)=\frac{E_2E_4-E_6}{3},\ \ \ \mathrm{and}\ \ \ D(E_6)=\frac{E_2E_6-E_4^2}{2}, \end{align} $$
$$ \begin{align} D(E_2) =\frac{E_2^2-E_4}{12},\ \ \ D(E_4)=\frac{E_2E_4-E_6}{3},\ \ \ \mathrm{and}\ \ \ D(E_6)=\frac{E_2E_6-E_4^2}{2}, \end{align} $$
where
![]() $D:=q\frac {d}{dq}.$
However, their results are not explicit. Indeed, Andrews and Berndt (see p. 364 of [Reference Andrews and Berndt3]) proclaim that “…it seems extremely difficult to find a general formula for all
$D:=q\frac {d}{dq}.$
However, their results are not explicit. Indeed, Andrews and Berndt (see p. 364 of [Reference Andrews and Berndt3]) proclaim that “…it seems extremely difficult to find a general formula for all
![]() $K_{\ell ,m,n}.$
”
$K_{\ell ,m,n}.$
”
We offer a solution to the general problem of obtaining the first explicit formulas for
![]() $U_{2t}$
and
$U_{2t}$
and
![]() $V_{2t}.$
We note that Ramanujan’s claim is that
$V_{2t}.$
We note that Ramanujan’s claim is that
![]() $U_{2t}$
and
$U_{2t}$
and
![]() $V_{2t}$
are weight
$V_{2t}$
are weight
![]() $2t$
quasimodular forms, as the ring of quasimodular forms is the polynomial ring (for example, see [Reference Kaneko and Zagier10])
$2t$
quasimodular forms, as the ring of quasimodular forms is the polynomial ring (for example, see [Reference Kaneko and Zagier10])
and so our goal is to obtain explicit formulas in terms of the classical sequence of Eisenstein series (for example, see Chapter 1 of [Reference Ono11] or Thm. 12.17 of [Reference Apostol4])
 $$ \begin{align} E_{2k}(q):=1-\frac{4k}{B_{2k}}\sum_{n=1}^{\infty}\sigma_{2k-1}(n)q^n, \end{align} $$
$$ \begin{align} E_{2k}(q):=1-\frac{4k}{B_{2k}}\sum_{n=1}^{\infty}\sigma_{2k-1}(n)q^n, \end{align} $$
where
![]() $B_{2k}$
is the
$B_{2k}$
is the
![]() $2k$
th Bernoulli number. We express Ramanujan’s q-series as explicit “traces of partition Eisenstein series.”
$2k$
th Bernoulli number. We express Ramanujan’s q-series as explicit “traces of partition Eisenstein series.”
As an important step toward this goal, we first derive generating functions for his series. In terms of Dedekind’s eta-function
![]() $\eta (q):=q^{\frac {1}{24}}\prod _{n=1}^{\infty }(1-q^n)$
and Jacobi–Kronecker quadratic characters, we have the following result.
$\eta (q):=q^{\frac {1}{24}}\prod _{n=1}^{\infty }(1-q^n)$
and Jacobi–Kronecker quadratic characters, we have the following result.
Theorem 1.1. As a power series in X, the following are true.
(1) If
![]() $\chi _{-4}(\cdot )=\left (\frac {-4}{\cdot }\right ),$
then we have
$\chi _{-4}(\cdot )=\left (\frac {-4}{\cdot }\right ),$
then we have
 $$ \begin{align*}\sum_{t\geq0}(-1)^t U_{2t}(q) \cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2 \eta(q)^3}\cdot \sum_{n\in\mathbb{Z}} \chi_{-4}(n)q^{\frac{n^2}8}\sin(nX). \end{align*} $$
$$ \begin{align*}\sum_{t\geq0}(-1)^t U_{2t}(q) \cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2 \eta(q)^3}\cdot \sum_{n\in\mathbb{Z}} \chi_{-4}(n)q^{\frac{n^2}8}\sin(nX). \end{align*} $$
(2) If
![]() $\chi _{12}(\cdot )=\left (\frac {12}{\cdot }\right ),$
then we have
$\chi _{12}(\cdot )=\left (\frac {12}{\cdot }\right ),$
then we have
 $$ \begin{align*}\sum_{t\geq0}(-1)^t V_{2t}(q) \cdot \frac{X^{2t}}{(2t)!}= \frac{1}{2 \eta(q)}\cdot \sum_{n\in\mathbb{Z}} \chi_{12}(n)q^{\frac{n^2}{24}}\cos(nX). \end{align*} $$
$$ \begin{align*}\sum_{t\geq0}(-1)^t V_{2t}(q) \cdot \frac{X^{2t}}{(2t)!}= \frac{1}{2 \eta(q)}\cdot \sum_{n\in\mathbb{Z}} \chi_{12}(n)q^{\frac{n^2}{24}}\cos(nX). \end{align*} $$
Remark 1.2. Theorem 1.1 represents two special cases of Lemma 2.1, which pertains to arbitrary theta functions. Using Theorem 1.1, we obtain Theorem 3.4 that gives two further identities for these particular generating functions as infinite products in trigonometric functions.
These generating functions shall offer the connection to traces of partition Eisenstein series. To make this precise, we recall that a partition of n is any nonincreasing sequence of positive integers
![]() $\lambda =(\lambda _1,\lambda _2,\dots , \lambda _s)$
that sum to n, denoted
$\lambda =(\lambda _1,\lambda _2,\dots , \lambda _s)$
that sum to n, denoted
![]() $\lambda \vdash n.$
Equivalently, we use the notation
$\lambda \vdash n.$
Equivalently, we use the notation
![]() $\lambda =(1^{m_1},\dots ,n^{m_n})\vdash n$
, where
$\lambda =(1^{m_1},\dots ,n^{m_n})\vdash n$
, where
![]() $m_j$
is the multiplicity of
$m_j$
is the multiplicity of
![]() $j.$
For such
$j.$
For such
![]() $\lambda ,$
we define the weight
$\lambda ,$
we define the weight
![]() $2n$
partition Eisenstein series
Footnote
1
$2n$
partition Eisenstein series
Footnote
1
The Eisenstein series
![]() $E_{2k}(q)$
corresponds to the partition
$E_{2k}(q)$
corresponds to the partition
![]() $\lambda =(k)$
, as we have
$\lambda =(k)$
, as we have
![]() $E_{(k^1)}(q)=E_{2k}(q)^1.$
$E_{(k^1)}(q)=E_{2k}(q)^1.$
To define partition traces, suppose that
![]() $\phi : \mathcal {P}\mapsto {\mathbb C}$
is a function on partitions. For each positive integer n, its partition Eisenstein trace is the weight
$\phi : \mathcal {P}\mapsto {\mathbb C}$
is a function on partitions. For each positive integer n, its partition Eisenstein trace is the weight
![]() $2n$
quasimodular form
$2n$
quasimodular form
 $$ \begin{align} \operatorname{\mathrm{Tr}}_n(\phi;q):=\sum_{\lambda \vdash n} \phi(\lambda)E_{\lambda}(q). \end{align} $$
$$ \begin{align} \operatorname{\mathrm{Tr}}_n(\phi;q):=\sum_{\lambda \vdash n} \phi(\lambda)E_{\lambda}(q). \end{align} $$
Such traces arise in recent work on MacMahon’s sums-of-divisors q-series (see Thm. 1.4 of [Reference Amdeberhan, Ono and Singh1]).
For partitions
![]() $\lambda =(1^{m_1},\dots ,n^{m_n})\vdash n$
, we require the following functions:
$\lambda =(1^{m_1},\dots ,n^{m_n})\vdash n$
, we require the following functions:
 $$ \begin{align} \phi_U(\lambda):=4^{n}(2n+1)! \cdot \prod_{k=1}^n \frac{1}{m_k !}\left(\frac{B_{2k}}{(2{\color{black}k}) \, (2k)!}\right)^{m_k} , \end{align} $$
$$ \begin{align} \phi_U(\lambda):=4^{n}(2n+1)! \cdot \prod_{k=1}^n \frac{1}{m_k !}\left(\frac{B_{2k}}{(2{\color{black}k}) \, (2k)!}\right)^{m_k} , \end{align} $$
 $$ \begin{align} \phi_V(\lambda):=4^n(2n)! \cdot\prod_{k=1}^n \frac1{m_k!} \left(\frac{(4^k-1)B_{2k}}{(2{\color{black}k})\, (2k)!}\right)^{m_k}. \end{align} $$
$$ \begin{align} \phi_V(\lambda):=4^n(2n)! \cdot\prod_{k=1}^n \frac1{m_k!} \left(\frac{(4^k-1)B_{2k}}{(2{\color{black}k})\, (2k)!}\right)^{m_k}. \end{align} $$
Ramanujan’s series are weighted traces of partition Eisenstein series of these functions.
Theorem 1.3. If t is a positive integer, then the following are true.
(1) We have that
![]() $U_{2t}(q)=\operatorname {\mathrm {Tr}}_t(\phi _U;q).$
$U_{2t}(q)=\operatorname {\mathrm {Tr}}_t(\phi _U;q).$
(2) We have that
![]() $V_{2t}(q)=\operatorname {\mathrm {Tr}}_t(\phi _V;q).$
$V_{2t}(q)=\operatorname {\mathrm {Tr}}_t(\phi _V;q).$
Examples. Here we offer examples of Theorem 1.3.
(1) By direct calculation, we find for
![]() $t=3$
that
$t=3$
that
This reproduces Ramanujan’s identity
(2) By direct calculation, we find for
![]() $t=4$
that
$t=4$
that
 $$ \begin{align*}\phi_V((4^1))&=-272, \phi_V((1^1, 3^1))=448, \phi_V((2^2))=140, \ \phi_V((1^2, 2^1))\\&=-420, \ \mathrm{and}\ \phi_V((1^4))=105. \end{align*} $$
$$ \begin{align*}\phi_V((4^1))&=-272, \phi_V((1^1, 3^1))=448, \phi_V((2^2))=140, \ \phi_V((1^2, 2^1))\\&=-420, \ \mathrm{and}\ \phi_V((1^4))=105. \end{align*} $$
Therefore, we have that
(3) Similar calculations give expressions for the weight 14 quasimodular forms
![]() $U_{14}$
and
$U_{14}$
and
![]() $V_{14}$
$V_{14}$
 $$ \begin{align*} U_{14} &=\frac1{405} (25025E_2^7 - 210210E_2^5E_4 + 420420E_2^3E_4^2 - 168168E_2E_4^3 + 400400E_2^4E_6 \\ &\quad - 960960E_2^2E_4E_6 + 192192E_4^2E_6 + 366080E_2E_6^2 - 720720E_2^3E_8 + 864864E_2E_4E_8 \\ &\quad - 329472E_6E_8 + 1048320E_2^2E_{10} - 419328E_4E_{10} - 1061376E_2E_{12} + 552960E_{14}), \\ V_{14} &=135135E_2^7 - 1891890E_2^5E_4 + 6306300E_2^3E_4^2 - 4204200E_2E_4^3 + 5045040E_2^4E_6 \\ & \quad - 20180160E_2^2E_4E_6 + 6726720E_4^2E_6 + 10762752E_2E_6^2 - 12252240E_2^3E_8 \\ & \quad + 24504480E_2E_4E_8 - 13069056E_6E_8 + 23831808E_2^2E_{10} - 15887872E_4E_{10} \\ & \quad - 32195072E_2E_{12} + 22368256E_{14}. \end{align*} $$
$$ \begin{align*} U_{14} &=\frac1{405} (25025E_2^7 - 210210E_2^5E_4 + 420420E_2^3E_4^2 - 168168E_2E_4^3 + 400400E_2^4E_6 \\ &\quad - 960960E_2^2E_4E_6 + 192192E_4^2E_6 + 366080E_2E_6^2 - 720720E_2^3E_8 + 864864E_2E_4E_8 \\ &\quad - 329472E_6E_8 + 1048320E_2^2E_{10} - 419328E_4E_{10} - 1061376E_2E_{12} + 552960E_{14}), \\ V_{14} &=135135E_2^7 - 1891890E_2^5E_4 + 6306300E_2^3E_4^2 - 4204200E_2E_4^3 + 5045040E_2^4E_6 \\ & \quad - 20180160E_2^2E_4E_6 + 6726720E_4^2E_6 + 10762752E_2E_6^2 - 12252240E_2^3E_8 \\ & \quad + 24504480E_2E_4E_8 - 13069056E_6E_8 + 23831808E_2^2E_{10} - 15887872E_4E_{10} \\ & \quad - 32195072E_2E_{12} + 22368256E_{14}. \end{align*} $$
Then, using the identities
we get
 $$ \begin{align*} U_{14} &=\frac1{27}(-3648E_4^2E_6 - 17920E_2E_6^2 + 19320E_2E_4^3 - 300300E_2^3E_4^2 \\ & \qquad + 400400E_2^4E_6 - 210210E_2^5E_4 + 87360E_2^2E_4E_6 + 25025E_2^7), \\ V_{14} &=138048E_4^2E_6 - 885248E_2E_6^2 - 246792E_2E_4^3 - 5945940E_2^3E_4^2 \\ & \qquad + 5045040E_2^4E_6 - 1891890E_2^5E_4 + 3651648E_2^2E_4E_6 + 135135E_2^7. \end{align*} $$
$$ \begin{align*} U_{14} &=\frac1{27}(-3648E_4^2E_6 - 17920E_2E_6^2 + 19320E_2E_4^3 - 300300E_2^3E_4^2 \\ & \qquad + 400400E_2^4E_6 - 210210E_2^5E_4 + 87360E_2^2E_4E_6 + 25025E_2^7), \\ V_{14} &=138048E_4^2E_6 - 885248E_2E_6^2 - 246792E_2E_4^3 - 5945940E_2^3E_4^2 \\ & \qquad + 5045040E_2^4E_6 - 1891890E_2^5E_4 + 3651648E_2^2E_4E_6 + 135135E_2^7. \end{align*} $$
Remark 1.4. The coefficients
![]() $\phi _V(\lambda )$
are always integers. The first author and other collaborators have found a combinatorial interpretation and a proof this fact, which will appear in a forthcoming work.
$\phi _V(\lambda )$
are always integers. The first author and other collaborators have found a combinatorial interpretation and a proof this fact, which will appear in a forthcoming work.
In view of these results, it is natural to pose the following problem.
Problem. Determine and characterize further functions
![]() $\phi : \mathcal {P}\mapsto {\mathbb C}$
for which
$\phi : \mathcal {P}\mapsto {\mathbb C}$
for which
![]() $\{\operatorname {\mathrm {Tr}}_t(\phi ;q)\}$
is a natural and rich family of weight
$\{\operatorname {\mathrm {Tr}}_t(\phi ;q)\}$
is a natural and rich family of weight
![]() $2t$
quasimodular forms.
$2t$
quasimodular forms.
To prove these results, we make use of the Jacobi Triple Product identity, special q-series, exponential generating functions for Bernoulli numbers, and properties of Pólya’s cycle index polynomials. In Section 2, we derive a general result for q-series of the form (1.1) and (1.2) (see Lemma 2.1), which gives Theorem 1.1 as special cases. In Section 3, we prove Theorem 1.3 using these results and properties of Pólya’s cycle index polynomials.
2 Generating functions for Ramanujan-type q-series
Theorem 1.1 gives two special cases of general generating functions associated to formal theta functions for Dirichlet characters. If
![]() $\chi $
modulo N is a Dirichlet character, then let
$\chi $
modulo N is a Dirichlet character, then let
 $$ \begin{align} \Theta(\chi;q):=\sum_{n=1}^{\infty} \chi(n)n^{a_{\chi}}q^{n^2}, \end{align} $$
$$ \begin{align} \Theta(\chi;q):=\sum_{n=1}^{\infty} \chi(n)n^{a_{\chi}}q^{n^2}, \end{align} $$
where we let
 $$ \begin{align} a_{\chi}:=\begin{cases} 0 \ \ \ \ \ &\mathrm{if } \chi \text{ is even},\\ 1 \ \ \ \ \ &\text{if } \chi \text{ is odd.} \end{cases} \end{align} $$
$$ \begin{align} a_{\chi}:=\begin{cases} 0 \ \ \ \ \ &\mathrm{if } \chi \text{ is even},\\ 1 \ \ \ \ \ &\text{if } \chi \text{ is odd.} \end{cases} \end{align} $$
Then, in analogy with Ramanujan’s
![]() $U_{2t}$
and
$U_{2t}$
and
![]() $V_{2t}$
(see (1.1) and (1.2)), we let
$V_{2t}$
(see (1.1) and (1.2)), we let
 $$ \begin{align} R_{2t}(\chi;q):=\frac{D^t\left(\Theta(\chi;q)\right)}{\Theta(\chi;q)}= \frac{\sum_{n=1}^{\infty} \chi(n)n^{2t+a_{\chi}}q^{n^2}}{\Theta(\chi;q)}. \end{align} $$
$$ \begin{align} R_{2t}(\chi;q):=\frac{D^t\left(\Theta(\chi;q)\right)}{\Theta(\chi;q)}= \frac{\sum_{n=1}^{\infty} \chi(n)n^{2t+a_{\chi}}q^{n^2}}{\Theta(\chi;q)}. \end{align} $$
Lemma 2.1. Assuming the notation above, as a power series in X we have
 $$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi;q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\Theta(\chi;q)}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_{\chi}-1}\cdot e^{inX}. \end{align*} $$
$$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi;q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\Theta(\chi;q)}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_{\chi}-1}\cdot e^{inX}. \end{align*} $$
Remark 2.2. Lemma 2.1 holds for periodic functions
![]() $\chi : {\mathbb Z}\rightarrow {\mathbb C}$
that are either even or odd.
$\chi : {\mathbb Z}\rightarrow {\mathbb C}$
that are either even or odd.
Proof. By direct calculation, we have that
 $$ \begin{align*}\frac{1}{2i}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_\chi-1}\cdot e^{inX}= \frac{1}{2i} \sum_{n=1}^{\infty}q^{n^2}n^{a_\chi-1}\left(\chi(n)e^{inX}+(-1)^{a_\chi-1}\chi(-n)e^{-inX}\right). \end{align*} $$
$$ \begin{align*}\frac{1}{2i}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_\chi-1}\cdot e^{inX}= \frac{1}{2i} \sum_{n=1}^{\infty}q^{n^2}n^{a_\chi-1}\left(\chi(n)e^{inX}+(-1)^{a_\chi-1}\chi(-n)e^{-inX}\right). \end{align*} $$
For all
![]() $\chi ,$
we have that
$\chi ,$
we have that
![]() $(-1)^{a_\chi -1}\chi (-n)=-\chi (n),$
and so this reduces to
$(-1)^{a_\chi -1}\chi (-n)=-\chi (n),$
and so this reduces to
 $$ \begin{align*}\frac{1}{2i}\sum_{n=1}^{\infty}\chi(n)q^{n^2}n^{a_\chi-1}\left(e^{inX}-e^{-inX}\right)= \sum_{n=1}^{\infty}\chi(n)q^{n^2}n^{a_\chi-1}\sin(nX). \end{align*} $$
$$ \begin{align*}\frac{1}{2i}\sum_{n=1}^{\infty}\chi(n)q^{n^2}n^{a_\chi-1}\left(e^{inX}-e^{-inX}\right)= \sum_{n=1}^{\infty}\chi(n)q^{n^2}n^{a_\chi-1}\sin(nX). \end{align*} $$
Using the Taylor series for
![]() $\sin (nX)$
, this gives (after change of summation)
$\sin (nX)$
, this gives (after change of summation)
 $$ \begin{align*} \frac{1}{2i}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_\chi-1}\cdot e^{inX}&= \sum_{n=1}^{\infty}\chi(n)n^{a_\chi-1}q^{n^2}\sum_{t=0}^{\infty}(-1)^t\cdot \frac{(nX)^{2t+1}}{(2t+1)!}\\ &=\sum_{t=0}^{\infty}(-1)^t\cdot \frac{X^{2t+1}}{(2t+1)!}\cdot D^t(\Theta(\chi;q)). \end{align*} $$
$$ \begin{align*} \frac{1}{2i}\sum_{n\in {\mathbb Z}} \chi(n)q^{n^2}n^{a_\chi-1}\cdot e^{inX}&= \sum_{n=1}^{\infty}\chi(n)n^{a_\chi-1}q^{n^2}\sum_{t=0}^{\infty}(-1)^t\cdot \frac{(nX)^{2t+1}}{(2t+1)!}\\ &=\sum_{t=0}^{\infty}(-1)^t\cdot \frac{X^{2t+1}}{(2t+1)!}\cdot D^t(\Theta(\chi;q)). \end{align*} $$
Thanks to (2.3), we obtain the claimed generating function by dividing through by
![]() $\Theta (\chi ;q).$
$\Theta (\chi ;q).$
Proof of Theorem 1.1
To prove claim (1), we consider
![]() ${\color {black}\chi _{-4}(n)}:= \left (\frac {-4}n\right ),$
which is the only odd character modulo
${\color {black}\chi _{-4}(n)}:= \left (\frac {-4}n\right ),$
which is the only odd character modulo
![]() $4$
. In this case, we have
$4$
. In this case, we have
![]() $a_{\color {black}\chi _{-4}}=1$
, and so Lemma 2.1 gives
$a_{\color {black}\chi _{-4}}=1$
, and so Lemma 2.1 gives
 $$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}({\color{black}\chi_{-4}};q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\Theta({\color{black}\chi_{-4}};q)}\sum_{n\in {\mathbb Z}} \chi_{-4}(n)q^{n^2}\cdot e^{inX}. \end{align*} $$
$$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}({\color{black}\chi_{-4}};q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\Theta({\color{black}\chi_{-4}};q)}\sum_{n\in {\mathbb Z}} \chi_{-4}(n)q^{n^2}\cdot e^{inX}. \end{align*} $$
Furthermore, Jacobi’s classical identity (for example, see p. 17 of [Reference Ono11]) implies that
 $$ \begin{align*}\eta(q^8)^3 = \Theta(\chi_{-4};q)=\sum_{n=0}^{\infty} (-1)^n (2n+1)q^{(2n+1)^2}. \end{align*} $$
$$ \begin{align*}\eta(q^8)^3 = \Theta(\chi_{-4};q)=\sum_{n=0}^{\infty} (-1)^n (2n+1)q^{(2n+1)^2}. \end{align*} $$
Therefore, we have that
 $$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi_{-4};q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\eta(q^8)^3}\sum_{n\in {\mathbb Z}} \chi_{-4}(n)q^{n^2}\cdot e^{inX}. \end{align*} $$
$$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi_{-4};q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\eta(q^8)^3}\sum_{n\in {\mathbb Z}} \chi_{-4}(n)q^{n^2}\cdot e^{inX}. \end{align*} $$
Claim (1) follows by letting
![]() $q\rightarrow q^{\frac {1}{8}},$
replacing the complex exponential in terms of trigonometric functions, followed by taking the real part.
$q\rightarrow q^{\frac {1}{8}},$
replacing the complex exponential in terms of trigonometric functions, followed by taking the real part.
To prove claim (2), we note that Euler’s Pentagonal Number Theorem (see p. 17 of [Reference Ono11]) implies that
 $$ \begin{align*}\eta(q^{24})=\sum_{n=1}^{\infty} \chi_{12}(n)q^{n^2}, \end{align*} $$
$$ \begin{align*}\eta(q^{24})=\sum_{n=1}^{\infty} \chi_{12}(n)q^{n^2}, \end{align*} $$
where
![]() $\chi _{12}(n)=\left (\frac {12}n\right )$
is the unique primitive character with conductor
$\chi _{12}(n)=\left (\frac {12}n\right )$
is the unique primitive character with conductor
![]() $12.$
Therefore,
$12.$
Therefore,
![]() $a_{\chi }=0$
, and so Lemma 2.1 gives
$a_{\chi }=0$
, and so Lemma 2.1 gives
 $$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi_{12};q) \cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\eta(q^{24})}\sum_{n\in {\mathbb Z}} \chi_{12}(n)\frac{q^{n^2}}{n}\cdot e^{inX}. \end{align*} $$
$$ \begin{align*}\sum_{t=0}^{\infty}(-1)^t R_{2t}(\chi_{12};q) \cdot \frac{X^{2t+1}}{(2t+1)!}= \frac{1}{2i\eta(q^{24})}\sum_{n\in {\mathbb Z}} \chi_{12}(n)\frac{q^{n^2}}{n}\cdot e^{inX}. \end{align*} $$
Claim (2) follows by letting
![]() $q\rightarrow q^{\frac {1}{24}},$
then differentiating in X, followed by taking the real part as in (1).
$q\rightarrow q^{\frac {1}{24}},$
then differentiating in X, followed by taking the real part as in (1).
3 Proof of Theorem 1.3
Here we prove Theorem 1.3 using the generating functions in Theorem 1.1. We apply Pólya’s cycle index polynomials and the exponential generating function for Bernoulli numbers.
3.1 Bernoulli numbers
Here we recall a convenient generating function for Bernoulli numbers, and we refer the reader to [Reference Gradshteyn and Ryzhik8, 1.518.1]. If
![]() $\mathrm {sinc}(X):=\sin X/X$
, then we have
$\mathrm {sinc}(X):=\sin X/X$
, then we have
 $$ \begin{align} \frac1{\mathrm{sinc}(X)}&=\exp\left(-\sum_{k\geq1}\frac{(-4)^kB_{2k}}{(2k)(2k)!}\cdot X^{2k}\right). \end{align} $$
$$ \begin{align} \frac1{\mathrm{sinc}(X)}&=\exp\left(-\sum_{k\geq1}\frac{(-4)^kB_{2k}}{(2k)(2k)!}\cdot X^{2k}\right). \end{align} $$
3.2 Pólya’s cycle index polynomials
We require Pólya’s cycle index polynomials in the case of symmetric groups (for example, see [Reference Stanley14]). Namely, recall that given a partition
![]() $\lambda =(\lambda _1,\dots ,\lambda _{\ell (\lambda )})\vdash t$
or
$\lambda =(\lambda _1,\dots ,\lambda _{\ell (\lambda )})\vdash t$
or
![]() $(1^{m_1},\dots ,t^{m_t})\vdash t$
, we have that the number of permutations in
$(1^{m_1},\dots ,t^{m_t})\vdash t$
, we have that the number of permutations in
![]() $\mathfrak {S}_t$
of cycle type
$\mathfrak {S}_t$
of cycle type
![]() $\lambda $
is
$\lambda $
is
![]() $t!/z_{\lambda }$
, where
$t!/z_{\lambda }$
, where
![]() $z_{\lambda }:=1^{m_1}\cdots t^{m_t}m_1!\cdots m_t!$
. The cycle index polynomial for the symmetric group
$z_{\lambda }:=1^{m_1}\cdots t^{m_t}m_1!\cdots m_t!$
. The cycle index polynomial for the symmetric group
![]() $\mathfrak {S}_t$
is given by
$\mathfrak {S}_t$
is given by
 $$ \begin{align} Z(\mathfrak{S}_t)&=\sum_{\lambda\vdash t}\frac1{z_{\lambda}}\prod_{i=1}^{\ell(\lambda)}x_{\lambda_i} =\sum_{\lambda\vdash t}\prod_{k=1}^t\frac1{m_k!}\left(\frac{x_k}{k}\right)^{m_k}. \end{align} $$
$$ \begin{align} Z(\mathfrak{S}_t)&=\sum_{\lambda\vdash t}\frac1{z_{\lambda}}\prod_{i=1}^{\ell(\lambda)}x_{\lambda_i} =\sum_{\lambda\vdash t}\prod_{k=1}^t\frac1{m_k!}\left(\frac{x_k}{k}\right)^{m_k}. \end{align} $$
We require the following well-known generating function in t-aspect.
Lemma 3.1. (Example 5.2.10 of [Reference Stanley14]) As a power series in y, the generating function for the cycle index polynomials satisfies
 $$ \begin{align*}\sum_{t\geq0}Z(\mathfrak{S}_t)\,y^t=\exp\left(\sum_{k\geq1}x_k\frac{y^k}k\right).\end{align*} $$
$$ \begin{align*}\sum_{t\geq0}Z(\mathfrak{S}_t)\,y^t=\exp\left(\sum_{k\geq1}x_k\frac{y^k}k\right).\end{align*} $$
Example 3.2. Here are the first few examples of Pólya’s cycle index polynomials:
3.3 Some power series identities
We consider the infinite series factors of the functions in Theorem 1.1.
Lemma 3.3. As a power series in X, the following are true.
(1) We have that
 $$ \begin{align*}\frac{q^{-\frac{1}{8}}}{2}\cdot\sum_{n\in\mathbb{Z}} \chi_{-4} (n)\cdot q^{\frac{n^2}8}\sin(nX) =\sin X \cdot \prod_{j\geq1}(1-q^j)(1-2\cos(2X)q^j+q^{2j}). \end{align*} $$
$$ \begin{align*}\frac{q^{-\frac{1}{8}}}{2}\cdot\sum_{n\in\mathbb{Z}} \chi_{-4} (n)\cdot q^{\frac{n^2}8}\sin(nX) =\sin X \cdot \prod_{j\geq1}(1-q^j)(1-2\cos(2X)q^j+q^{2j}). \end{align*} $$
(2) We have that
 $$ \begin{align*} \frac{q^{-\frac{1}{24}}}{2}&\sum_{n\in\mathbb{Z}} \chi_{12} (n)\cdot q^{\frac{n^2}{24}} \cos(nX)\\ &\ \ \ \ = \cos X\prod_{n\geq1}(1-q^n)(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}). \end{align*} $$
$$ \begin{align*} \frac{q^{-\frac{1}{24}}}{2}&\sum_{n\in\mathbb{Z}} \chi_{12} (n)\cdot q^{\frac{n^2}{24}} \cos(nX)\\ &\ \ \ \ = \cos X\prod_{n\geq1}(1-q^n)(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}). \end{align*} $$
Proof. Both claims follow from the Jacobi Triple Product Identity (see Th. 2.8 of [Reference Andrews2])
 $$ \begin{align} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2}2}z^n=\prod_{j\geq1}(1-q^j)(1-q^{j-\frac{1}2}z)(1-q^{j-\frac{1}2}z^{-1}). \end{align} $$
$$ \begin{align} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2}2}z^n=\prod_{j\geq1}(1-q^j)(1-q^{j-\frac{1}2}z)(1-q^{j-\frac{1}2}z^{-1}). \end{align} $$
To prove (1), we make the substitutions
![]() $2i\sin X=e^{iX}(1-e^{-2iX})$
and
$2i\sin X=e^{iX}(1-e^{-2iX})$
and
![]() $z=q^{\frac 12}e^{2iX}$
to obtain
$z=q^{\frac 12}e^{2iX}$
to obtain
 $$ \begin{align*} \frac1{2i} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2+n}{2}}e^{(2n+1)iX}=\sin X\prod_{j\geq1}(1-q^j)(1-q^je^{2iX})(1-q^je^{-2iX}). \end{align*} $$
$$ \begin{align*} \frac1{2i} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2+n}{2}}e^{(2n+1)iX}=\sin X\prod_{j\geq1}(1-q^j)(1-q^je^{2iX})(1-q^je^{-2iX}). \end{align*} $$
To obtain claim (1), we note the following simple reformulation
 $$ \begin{align*} \frac1{2i} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2+n}{2}}e^{(2n+1)iX} =\frac{q^{-\frac{1}{8}}}{2i}\cdot\sum_{n\in\mathbb{Z}} \chi_{-4} (n)\cdot q^{\frac{n^2}8}e^{inX}, \end{align*} $$
$$ \begin{align*} \frac1{2i} \sum_{n\in\mathbb{Z}}(-1)^nq^{\frac{n^2+n}{2}}e^{(2n+1)iX} =\frac{q^{-\frac{1}{8}}}{2i}\cdot\sum_{n\in\mathbb{Z}} \chi_{-4} (n)\cdot q^{\frac{n^2}8}e^{inX}, \end{align*} $$
and then take the real part of both sides.
We now turn to claim (2). Thanks to [Reference Carlitz and Subbarao7, (1.1)], we obtain
 $$ \begin{align*}\sum_{n\in\mathbb{Z}} q^{n(3n+1)}(z^{3n}-z^{-3n-1}) &=\prod_{n\geq1}(1-q^{2n})(1-zq^{2n})\left(1-\frac{q^{2n-2}}{z}\right)\\&\quad \times (1-z^2q^{4n-2})\left(1-\frac{q^{4n-2}}{z^2}\right).\end{align*} $$
$$ \begin{align*}\sum_{n\in\mathbb{Z}} q^{n(3n+1)}(z^{3n}-z^{-3n-1}) &=\prod_{n\geq1}(1-q^{2n})(1-zq^{2n})\left(1-\frac{q^{2n-2}}{z}\right)\\&\quad \times (1-z^2q^{4n-2})\left(1-\frac{q^{4n-2}}{z^2}\right).\end{align*} $$
By replacing
![]() $q\rightarrow q^{\frac {1}{2}}, z\rightarrow -z^2$
, factoring out
$q\rightarrow q^{\frac {1}{2}}, z\rightarrow -z^2$
, factoring out
![]() $1+z^{-2}$
and multiplying through by z, we get
$1+z^{-2}$
and multiplying through by z, we get
 $$ \begin{align*}\sum_{n\in\mathbb{Z}} (-1)^nq^{\frac{n(3n+1)}2}\frac{z^{6n+1}+z^{-6n-1}}{z+z^{-1}} &=\prod_{n\geq1}(1-q^n)(1+z^2q^n)\left(1+\frac{q^n}{z^2}\right)\\&\quad \times (1-z^4q^{2n-1})\left(1-\frac{q^{2n-1}}{z^4}\right).\end{align*} $$
$$ \begin{align*}\sum_{n\in\mathbb{Z}} (-1)^nq^{\frac{n(3n+1)}2}\frac{z^{6n+1}+z^{-6n-1}}{z+z^{-1}} &=\prod_{n\geq1}(1-q^n)(1+z^2q^n)\left(1+\frac{q^n}{z^2}\right)\\&\quad \times (1-z^4q^{2n-1})\left(1-\frac{q^{2n-1}}{z^4}\right).\end{align*} $$
After letting
![]() $z=e^{iX},$
we pair up conjugate terms to get
$z=e^{iX},$
we pair up conjugate terms to get
 $$ \begin{align*} &\sum_{n\in\mathbb{Z}} (-1)^nq^{\frac{n(3n+1)}2}\cos(6n+1)X\\ &\hskip.5in =\cos X \prod_{n\geq1}(1-q^n)(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}). \end{align*} $$
$$ \begin{align*} &\sum_{n\in\mathbb{Z}} (-1)^nq^{\frac{n(3n+1)}2}\cos(6n+1)X\\ &\hskip.5in =\cos X \prod_{n\geq1}(1-q^n)(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}). \end{align*} $$
The left hand side of the expression above equals the infinite sum in Lemma 3.3 (2).
To prove Theorem 1.3, we also require the following power series identities.
Theorem 3.4. The following identities are true.
(1) As power series in X, we have
 $$ \begin{align*}\sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \sin X \cdot \prod_{j\geq1}\left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align*} $$
$$ \begin{align*}\sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}= \sin X \cdot \prod_{j\geq1}\left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align*} $$
(2) As power series in X, we have
 $$ \begin{align*}\sum_{t\geq0} (-1)^t V_{2t}(q)\cdot \frac{X^{2t}}{(2t)!}= \cos X \cdot \prod_{j\geq1}\left[1-\frac{4(\sin^2X)q^j}{(1+q^j)^2}\right]\left[1+\frac{4(\sin^22X)q^{2j-1}}{(1-q^{2j-1})^2}\right]. \end{align*} $$
$$ \begin{align*}\sum_{t\geq0} (-1)^t V_{2t}(q)\cdot \frac{X^{2t}}{(2t)!}= \cos X \cdot \prod_{j\geq1}\left[1-\frac{4(\sin^2X)q^j}{(1+q^j)^2}\right]\left[1+\frac{4(\sin^22X)q^{2j-1}}{(1-q^{2j-1})^2}\right]. \end{align*} $$
Proof. We first prove claim (1). By combining Theorem 1.1 (1) and Lemma 3.3 (1), we obtain
 $$ \begin{align*} \sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}=\sin X\cdot \prod_{j\geq1}\frac{(1-2\cos(2X)q^j+q^{2j})}{(1-q^j)^2}. \end{align*} $$
$$ \begin{align*} \sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}=\sin X\cdot \prod_{j\geq1}\frac{(1-2\cos(2X)q^j+q^{2j})}{(1-q^j)^2}. \end{align*} $$
A straightforward algebraic manipulation with
![]() $-2\cos (2X)=-2+4\sin ^2X$
yields
$-2\cos (2X)=-2+4\sin ^2X$
yields
 $$ \begin{align*}\sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}=\sin X \cdot \prod_{j\geq1}\left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align*} $$
$$ \begin{align*}\sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!}=\sin X \cdot \prod_{j\geq1}\left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align*} $$
Now we turn to claim (2). By combining Theorem 1.1 (2) and Lemma 3.3 (2), we obtain
 $$ \begin{align*} \sum_{t\geq0} (-1)^t V_{2t}(q)\cdot \frac{X^{2t}}{(2t)!}& =\cos X\prod_{n\geq1}(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}) \\ & =\cos X \prod_{k\geq1}(1+q^k)^2(1-q^{2k-1})^2 \prod_{j\geq1}\left[1-\frac{4(\sin^2X)q^j}{(1+q^j)^2}\right]\\&\quad\left[1+\frac{4(\sin^22X)q^{2j-1}}{(1-q^{2j-1})^2}\right]. \end{align*} $$
$$ \begin{align*} \sum_{t\geq0} (-1)^t V_{2t}(q)\cdot \frac{X^{2t}}{(2t)!}& =\cos X\prod_{n\geq1}(1+2\cos(2X)q^n+q^{2n})(1-2\cos(4X)q^{2n-1}+q^{4n-2}) \\ & =\cos X \prod_{k\geq1}(1+q^k)^2(1-q^{2k-1})^2 \prod_{j\geq1}\left[1-\frac{4(\sin^2X)q^j}{(1+q^j)^2}\right]\\&\quad\left[1+\frac{4(\sin^22X)q^{2j-1}}{(1-q^{2j-1})^2}\right]. \end{align*} $$
The proof now follows from the simple identity
 $$ \begin{align*}\prod_{k=1}^{\infty}(1+q^k)=\prod_{k=1}^{\infty}\frac1{1-q^{2k-1}}. \end{align*} $$
$$ \begin{align*}\prod_{k=1}^{\infty}(1+q^k)=\prod_{k=1}^{\infty}\frac1{1-q^{2k-1}}. \end{align*} $$
3.4 Proof of Theorem 1.3
For each positive odd integer j, we consider the Lambert series
 $$ \begin{align} \mathbf{S}_j(q):=\sum_{m\geq1}\frac{m^jq^m}{1-q^m} = \frac{B_{j+1}}{2(j+1)} - \frac{B_{j+1}}{2(j+1)}E_{j+1}(q). \end{align} $$
$$ \begin{align} \mathbf{S}_j(q):=\sum_{m\geq1}\frac{m^jq^m}{1-q^m} = \frac{B_{j+1}}{2(j+1)} - \frac{B_{j+1}}{2(j+1)}E_{j+1}(q). \end{align} $$
This expression in terms of the
![]() $E_{j+1}(q)$
follows from (1.4). The proof of Theorem 1.3 boils down to deriving expressions for the power series in Theorem 3.4 in terms of the
$E_{j+1}(q)$
follows from (1.4). The proof of Theorem 1.3 boils down to deriving expressions for the power series in Theorem 3.4 in terms of the
![]() $\mathbf {S}_j(q).$
$\mathbf {S}_j(q).$
Proof of Theorem 1.3
We first prove claim (1) regarding Ramanujan’s
![]() $U_{2t}$
series. The key fact underlying the proof is the following power series identity.
$U_{2t}$
series. The key fact underlying the proof is the following power series identity.
 $$ \begin{align} \sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!} =\sin X\cdot \exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r \right). \end{align} $$
$$ \begin{align} \sum_{t\geq0} (-1)^t U_{2t}(q)\cdot \frac{X^{2t+1}}{(2t+1)!} =\sin X\cdot \exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r \right). \end{align} $$
Thanks to Theorem 3.4 (1), this identity will follow from
 $$ \begin{align} \exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r\right)= \prod_{j\geq1} \left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align} $$
$$ \begin{align} \exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r\right)= \prod_{j\geq1} \left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]. \end{align} $$
To establish (3.6), we compute the following double-sum in two different ways. First, we use the Taylor expansion of
![]() $\cos (y)$
and then interchange the order of summation to get
$\cos (y)$
and then interchange the order of summation to get
 $$ \begin{align*}\sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =\sum_{r\geq0}\frac{(-4X^2)^r}{(2r)!}\sum_{k\geq1}k^{2r-1}\sum_{j\geq1}q^{kj}.\end{align*} $$
$$ \begin{align*}\sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =\sum_{r\geq0}\frac{(-4X^2)^r}{(2r)!}\sum_{k\geq1}k^{2r-1}\sum_{j\geq1}q^{kj}.\end{align*} $$
By combining the geometric series and the Taylor series for
![]() $\log (1-Y)$
with (3.4), we obtain
$\log (1-Y)$
with (3.4), we obtain
 $$ \begin{align} \sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =\sum_{r\geq0}\frac{(-4X^2)^r}{(2r)!}\sum_{k\geq1} \frac{k^{2r-1}q^k}{1-q^k} =-\log(q)_{\infty}+\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r, \end{align} $$
$$ \begin{align} \sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =\sum_{r\geq0}\frac{(-4X^2)^r}{(2r)!}\sum_{k\geq1} \frac{k^{2r-1}q^k}{1-q^k} =-\log(q)_{\infty}+\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r, \end{align} $$
where
![]() $(q)_{\infty }:=\prod _{n=1}^{\infty }(1-q^n)$
is the q-Pochhammer symbol.
$(q)_{\infty }:=\prod _{n=1}^{\infty }(1-q^n)$
is the q-Pochhammer symbol.
On the other hand, using
![]() $2\cos \theta =e^{i\theta }+e^{-i\theta }$
and Taylor expansion of
$2\cos \theta =e^{i\theta }+e^{-i\theta }$
and Taylor expansion of
![]() $\log (1-Y)$
, we find that
$\log (1-Y)$
, we find that
 $$ \begin{align*}\sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k &=\frac12\sum_{j\geq1} \left(\sum_{k\geq1} \frac{(e^{2iX}q^j)^k}k+\sum_{k\geq1} \frac{(e^{-2iX}q^j)^k}k\right)\\ &=-\frac12\sum_{j\geq1} \log\left[1-2(\cos 2X )q^j+q^{2j}\right]. \end{align*} $$
$$ \begin{align*}\sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k &=\frac12\sum_{j\geq1} \left(\sum_{k\geq1} \frac{(e^{2iX}q^j)^k}k+\sum_{k\geq1} \frac{(e^{-2iX}q^j)^k}k\right)\\ &=-\frac12\sum_{j\geq1} \log\left[1-2(\cos 2X )q^j+q^{2j}\right]. \end{align*} $$
After straightforward algebraic manipulation, we get
 $$ \begin{align} \sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =-\log(q)_{\infty}-\frac12\log\left(\prod_{j\geq1} \left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]\right). \end{align} $$
$$ \begin{align} \sum_{j,k\geq1}\frac{q^{kj}\cos(2kX)}k =-\log(q)_{\infty}-\frac12\log\left(\prod_{j\geq1} \left[1+\frac{4(\sin^2X)q^j}{(1-q^j)^2}\right]\right). \end{align} $$
Identity (3.6) follows by comparing (3.7) and (3.8), and in turn confirms (3.5).
We now investigate the exponential series in (3.5). Thanks to (3.4), followed by an application of (3.1), we obtain
 $$ \begin{align*} &\exp\left(-2\sum_{k\geq1}\frac{(-4)^k\mathbf{S}_{2k-1}(q)}{(2k)!} \,X^{2k} \right) \\ &\hskip.4in =\exp\left(-\sum_{k\geq1}\frac{(-4)^kB_{2k}}{(2k)(2k)!}\,X^{2k} \right) \cdot \exp\left(\sum_{k\geq1}\frac{B_{2k}\cdot E_{2k}(q)}{(2k)(2k)!} \,(-4X^2)^k\right) \\ &\hskip.4in =\frac{X}{\sin X}\cdot \exp\left(\sum_{k\geq1}\frac{B_{2k}\cdot E_{2k}(q)}{2\,(2k)!} \,\frac{(-4X^2)^k}k \right). \end{align*} $$
$$ \begin{align*} &\exp\left(-2\sum_{k\geq1}\frac{(-4)^k\mathbf{S}_{2k-1}(q)}{(2k)!} \,X^{2k} \right) \\ &\hskip.4in =\exp\left(-\sum_{k\geq1}\frac{(-4)^kB_{2k}}{(2k)(2k)!}\,X^{2k} \right) \cdot \exp\left(\sum_{k\geq1}\frac{B_{2k}\cdot E_{2k}(q)}{(2k)(2k)!} \,(-4X^2)^k\right) \\ &\hskip.4in =\frac{X}{\sin X}\cdot \exp\left(\sum_{k\geq1}\frac{B_{2k}\cdot E_{2k}(q)}{2\,(2k)!} \,\frac{(-4X^2)^k}k \right). \end{align*} $$
We recognize this last expression in the context of Pólya’s cycle index polynomials. Namely, Lemma 3.1 gives the identity (here
![]() $\lambda =(1^{m_1},\dots, t^{m_t}\vdash t)$
)
$\lambda =(1^{m_1},\dots, t^{m_t}\vdash t)$
)
 $$ \begin{align*}\exp\left(\sum_{k\geq1}Y_k\,\frac{w^k}k\right) =\sum_{t\geq0}\left(\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{Y_k}{k}\right)^{m_k} \right)w^t, \end{align*} $$
$$ \begin{align*}\exp\left(\sum_{k\geq1}Y_k\,\frac{w^k}k\right) =\sum_{t\geq0}\left(\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{Y_k}{k}\right)^{m_k} \right)w^t, \end{align*} $$
which we apply with
![]() $Y_k=\frac {B_{2k}\cdot E_{2k}(q)}{2(2k)!}$
and
$Y_k=\frac {B_{2k}\cdot E_{2k}(q)}{2(2k)!}$
and
![]() $w=-4X^2.$
This gives
$w=-4X^2.$
This gives
 $$ \begin{align*} \sum_{t\geq0} (-1)^t U_{2t}(q)\frac{X^{2t+1}}{(2t+1)!} &=\sin X\cdot \exp\left(-2\sum_{k\geq1}\frac{\mathbf{S}_{2k-1}(q)}{(2k)!} \,(-4X^2)^{k}\right) \\ &=\sin X\cdot\frac{X}{\sin X}\cdot \sum_{t\geq0} \left(\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{B_{2k}\cdot E_{2k}(q)}{(2k)(2k)!}\right)^{m_k}\right)(-4X^2)^t. \end{align*} $$
$$ \begin{align*} \sum_{t\geq0} (-1)^t U_{2t}(q)\frac{X^{2t+1}}{(2t+1)!} &=\sin X\cdot \exp\left(-2\sum_{k\geq1}\frac{\mathbf{S}_{2k-1}(q)}{(2k)!} \,(-4X^2)^{k}\right) \\ &=\sin X\cdot\frac{X}{\sin X}\cdot \sum_{t\geq0} \left(\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{B_{2k}\cdot E_{2k}(q)}{(2k)(2k)!}\right)^{m_k}\right)(-4X^2)^t. \end{align*} $$
By comparing the coefficients of
![]() $X^{2t+1}$
, we find that
$X^{2t+1}$
, we find that
 $$ \begin{align*}(-1)^t \frac{U_{2t}(q)}{(2t+1)!}=(-4)^t\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{B_{2k}\cdot E_{2k}(q)}{{(2\color{black}k)}(2k)!}\right)^{m_k},\end{align*} $$
$$ \begin{align*}(-1)^t \frac{U_{2t}(q)}{(2t+1)!}=(-4)^t\sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{B_{2k}\cdot E_{2k}(q)}{{(2\color{black}k)}(2k)!}\right)^{m_k},\end{align*} $$
which in turn, thanks to (1.7), proves Theorem 1.3 (1).
We now turn to claim (2) regarding Ramanujan’s
![]() $V_{2t}$
whose proof is analogous to the proof of (1). The main difference follows from the need for the generalized Lambert series
$V_{2t}$
whose proof is analogous to the proof of (1). The main difference follows from the need for the generalized Lambert series
 $$ \begin{align} \mathbf{A}_{2r-1}(q):=\sum_{k\geq1}\frac{(-1)^{k-1}k^{2r-1}q^k}{1-q^k} = \mathbf{S}_{2r-1}(q) - 4^r\mathbf{S}_{2r-1}(q^2). \end{align} $$
$$ \begin{align} \mathbf{A}_{2r-1}(q):=\sum_{k\geq1}\frac{(-1)^{k-1}k^{2r-1}q^k}{1-q^k} = \mathbf{S}_{2r-1}(q) - 4^r\mathbf{S}_{2r-1}(q^2). \end{align} $$
The expression in
![]() $\mathbf {S}_{2r-1}$
is straightforward. For the sake of brevity, we note that calculations analogous to the proof of (3.6) give the identity
$\mathbf {S}_{2r-1}$
is straightforward. For the sake of brevity, we note that calculations analogous to the proof of (3.6) give the identity
 $$ \begin{align*}\prod_{n\geq1}\left[1-\frac{4(\sin^2X)q^n}{(1+q^n)^2}\right]= \exp\left(2\sum_{r\geq1}\frac{\mathbf{A}_{2r-1}(q)(-4X^2)^r}{(2r)!} \right), \end{align*} $$
$$ \begin{align*}\prod_{n\geq1}\left[1-\frac{4(\sin^2X)q^n}{(1+q^n)^2}\right]= \exp\left(2\sum_{r\geq1}\frac{\mathbf{A}_{2r-1}(q)(-4X^2)^r}{(2r)!} \right), \end{align*} $$
as well as
 $$ \begin{align*} &\prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^{2n-1}}{(1-q^{2n-1})^2}\right] =\prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^n}{(1-q^n)^2}\right] \cdot \prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^{2n}}{(1-q^{2n})^2}\right]^{-1} \\ &\quad=\exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)(-16X^2)^r}{(2r)!} \right)\exp\left(2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q^2)(-16X^2)^r}{(2r)!} \right). \end{align*} $$
$$ \begin{align*} &\prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^{2n-1}}{(1-q^{2n-1})^2}\right] =\prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^n}{(1-q^n)^2}\right] \cdot \prod_{n\geq1}\left[1+\frac{4(\sin^22X)q^{2n}}{(1-q^{2n})^2}\right]^{-1} \\ &\quad=\exp\left(-2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)(-16X^2)^r}{(2r)!} \right)\exp\left(2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q^2)(-16X^2)^r}{(2r)!} \right). \end{align*} $$
Therefore, combining these two expressions with (3.9), Theorem 3.4 (2) gives
 $$ \begin{align*} &\sum_{t\geq0} (-1)^t V_{2t}(q) \cdot \frac{X^{2t}}{(2t)!} \\ &\hskip.7in = \cos X\cdot \exp\left( -2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4(2X)^2)^r\right) \exp\left( 2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r\right). \end{align*} $$
$$ \begin{align*} &\sum_{t\geq0} (-1)^t V_{2t}(q) \cdot \frac{X^{2t}}{(2t)!} \\ &\hskip.7in = \cos X\cdot \exp\left( -2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4(2X)^2)^r\right) \exp\left( 2\sum_{r\geq1}\frac{\mathbf{S}_{2r-1}(q)}{(2r)!} (-4X^2)^r\right). \end{align*} $$
As in the proof of (1), we recognize generating functions for Pólya’s cycle index polynomials. Namely, by applying (3.1) and Lemma 3.1 we obtain
 $$ \begin{align*} \sum_{t\geq0} \frac{(-1)^t V_{2t}(q) X^{2t}}{(2t)!} &=\cos X\cdot \frac{2X}{\sin(2X)} \cdot \exp\left(\sum_{k\geq1}\frac{4^kB_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ & \quad\times \frac{\sin X}{X} \cdot \exp\left(\sum_{k\geq1}\frac{-B_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ &=\exp\left(\sum_{k\geq1}\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ &=\sum_{t\geq0}\left(\sum_{\lambda\vdash t}\prod_{k=1}^t\frac1{m_k!}\left(\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{(2{\color{black}k}) (2k)!}\right)^{m_k}\right) (-4X^2)^t. \end{align*} $$
$$ \begin{align*} \sum_{t\geq0} \frac{(-1)^t V_{2t}(q) X^{2t}}{(2t)!} &=\cos X\cdot \frac{2X}{\sin(2X)} \cdot \exp\left(\sum_{k\geq1}\frac{4^kB_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ & \quad\times \frac{\sin X}{X} \cdot \exp\left(\sum_{k\geq1}\frac{-B_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ &=\exp\left(\sum_{k\geq1}\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{2\, (2k)!}\frac{(-4X^2)^k}k\right) \\ &=\sum_{t\geq0}\left(\sum_{\lambda\vdash t}\prod_{k=1}^t\frac1{m_k!}\left(\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{(2{\color{black}k}) (2k)!}\right)^{m_k}\right) (-4X^2)^t. \end{align*} $$
By comparing the coefficients of
![]() $X^{2t}$
, we deduce that
$X^{2t}$
, we deduce that
 $$ \begin{align*}V_{2t}(q)=4^t\, (2t)! \sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{(2{\color{black}k}) (2k)!}\right)^{m_k}, \end{align*} $$
$$ \begin{align*}V_{2t}(q)=4^t\, (2t)! \sum_{\lambda\vdash t} \prod_{k=1}^t \frac1{m_k!} \left(\frac{(4^k-1)B_{2k}\cdot E_{2k}(q)}{(2{\color{black}k}) (2k)!}\right)^{m_k}, \end{align*} $$
which thanks to (1.8) completes the proof of Theorem 1.3 (2).
Acknowledgment
The authors thank the referee, George Andrews and Wei-Lun Tsai for comments on earlier drafts of this paper. The second author thanks the Thomas Jefferson Fund and the NSF (DMS-2002265 and DMS-2055118). The third author is grateful for the support of a Fulbright Nehru Postdoctoral Fellowship.
Data availability statement
Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.