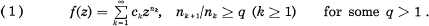Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gnuschke, D.
and
Pommerenke, Ch.
1984.
On annular functions with hadamard gaps.
Complex Variables, Theory and Application: An International Journal,
Vol. 3,
Issue. 1-3,
p.
125.
Pommerenke, Ch.
1984.
Some examples of power series with non-Hadamard gaps.
Mathematische Zeitschrift,
Vol. 187,
Issue. 2,
p.
165.
Steele, J.Michael
1987.
Sharper Wiman inequality for entire functions with rapidly oscillating coefficients.
Journal of Mathematical Analysis and Applications,
Vol. 123,
Issue. 2,
p.
550.
Gnuschke-Hauschild, D.
and
Pommerenke, Ch
1987.
On dominance in hadamard gap series.
Complex Variables, Theory and Application: An International Journal,
Vol. 9,
Issue. 2-3,
p.
189.
1989.
Subharmonic Functions.
p.
865.
Koh, Ngin-Tee
2011.
Lacunary series and Tauberian-type hypotheses.
Archiv der Mathematik,
Vol. 97,
Issue. 2,
p.
179.
Dong, Xin-Han
Lau, Ka-Sing
and
Liu, Jing-Cheng
2013.
Cantor boundary behavior of analytic functions.
Advances in Mathematics,
Vol. 232,
Issue. 1,
p.
543.
Kakaroumpas, Spyridon
and
Soler i Gibert, Odí
2024.
Preimages under linear combinations of iterates of finite Blaschke products.
Analysis and Mathematical Physics,
Vol. 14,
Issue. 3,