ARITHMETIC STRUCTURES FOR DIFFERENTIAL OPERATORS ON FORMAL SCHEMES

Abstract

Let $\mathfrak{o}$ be a complete discrete valuation ring of mixed characteristic $(0,p)$ and $\mathfrak{X}_{0}$ a smooth formal $\mathfrak{o}$-scheme. Let 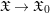$\mathfrak{X}\rightarrow \mathfrak{X}_{0}$ be an admissible blow-up. In the first part, we introduce sheaves of differential operators $\mathscr{D}_{\mathfrak{X},k}^{\dagger }$ on $\mathfrak{X}$, for every sufficiently large positive integer $k$, generalizing Berthelot’s arithmetic differential operators on the smooth formal scheme $\mathfrak{X}_{0}$. The coherence of these sheaves and several other basic properties are proven. In the second part, we study the projective limit sheaf 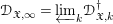$\mathscr{D}_{\mathfrak{X},\infty }=\mathop{\varprojlim }\nolimits_{k}\mathscr{D}_{\mathfrak{X},k}^{\dagger }$ and introduce its abelian category of coadmissible modules. 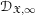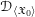The inductive limit of the sheaves $\mathscr{D}_{\mathfrak{X},\infty }$, over all admissible blow-ups $\mathfrak{X}$, is a sheaf $\mathscr{D}_{\langle \mathfrak{X}_{0}\rangle }$ on the Zariski–Riemann space of $\mathfrak{X}_{0}$, which gives rise to an abelian category of coadmissible modules. Analogues of Theorems A and B are shown to hold in each of these settings, that is, for 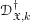$\mathscr{D}_{\mathfrak{X},k}^{\dagger }$, 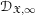$\mathscr{D}_{\mathfrak{X},\infty }$, and 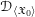$\mathscr{D}_{\langle \mathfrak{X}_{0}\rangle }$.