No CrossRef data available.
Article contents
THE DECOMPOSITION OF PERMUTATION MODULE FOR INFINITE CHEVALLEY GROUPS, II
Part of:
Linear algebraic groups and related topics
Special aspects of infinite or finite groups
Representation theory of groups
Published online by Cambridge University Press: 27 December 2024
Abstract
Let 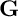 $\mathbf {G}$ be a connected reductive algebraic group over an algebraically closed field
$\mathbf {G}$ be a connected reductive algebraic group over an algebraically closed field  $\Bbbk $ and
$\Bbbk $ and 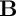 ${\mathbf B}$ be a Borel subgroup of
${\mathbf B}$ be a Borel subgroup of 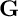 ${\mathbf G}$. In this paper, we completely determine the composition factors of the permutation module
${\mathbf G}$. In this paper, we completely determine the composition factors of the permutation module 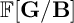 $\mathbb {F}[{\mathbf G}/{\mathbf B}]$ for any field
$\mathbb {F}[{\mathbf G}/{\mathbf B}]$ for any field 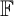 $\mathbb {F}$.
$\mathbb {F}$.
MSC classification
Secondary:
20F18: Nilpotent groups
- Type
- Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Foundation Nagoya Mathematical Journal
References
Carter, R. W., Finite Groups of Lie Type: Conjugacy Classes and Complex Characters, Pure Appl. Math., John Wiley and Sons, New York, 1985.Google Scholar
Chen, X. and Dong, J.,
The permutation module on flag varieties in cross characteristic
, Math. Z. 293 (2019), 475–484.CrossRefGoogle Scholar
Chen, X. and Dong, J.,
The decomposition of permutation module for infinite Chevalley groups
, Sci. China Math 64 (2021), no. 5, 921–930.CrossRefGoogle Scholar
Geck, M.,
Kazhdan–Lusztig cells and the Murphy basis
, Proc. Lond. Math. Soc. 93 (2006), no. 3, 635–665.CrossRefGoogle Scholar
Kazhdan, D. and Lusztig, G.,
Representations of Coxeter groups and Hecke algebras
, Invent. Math. 53 (1979), 165–184.CrossRefGoogle Scholar
Putman, A. and Snowden, A.,
The Steinberg representation is irreducible
, Duke Math. J. 172 (2023), 775–808.CrossRefGoogle Scholar
Serre, J. P., Linear Representations of Finite Groups, GTM, 42, Springer-Verlag, New York–Heidelberg, 1977.CrossRefGoogle Scholar
Xi, N.,
Some infinite dimensional representations of reductive groups with Frobenius maps
, Sci. China Math. 57 (2014), 1109–1120.CrossRefGoogle Scholar
Yang, R.,
Irreducibility of infinite dimensional Steinberg modules of reductive groups with Frobenius maps
, J. Algebra 533 (2019), 17–24.CrossRefGoogle Scholar



