Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-19T09:49:17.675Z Has data issue: false hasContentIssue false
  • English
  • Français

K-Na exchange equilibria between muscovite-paragonite solid solution and hydrothermal chloride solutions

Published online by Cambridge University Press:  05 July 2018

M. L. Pascal  and
J. Roux
M. L. Pascal
Affiliation:
CNRS-CRSCM, 1A rue de la Férollerie, 45045 Orléans cedex, France
J. Roux
Affiliation:
CNRS-CRSCM, 1A rue de la Férollerie, 45045 Orléans cedex, France
Get access Rights & Permissions [Opens in a new window]

Abstract

Three ion exchange equilibrium isotherms between muscovite-paragonite solid solution and 2-molal KCl-NaCl aqueous solutions have been studied at (1) 420°C 1 kbar, (2) 420°C, 2 kbar, and (3) 550°C, 2 kbar. The ΔG°(Joules) ± 2σ of the ion exchange reaction are: ΔG° (1) = −17 259±686, ΔG° (2) = −18 268 ± 560, ΔG° (3) = −16018 ± 336. The excess mixing parameters (‘subregular solution’) of the solid solution (at 1 bar) have been calculated:

The corresponding binodal compositions are (muscovite mol fraction): 12–56% at 420 °C, 1 bar and 15–51% at 550 °C 1 bar. The compositions of micas in equilibrium with perthites (high structural state) at 400, 500, 600 °C and 2 kbar are respectively: Xmus = 91, 86, and 82%.

The mixing properties of the solution were estimated using the speciation of two molal chloride solutions calculated from the dissociation constants of NaCl and KCl in aqueous solution. Although NaCl appears to be substantially more dissociated than KCl, the resulting excess free energy of mixing of the hydrothermal (Na,K)Cl solution was found less than 500 J at temperatures above 400 °C and pressures up to 2 kbar.

The difference in Gibbs free energy of formation (from the elements at 25 °C, 1 bar) between NaCl and KCl in two molal aqueous solutions is proposed:

Keywords

muscoviteparagoniteion exchangechloride solutions
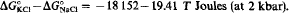
Type
Research Article
Information
Mineralogical Magazine , Volume 49 , Issue 353 , September 1985 , pp. 515 - 521
Copyright
Copyright © The Mineralogical Society of Great Britain and Ireland 1985

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barnes, H. L., and Ernst, W. G. (1963) Am. J. Sci. 261, 129-50.CrossRefGoogle Scholar
Chatterjee, N. D., and Froese, E. (1975) Am. Mineral. 60, 985-93.Google Scholar
Delbove, F., and Sabatier, G. (1974) Bull. Soc.fr. Mineral. Cristallogr. 97, 152-8.Google Scholar
Eugster, H. P., Albee, A. L., Bence, A. E., Thompson, J. B., and Waldbaum, D. R. (1972) J. Petrol. 13, 147-79.CrossRefGoogle Scholar
Grambling, J. A. (1984) Am. Mineral. 69, 79-87.Google Scholar
Gunter, W. D., and Eugster, H. P. (1980) Contrib. Mineral. Petrol. 75, 235-50.CrossRefGoogle Scholar
Helgeson, H. C. (1981) In Chemistry and geochemistry of solutions at high temperatures and pressures (Rickard, D. T. and Wickman, F. E., eds.), 132-74, Pergamon.Google Scholar
Helgeson, H. C., Delany, J. M., Nesbitt, H. W., and Bird, D. K. (1978) Am. J. Sci. 278A, 1–229.Google Scholar
Iiyama, J. T. (1970) C.R. Acad. Sc. Paris, 271, 1925-7.Google Scholar
Lagache, M., and Weisbrod, A. (1977) Contrib. Mineral. Petrol. 62, 77-101.CrossRefGoogle Scholar
Luth, W. C. (1972) In The feldspars (McKenzie, and Zussman, , eds.), 249-96, Manchester University Press.Google Scholar
Orville, P. M. (1972) Am. J. Sci. 272, 234-72.CrossRefGoogle Scholar
Pascal, M. L., and Roux, J. (1982) Geochim. Cosmochim. Acta, 46, 331-7.CrossRefGoogle Scholar
Prigogine, I., and Defay, R. (1954) Chemical thermodynamics, Longmans, London, 543 pp.Google Scholar
Quist, A. M., and Marshall, W. (1968a) J. Phys. Chem. 72, 684–703.CrossRefGoogle Scholar
Quist, A. M., and Marshall, W. (1968b) Ibid. 72, 1536-44.CrossRefGoogle Scholar
Ritzert, G., and Franck, E. U. (1968) Bet Bunsenges Phys. Chem. 72, 798-808.Google Scholar
Schulien, S. (1979) Contrib. Mineral. Petrol. 74, 85-93.CrossRefGoogle Scholar
Schulien, S., Friedrichsen, H., and Hellner, E. (1970) Neues Jahrb. Mineral. Mh. 1417.Google Scholar
Wood, S. A., Crerar, D. A., Brantley, S. L., and Borcsik, M. (1984) Am. J. Sci. 284, 668-705.CrossRefGoogle Scholar