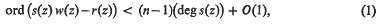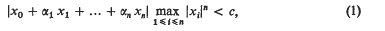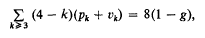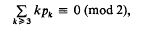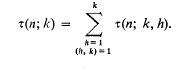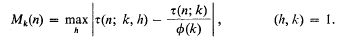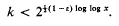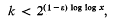Research Article
The convex generation of convex Borel sets in Banach spaces
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 1-3
-
- Article
- Export citation
An effective lower bound on the “Diophantine approximation” of algebraic functions by rational functions
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 4-15
-
- Article
- Export citation
Diophantine approximation of linear forms over an algebraic number field
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 16-23
-
- Article
- Export citation
Bochner's theorem and mean-field electrodynamics
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 24-33
-
- Article
- Export citation
Bending of a thin semi-circular plate elastically restrained along the circular edge and clamped along the diameter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 34-46
-
- Article
- Export citation
The flow in the neighbourhood of the rim of a finite rotating disc
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 47-52
-
- Article
- Export citation
Isomorphic Koszul complexes
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 53-58
-
- Article
- Export citation
On the K-theory of the quaternion group
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 59-62
-
- Article
- Export citation
A theorem on the structure of cell–decompositions of orientable 2–manifolds
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 63-82
-
- Article
- Export citation
An application of a reduction method of R. Rado to the study of common transversals
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 83-86
-
- Article
- Export citation
The divisors of p-1
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 87-97
-
- Article
- Export citation
On self-induced separation II
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 98-108
-
- Article
- Export citation
Difference covers which have small k-sums for any k
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 109-118
-
- Article
- Export citation
Front matter
MTK volume 20 issue 1 Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f2
-
- Article
-
- You have access
- Export citation