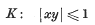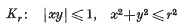Various attempts have been made to identify the slip lines or Lüder lines which are observed in solids with surfaces of discontinuity or characteristic surfaces associated with solutions of equations of plasticity. Results such as those obtained in [1], together with the observed fact that such lines occur in a variety of types of experiments, indicate that, for two well-known theories of plasticity, characteristic surfaces fail to exist in situations in which such lines are observed. This can come about in two ways, one being that real characteristic directions do not exist, the other being that they do, but that the characteristic surface elements do not unite to form surfaces. The latter situation seems to arise from the fact that, even in truly three-dimensional problems, the equations considered admit only a finite number of characteristic directions. Results such as those given in [2] indicate that, if real characteristic directions do not always exist, there is some doubt as to whether one can identify such lines with surfaces of discontinuity. Another point to be considered is the ease with which solutions may be obtained. For equations possessing real characteristics, the method of characteristics is a powerful tool to use in solving two-dimensional problems. It is noted in [3], Ch. X, that, in axially symmetric problems, one cannot use this method to obtain solutions of the von Mises equations. In treating such problems, it may be easier to use equations which appear to be more complicated, but which possess real characteristics. These facts suggest that plasticity equations which always possess real characteristic directions are to be preferred to those which do not. Some workers in plasticity appreciate this, as is indicated by remarks made in [4]. However, no one has taken a rather general theory of plasticity, such as the theory of perfectly plastic solids, and attempted to determine which of the equations included in it have this property. We made an unsuccessful attempt to do so for a theory which is roughly equivalent. The purpose of this paper is to present this theory, to indicate the basic mathematical problem involved, and to record a partial solution of it.

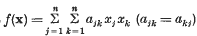 be a positive definite quadratic form in
be a positive definite quadratic form in