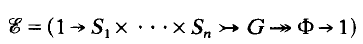Research Article
Mean values of character sums II
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 1-7
-
- Article
- Export citation
An improvement to the Minkowski-Hiawka bound for packing superballs
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 8-18
-
- Article
- Export citation
Automorphism groups of sandwich semigroups
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 19-28
-
- Article
- Export citation
Enhancement of temperature decay by controlling conditions at the boundary
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 29-38
-
- Article
- Export citation
Existence and stability in convection in porous media
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 39-52
-
- Article
- Export citation
A gap condition for the zeroes of certain polynomials in Kaplan classes K(α, β)
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 53-63
-
- Article
- Export citation
Effective properties in compact sets of Borel functions
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 64-68
-
- Article
- Export citation
Families of plane curves having translates in a set of measure zero
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 69-76
-
- Article
- Export citation
Integral inequalities for ovaloids in Euclidean space
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 77-81
-
- Article
- Export citation
On surface Bieberbach groups
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 82-85
-
- Article
- Export citation
Complete breakdown of an unsteady interactive boundary layer (over a surface distortion or in a liquid layer)
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 86-100
-
- Article
- Export citation
Topological spaces with dense subspaces that are homeomorphic to complete metric spaces and the classification of C(K) Banach spaces
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 101-107
-
- Article
- Export citation
Book Reviews
Book Reviews - J. Aczél. A short course on Functional Equations. D. Reidel. 1987, 169 pp., £35. - L. V. Ahlfors. Lectures on quasiconformal mappings. Reprint of 1966 edition. Wadsworth. 1987, 146 pp., $24·95. - J. S. R. Chisholm and A. K. Common, Editors. Clifford Algebras and their applications in Mathematical Physics, NATO-ASI series. D. Reidel. 1986, 592 pp., £74·50. - L. Collatz. Differential Equations, an Introduction with Applications. J. Wiley & Sons. 1986, 372 pp., £11·95. - P. G. N. Digby and J. C. Gower. Ordination and Classification. Presses de l'Université de Montréal. 1986, 87 pp., Canadian $16. - D. G. Duffy. Solutions of Partial Differential Equations. J. Wiley & Sons. 1986, 542 pp., £23.85. - D. D. Holm, J. E. Marsden and T. S. Ratiu. Hamiltonian structure and Lyapunov stability for ideal continuum dynamics. Presses de l'Université de Montréal. 1986, 208 pp., Canadain $20. - A. Howard and P.-M. Wong, Editors. Contributions to several complex variables. Friedr. Vieweg & Sohn. 1986, 353 pp., £23·70. - J. A. John. Cyclic Design. Chapman & Hall. 1987, 232 pp, £16. - R. Kaya, P. Plaumann and K. Strambach, Editors. Rings and Geometry, NATO-ASI series. D. Reidel. 1985, 567 pp., £56·95. - G. G. Koch and P. B. Imrey. Analysis of Categorical Data. Presses de l'Université de Montréal. 1985, 288 pp., Canadian $22. - E. Kunz. Kähler Differentials. J. Wiley & Sons (for F. Vieweg & Sohn). 1986, 402 pp., £26·50. - W. Linde. Probability in Banach Spaces—Stable and Infinitely Divisible Distributions. J. Wiley & Sons. 1986, 195 pp., £16·50. - B. F. J. Manly. Multivariate Statistical Methods: A Primer. Chapman & Hall. 1986, 159 pp., hardback £15, paperback £6·95. - M. Minoux. Mathematical Programming. J. Wiley & Sons. 1986, 489 pp., £34.95. - J. Nečas. Introduction to the theory of non-linear elliptic equations. J. Wiley & Sons. 1986, 164 pp., £14·95. - H.-O. Peitgen and P. H. Richter. The Beauty of Fractals. Springer. 1986, 199 pp., DM78. - A. Pressley and G. Segal. Loop Groups. Oxford University Press. 1986, 318 pp., £40. - G.-C. Rota, Editor. Probability, Statistical Mechanics, and Number Theory: A volume dedicted to Mark Kac. Advances in Mathematics: supplementary studies, Vol. 9. Academic Press, 1986, 194 pp., £49.50. - J. D. H. Smith. Representation theory of infinite groups and finite quasigroups. Presses de l'Université de Montréal. 1986, 132 pp., Canadian $18. - E. J. Snell. Applied Statistics, a handbook of BMDP analyses. Chapman & Hall. 1987, 171 pp., paperback £7·95. - R. P. Stanley. Enumerative Combinatorics I. Wadsworth & Brooks/Cole. 1986, 306 pp. £42.95. - I. Stewart. The problems of mathematics. Oxford University Press. 1987, 257 pp., hard cover £17·50, paperback £5·95. - D. J. Struick, Editor. A source book in Mathematics, 1200–1800. Princeton University Press. 1986, 427 pp., £8·40. - M. Suzuki. Group Theory II. Springer. 1986, 621 pp., DM 268. - Ā Szendrei. Clones in universal algebra. Presses de l'Université de Montréal, 1986, 166 pp., Canadian $19. - E. C. Titchmarsh. The theory of the Riemann Zeta-function. 2nd Edition revised by D. R. Heath-Brown. Oxford University Press. 1986, 412 pp, £25. - P. Winternitz. Systéms dynamiques non-lineareaires: intégrabilité et comportement qualitatif. Presses de l'Université de Montréal. 1986, 339 pp., Canadian $30.
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 108-113
-
- Article
- Export citation
Front matter
MTK volume 34 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f4
-
- Article
-
- You have access
- Export citation



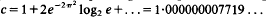 for σ = 2 (the classical sphere packing problem), worse than but surprisingly close to the Minkowski-Hlawka bound, and
for σ = 2 (the classical sphere packing problem), worse than but surprisingly close to the Minkowski-Hlawka bound, and 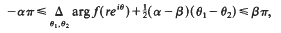
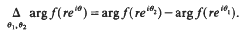
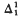 sequence of continuous functions on some recursively presentable Polish space, such that any pointwise cluster point of (
sequence of continuous functions on some recursively presentable Polish space, such that any pointwise cluster point of (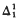 -subsequence of (
-subsequence of (