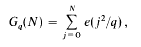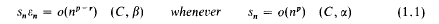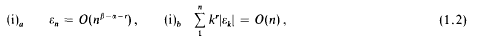Research Article
The graphs of exponential sums
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 153-163
-
- Article
- Export citation
Small solutions of congruences
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 164-188
-
- Article
- Export citation
K-analytic sets
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 189-221
-
- Article
- Export citation
On a free boundary problem for the equations of tidal motions
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 222-254
-
- Article
- Export citation
Convergence and summability factors in a sequence (II)
- Part of:
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 255-273
-
- Article
- Export citation
The toroidal analogue to Eberhard's theorem
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 274-290
-
- Article
- Export citation
Aspherical manifolds with the Q-homology of a sphere
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 291-294
-
- Article
- Export citation
Back matter
MTK volume 30 issue 2 Back matter
-
- Published online by Cambridge University Press:
- 26 February 2010, p. b1
-
- Article
-
- You have access
- Export citation
Front matter
MTK volume 30 issue 2 Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f10
-
- Article
-
- You have access
- Export citation