A new and more geometric proof is obtained of Stanley's lower bounds on the face numbers of centrally symmetric simple polytopes.

In connection with the thesis of Ch. Charitos (1989), T. Hasanis posed the following question.

Several results are proved related to a question of Steinhaus: is there a set E⊂ℝ2 such that the image of E under each rigid motion of IR2 contains exactly one lattice point? Assuming measurability, the analogous question in higher dimensions is answered in the negative, and on the known partial results in the two dimensional case are improved on. Also considered is a related problem involving finite sets of rotations.

We are interested in the distribution of those zeros of the Riemann zeta-function which lie on the critical line ℜs = ½, and the maxima of the function between successive zeros. Our results are to be independent of any unproved hypothesis. Put

In this paper, it is proved that, for any m unit vectors. x1…, xm in any n-dimensional real Hilbert space, there exists a unit vector x0 such that

for any y∈Sn−1. The exact value of the above integral is calculated, and these results used to improve some lower bounds for multilinear forms on real Hilbert spaces. An integral expression is also given for the complex case.

In [4], we investigated the spaces of continuous functions on countable products of compact Hausdorff spaces. Our main object here is to extend the discussion to arbitrary products of compact Hausdorff spaces. We prove the following theorems in Section 3.

A (countably) compact measure is one which is inner regular with respect to a (countably) compact class of sets. This note characterizes compact probability measures in terms of the representation of Boolean homomorphisms of their measure algebras, and shows that the same ideas can be used to give a direct proof of J. Pachl's theorem that any image measure of a countably compact measure is again countably compact.

Questions of Haight and of Weizsäcker are answered in the following result. There exists a measurable function f: (0, + ∞) → {0,1} and two non-empty intervals IFI∞⊂[½,1) such that Σ∞n = 1f(nx) = +∞ for everyx εI∞, and Σ∞n = 1f(nx) >+∞ for almost every xεIf. The function f may be taken to be the characteristic function of an open set E.

Given a Banach space X and a norming subspace Z⊂X*, a geometrical method is introduced to characterize the existence of an equivalent σ(X, Z)-lsc LUR norm on X. A new simple proof of the Theorem of Troyanski: every rotund space with a Kadec norm is LUR renormable, and a generalization of the Moltó, Orihuela and Troyanski characterization of the LUR renormability, are provided without probability arguments. Among other applications, it is shown that a dual Banach space with a w*-Kadec norm admits a dual LUR norm.

In this paper it is proved that, for x sufficiently large, every integer m with

can be written as m = Σ1≤n≤xεn/n, where εi, = 0 or 1.

It is well known that, if p is prime, the multiplicative group (ℤ/pℤ)* of reduced residues is cyclic. A generator is called a primitive root; there are φ(p − 1) of them, where φ is Euler's function. Thus, (φ(p − 1)/(p−1) is the proportion of primitive roots modulo p in (ℤ/pℤ)*. Elliott has proved that φp − 1)/(p − 1) has a limiting distribution function [2], in the sense that

exists for all real numbers u.

Let φ(n) be the Euler function (i.e., φ(n) denotes the number of integers less than n which are relatively prime to n), and define

These functions were extensively studied by several mathematicians. One of the problems investigated concerns their sign changes. We say that a function fx) has a sign change at x = x0 if f(x0 −) f(x0 +) < 0, and f(x) has a sign change on the integer n if (n)f(n+1) < 0. The numbers of sign changes and sign changes on integers of f(x) in the interval [1, T] are denoted by Xf(T) and Nf(T), respectively.

Assuming the abc-conjecture, it is shown that there are only finitely many powerful binomial coefficients with 3≤k≤n/2 in fact, if q2 divides , then . Unconditionally, it is shown that there are N1/2+σ(1) powerful binomial coefficients in the top N rows of Pascal's Triangle.

A long-standing conjecture claims that the Diophantine equation

has finitely many solutions, and, maybe, only those given by

In 1984 Heath-Brown [2] proved the conjecture of Erdős and Mirsky [1], and obtained the following result.

Theorem. There are infinitely many integers n for which d(n+ 1)= d(n). Indeed, for large x, the number of such n≤x is at least of orderx ln-7x.

It is shown that an integral domain R has the property that every pure submodule of a finite direct sum of ideals of R is a summand if and only if R is an h-local Prüfer domain; equivalently, (J + K:I) = (J:I) + (K:I) for all ideals I, J and K of R. These results are extended to submodules of the quotient field of an integral domain.

The existence of global solutions to the discrete coagulation equations is investigated for a class of coagulation rates of the form ai, j = rirj + αi, j with αi, j≤Krirj. In particular, global solutions are shown to exist when the sequence (ri) increases linearly or superlinearly with respect to i. In this case also, the failure of density conservation (indicating the occurrence of the gelation phenomenon) is studied.

This paper treats the reducibility of the quasiperiodic linear differential equations

where A is a constant matrix with multiple eigenvalues, Q(t) is a quasiperiodic matrix with respect to time t, and ε is a small perturbation parameter. Under some non-resonant conditions, rapidly convergent methods prove that, for most sufficiently small ε, the differential equations are reducible to a constant coefficient differential equation by means of a quasiperiodic change of variables with the same frequencies as Q(t).

Consider the equation

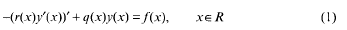
where 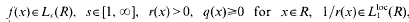, . The inversion problem for (1) is called regular in Lp if, uniformly in p∈[1, ∞] for any f(x)∈ Lp(R), equation (1) has a unique solution y(x)∈ Lp(R) of the form

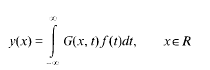
with . Here G(x, t) is the Green function corresponding to (1) and c is an absolute constant. For a given s∈[l, ∞], necessary and sufficient conditions are obtained for assertions (2) and (3) to hold simultaneously:

(2) the inversion problem for (1) is regular in Lp;

(3) for any f(x)∈LS(R).