Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T17:43:49.340Z Has data issue: false hasContentIssue false
  • English
  • Français

PERFECT POWERS THAT ARE SUMS OF CONSECUTIVE CUBES

Part of: Diophantine equations Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  05 October 2016

Michael A. Bennett ,
Vandita Patel  and
Samir Siksek
Michael A. Bennett
Affiliation:
Department of Mathematics, University of British Columbia, VancouverB.C., V6T 1Z2, Canada email [email protected]
Vandita Patel
Affiliation:
Mathematics Institute, University of Warwick, Coventry CV4 7AL, U.K. email [email protected]
Samir Siksek
Affiliation:
Mathematics Institute, University of Warwick, Coventry CV4 7AL, U.K. email [email protected]
Get access

Abstract

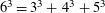Euler noted the relation $6^{3}\,=\,3^{3}+4^{3}+5^{3}$ and asked for other instances of cubes that are sums of consecutive cubes. Similar problems have been studied by Cunningham, Catalan, Gennochi, Lucas, Pagliani, Cassels, Uchiyama, Stroeker and Zhongfeng Zhang. In particular, Stroeker determined all squares that can be written as a sum of at most 50 consecutive cubes. We generalize Stroeker’s work by determining all perfect powers that are sums of at most 50 consecutive cubes. Our methods include descent, linear forms in two logarithms and Frey–Hellegouarch curves.

MSC classification

Primary: 11D61: Exponential equations
Secondary: 11D41: Higher degree equations; Fermat's equation 11F80: Galois representations 11F11: Holomorphic modular forms of integral weight
Type
Research Article
Information
Mathematika , Volume 63 , Issue 1 , 2017 , pp. 230 - 249
Copyright
Copyright © University College London 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bosma, W., Cannon, J. and Playoust, C., The Magma algebra system I: the user language. J. Symbolic Comput. 24 1997, 235–265 (see also http://magma.maths.usyd.edu.au/magma/).CrossRefGoogle Scholar
Breuil, C., Conrad, B., Diamond, F. and Taylor, R., On the modularity of elliptic curves over ℚ: wild 3-adic exercises. J. Amer. Math. Soc. 14 2001, 843–939.Google Scholar
Cassels, J. W. S., A Diophantine equation. Glasg. Math. J. 27 1985, 11–88.Google Scholar
Dickson, L. E., History of the Theory of Numbers, Vol. II, Chelsea (New York, 1971).Google Scholar
Euler, L., Vollständige Anleitung zur Algebra, Vol. 2, Kayserliche Akademie der Wissenschaften (St. Petersburg, 1770).Google Scholar
Kraus, A., Majorations effectives pour l’équation de Fermat généralisée. Canad. J. Math. 49 1997, 1139–1161.Google Scholar
Laurent, M., Linear forms in two logarithms and interpolation determinants. II. Acta Arith. 133 2008, 325–348.Google Scholar
Lucas, E., Researches sur l’analyse indéterminée et l’arithmétique de Diophante, Moulin (1873).Google Scholar
Mazur, B., Rational isogenies of prime degree. Invent. Math. 44 1978, 129–162.CrossRefGoogle Scholar
Nagell, T., Des équations indéterminées x 2 + x + 1 = y n et x 2 + x + 1 = 3y n . Norsk Mat. Forenings Skr. 1(2) 1921, 1–14.Google Scholar
Pagliani, C., Solution du problème d’analyse indéterminée énoncé à la page 212 du présent volume. Ann. Math. Appl. 20 1829–1830, 382–384.Google Scholar
Ribet, K., On modular representations of Gal(ℚ/ℚ) arising from modular forms. Invent. Math. 100 1990, 431–476.Google Scholar
Siksek, S., The modular approach to Diophantine equations. In Explicit Methods in Number Theory: Rational Points and Diophantine Equations (Panoramas et synthèses 36 ), Société Mathématique de France (Paris, 2012), 151–179.Google Scholar
Silverman, J. H., Arithmetic of Elliptic Curves (Graduate Texts in Mathematics 106 ), 2nd edn., Springer (New York, 2008).Google Scholar
Smart, N. P., The Algorithmic Resolution of Diophantine Equations (London Mathematical Society Student Texts 41 ), Cambridge University Press (Cambridge, 1997).Google Scholar
Stroeker, R. J., On the sum of consecutive cubes being a square. Compos. Math. 97 1995, 295–307.Google Scholar
Uchiyama, S., On a Diophantine equation. Proc. Japan Acad. Ser. A Math. Sci. 55(9) 1979, 367–369.Google Scholar
Wiles, A., Modular elliptic curves and Fermat’s Last Theorem. Ann. of Math. (2) 141 1995, 443–551.CrossRefGoogle Scholar
Zhang, Z., On the Diophantine equation (x - 1) k + x k + (x + 1) k = y n . Publ. Math. Debrecen 85 2014, 93–100.Google Scholar