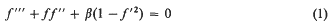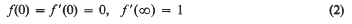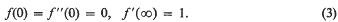Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Utz, W.R
1978.
Existence of solutions of a generalized Blasius equation.
Journal of Mathematical Analysis and Applications,
Vol. 66,
Issue. 1,
p.
55.
Schwierz, K. P.
1979.
Zur Existenz und Eindeutigkeit der Görtler‐Funktionen II. Das Existenzproblem.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 59,
Issue. 12,
p.
685.
Abu-Sitta, A.M.M.
1994.
A note on a certain boundary-layer equation.
Applied Mathematics and Computation,
Vol. 64,
Issue. 1,
p.
73.
El Amrani, Jamal
Amtout, Tarik
Er-Riani, Mustapha
Lahrouz, Aadil
and
Settati, Adel
2024.
On the positive self-similar solutions of the boundary-layer wedge flow problem of a power-law fluid.
Journal of Engineering Mathematics,
Vol. 148,
Issue. 1,