Article contents
ON LINEAR COMBINATIONS OF UNITS WITH BOUNDED COEFFICIENTS
Published online by Cambridge University Press: 31 May 2011
Abstract
Starting with a paper of Jacobson from the 1960s, many authors became interested in characterizing all algebraic number fields in which each integer is the sum of pairwise distinct units. Although there exist many partial results for number fields of low degree, a full characterization of these number fields is still not available. Narkiewicz and Jarden posed an analogous question for sums of units that are not necessarily distinct. In this paper we propose a generalization of these problems. In particular, for a given rational integer n we consider the following problem. Characterize all number fields for which every integer is a linear combination 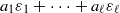 of finitely many units εi in a way that the coefficients ai∈ℕ are bounded by n. The paper gives several partial results on this problem. In our proofs we exploit the fact that these representations are related to symmetric beta expansions with respect to Pisot bases.
of finitely many units εi in a way that the coefficients ai∈ℕ are bounded by n. The paper gives several partial results on this problem. In our proofs we exploit the fact that these representations are related to symmetric beta expansions with respect to Pisot bases.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2011
References
- 4
- Cited by


