Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T11:59:12.797Z Has data issue: false hasContentIssue false
  • English
  • Français

On a problem of Barban and Vehov

Published online by Cambridge University Press:  26 February 2010

Matti Jutila
Matti Jutila
Affiliation:
Department of Mathematics, University of Turku, Turku, Finland
Get access

Extract

Barban and Vehov posed in [1] the problem of minimizing the quadratic form

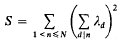
under the conditions

where 1 ≤ z1 < z2 and μ(n) is the Möbius function. They also commented on the connections of this problem with zero-density estimates of L-functions near the lines δ = 1 and ½, and with Linnik's prime number theorem. This program was carried out some years later by other authors, in the first place by Selberg [10], and later by Motohashi ([7]–[9]), Graham [2] and the present author ([4], [5]).

Type
Research Article
Information
Mathematika , Volume 26 , Issue 1 , June 1979 , pp. 62 - 71
Copyright
Copyright © University College London 1979

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Barban, M. B. and Vehov, P. P.. “On an extremal problem” (in Russian), Trudy Mosk. Mat. Obsc., 18 (1968), 83–90. See also: Trans. Moscow Math. Soc, 18 (1968), 91–99.Google Scholar
2.Graham, S.. Applications of sieve methods. Dissertation (Univ. of Michigan, Ann Arbor, 1977).Google Scholar
3.Graham, S.. “An asymptotic estimate related to Selberg's Sieve”, J. Number Theory, 10 (1978), 83–94.Google Scholar
4.Jutila, M.. “On Linnik's constant”, Math. Scand., 41 (1977), 45–62.Google Scholar
5.Jutila, M.. “Zeros of the zeta-function near the critical line” (to appear).Google Scholar
6.Motohashi, Y.. “On a problem in the theory of sieve methods” (in Japanese), Res. Inst. Math. Sci. Kyoto Univ. Kōkyuroko, 222 (1974), 9–50.Google Scholar
7.Motohashi, Y.. “On a density theorem of Linnik”, Proc. Japan Acad., 51 (1975), 815–817.CrossRefGoogle Scholar
8.Motohashi, Y.. “On the Deuring-Heilbronn phenomenon I–II, Proc. Japan Acad., 53 (1977), 1–2 and. 25-27.Google Scholar
9.Motohashi, Y.. “Primes in arithmetic progressions”, lnventiones Math., 44 (1978), 163–178.CrossRefGoogle Scholar
10.Selberg, A.. “Remarks on sieves”, Proc. of the 1972 Number Theory Conference (Boulder), 205-216.Google Scholar
11.Titchmarsh, E. C.. The theory of the Riemann zeta-function (Clarendon Press, Oxford, 1951).Google Scholar