A note on Diophantine approximation (II)

Extract

In 1956 Cassels proved the following result, which generalized a theorem of Marshall Hall on continued fractions. Let λ₁ …, λ_r be any real numbers. Then there exists a real number α such that

for all integers u > 0 and for q = 1,…,r, where C = C(r) > 0. Thus all the numbers α+ λ₁, …, α+ λ_r are badly approximable by rational numbers, which is equivalent to saying that the partial quotients in their continued fractions are bounded. In a previous paper I extended Cassels's result to simultaneous approximation. In the simplest case—that of simultaneous approximation to pairs of numbers—I proved that for any real λ₁, …, λ_r and μ₁, …, μ_r there exist α, β such that

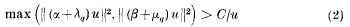
for all integers u > 0 and for q=1,…, r, where again C = C(r) > 0. Both the construction of Cassels and my extension of it to more dimensions allow one to introduce an infinity of arbitrary choices, and consequently the set of α for (1) and the set of α, β for (2) may be made to have the cardinal of the continuum.