Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T16:20:53.787Z Has data issue: false hasContentIssue false
  • English
  • Français

New Radon–Nikodym ideals

Part of: Set theory

Published online by Cambridge University Press:  26 February 2010

Vladimir Kanovei  and
Michael Reeken
Vladimir Kanovei
Affiliation:
Moscow Center for Continuous Mathematical Education, Moscow 121002, Russia. E-mail: [email protected]
Michael Reeken
Affiliation:
Fachbereich Mathematik, Universität Wuppertal, D-42097 Wuppertal, Germany. E-mail: [email protected]
Get access

Abstract

Farah recently proved that many Borel P-ideals. 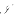on 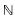satisfy the following requirement: any measurable homomorphism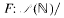 has a continuous lifting 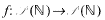which is a homomorphism itself. Ideals having such a property were called Radon–Nikodym (RN) ideals. Answering some Farah's questions, it is proved that many non-P ideals, including, for instance, Fin ⊗ Fin, are Radon–Nikodym. To prove this result, another property of ideals called the Fubini property, is introduced, which implies RN and is stable under some important transformations of ideals.

MSC classification

Secondary: 03E15: Descriptive set theory
Type
Research Article
Information
Mathematika , Volume 47 , Issue 1-2 , December 2000 , pp. 219 - 227
Copyright
Copyright © University College London 2000

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Farah, I.. Completely additive liftings. Bull. Symb. Logic 4 (1998), 37–54.CrossRefGoogle Scholar
2.Farah, I.. Liftings of homoraorphisms between quotient structures and Ulam stability. In eds. Buss, S.et al, Logic Colloquium 98 Lecture Notes in Logic, 13 (1998), 173–196.Google Scholar
3.Farah, I.. Analytic quotients: theory of liftings for quotients over analytic ideals on the integers. Memoirs Amer. Math. Soc., 148 (2000), 177 pp..CrossRefGoogle Scholar
4.Farah, I.. Approximate homomorphisms II: Group homomorphisms. Combinatorica, 20 (2000), 37–60.CrossRefGoogle Scholar
5.Kanovei, V. and Reeken, M.. On Borel automorphisms of the reals modulo a countable group. Math. Logic Quarterly, 46 (2000), 377–384.3.0.CO;2-9>CrossRefGoogle Scholar
6.Kanovei, V. and Reeken, M.. On Ulam's problem of stability of approximate homomorphisms. Proc. Moscow Sleklov Math. Inst. MIAN, 231 (2000), 249–283.Google Scholar
7.Kechris, A. S.. Classical Descriptive Set Theory, Graduate Texts in Mathematics, 156 (Springer, 1995).CrossRefGoogle Scholar
8.Kechris, A. S.. Rigidity properties of Borel ideals on the integers. Topology and Applications, 85 (1998), 195–205.CrossRefGoogle Scholar
9.Todorcevic, S.. Analytic gaps. Fund. Math., 150 (1996), 55–66.Google Scholar
10.Velickovic, B.. Definable automorphisms of Proc. Amer. Math. Soc. 96 (1986), pp. 130–135.Google Scholar