Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-28T05:26:27.057Z Has data issue: false hasContentIssue false
  • English
  • Français

Homogeneous quadratic equations

Published online by Cambridge University Press:  26 February 2010

H. Davenport
Get access

Extract

Let

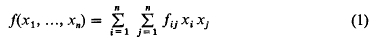
be a quadratic form with integral coefficients, and suppose the equation

has a solution in integers x1…, xn, not all 0. It was proved by Cassels [2] that there is such a solution, which satisfies the estimate

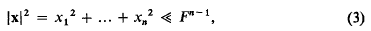
where F = max|fij|. It was later observed by Birch and Davenport [1] that the result can be stated in a slightly more general form. Let

be a quadratic form which assumes only integral values at the points (x1 …, x2) of an n-dimensional lattice Λ of determinant Δ. Suppose there is some point of Λ, other than the origin, at which ø = 0. Then there is such a point for which also

where Φ = max |øij|.

Type
Research Article
Information
Mathematika , Volume 18 , Issue 1 , June 1971 , pp. 1 - 4
Copyright
Copyright © University College London 1971

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Birch, B. J. and Davenport, H., Proc. Camb. Phil. Soc., 54 (1958), 135–138.CrossRefGoogle Scholar
2.Cassels, J. W. S., Proc. Camb. Phil. Soc., 51 (1955), 262–264 and 52 (1956), 604.CrossRefGoogle Scholar
3.Minkowski, H., Geometrie der Zahlen, Kap. 5.Google Scholar