Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hilhorst, H J
Calka, P
and
Schehr, G
2008.
Sylvester’s question and the random acceleration process.
Journal of Statistical Mechanics: Theory and Experiment,
Vol. 2008,
Issue. 10,
p.
P10010.
Balogh, József
González-Aguilar, Hernán
and
Salazar, Gelasio
2013.
Large convex holes in random point sets.
Computational Geometry,
Vol. 46,
Issue. 6,
p.
725.
Dolgikh, V. V.
Senderskiy,, I. V.
Tetz, G. V.
and
Tetz, V. V.
2013.
Extracellular domain of HER2 heterologous expression in bacteria.
The Scientific Notes of the I. P. Pavlov St. Petersburg State Medical University,
Vol. 20,
Issue. 4,
p.
70.
Buchta, Christian
2013.
Exact Formulae for Variances of Functionals of Convex Hulls.
Advances in Applied Probability,
Vol. 45,
Issue. 4,
p.
917.
Reitzner, Matthias
and
Schulte, Matthias
2013.
Central limit theorems for $U$-statistics of Poisson point processes.
The Annals of Probability,
Vol. 41,
Issue. 6,
Buchta, Christian
2013.
Exact Formulae for Variances of Functionals of Convex Hulls.
Advances in Applied Probability,
Vol. 45,
Issue. 4,
p.
917.
Ng, Chi Tim
Lim, Johan
Lee, Kyeong Eun
Yu, Donghyeon
and
Choi, Sujung
2014.
A fast algorithm to sample the number of vertexes and the area of the random convex hull on the unit square.
Computational Statistics,
Vol. 29,
Issue. 5,
p.
1187.
Son, Won
Ng, Chi Tim
and
Lim, Johan
2015.
A New Integral Representation of the Coverage Probability of a Random Convex Hull.
Communications for Statistical Applications and Methods,
Vol. 22,
Issue. 1,
p.
69.
Marckert, Jean-François
2017.
The probability that $n$ random points in a disk are in convex position.
Brazilian Journal of Probability and Statistics,
Vol. 31,
Issue. 2,
Shilat, Yoel
Salti, Moti
and
Henik, Avishai
2021.
Shaping the way from the unknown to the known: The role of convex hull shape in numerical comparisons.
Cognition,
Vol. 217,
Issue. ,
p.
104893.
Gusakova, Anna
and
Thäle, Christoph
2021.
ON RANDOM CONVEX CHAINS, ORTHOGONAL POLYNOMIALS, PF SEQUENCES AND PROBABILISTIC LIMIT THEOREMS.
Mathematika,
Vol. 67,
Issue. 2,
p.
434.
Godrèche, Claude
and
Luck, Jean-Marc
2024.
On sequences of convex records in the plane.
Journal of Statistical Mechanics: Theory and Experiment,
Vol. 2024,
Issue. 9,
p.
093208.
 be the probability that the convex chain consists – apart from the points (0, 1) and (1, 0) – of exactly k of the points P1,…,Pn. Bárány, Rote, Steiger, and Zhang [3] proved that
be the probability that the convex chain consists – apart from the points (0, 1) and (1, 0) – of exactly k of the points P1,…,Pn. Bárány, Rote, Steiger, and Zhang [3] proved that 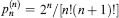 . The values of
. The values of  are determined for k = 1,…,n − 1, and thus the distribution of the number of vertices of a random convex chain is obtained. Knowing this distribution provides the key to the answer of some long-standing questions in geometrical probability.
are determined for k = 1,…,n − 1, and thus the distribution of the number of vertices of a random convex chain is obtained. Knowing this distribution provides the key to the answer of some long-standing questions in geometrical probability.

