No CrossRef data available.
Published online by Cambridge University Press: 26 February 2010
The key result of this paper proves the existence of functions ρn(h) for which, whenever H is a (Lebesgue) measurable subset of the n-dimensional unit cube In with measure |H| > h and ℛ is a class of subintervals (n-dimensional axis-parallel rectangles) of In that covers H, then there exists an interval R∈ℛ in which the density of H is greater than ρn(h); that is, |H∩R|/|R|>ρn (h) (=(h/2n)2). It is shown how to use this result to find 4 points of a measurable subset of the unit square which are the vertices of an axis-parallel rectangle that has quite large intersection with the original set. Density and covering properties of classes of subsets of ℝn are introduced and investigated. As a consequence, a covering property of the class of intervals of ℝn is obtained: if ℛ is a family of n-dimensional intervals with 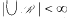 , then there is a finite sequence R1, …, Rm∈ℛ such that
, then there is a finite sequence R1, …, Rm∈ℛ such that 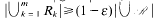 and
and 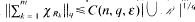 .
.