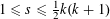Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cellarosi, Francesco
and
Marklof, Jens
2016.
Quadratic Weyl sums, automorphic functions and invariance principles.
Proceedings of the London Mathematical Society,
Vol. 113,
Issue. 6,
p.
775.
Bourgain, Jean
and
Demeter, Ciprian
2017.
Decouplings for curves and hypersurfaces with nonzero Gaussian curvature.
Journal d'Analyse Mathématique,
Vol. 133,
Issue. 1,
p.
279.
Bourgain, Jean
Demeter, Ciprian
and
Guo, Shaoming
2017.
Sharp bounds for the cubic Parsell–Vinogradov system in two dimensions.
Advances in Mathematics,
Vol. 320,
Issue. ,
p.
827.
García, Victor Cuauhtemoc
2018.
The finite Littlewood problem in
$${{\mathbb {F}}}_p$$
F
p
.
The Ramanujan Journal,
Vol. 47,
Issue. 1,
p.
85.
Wooley, Trevor D.
2019.
Nested efficient congruencing and relatives of Vinogradov's mean value theorem.
Proceedings of the London Mathematical Society,
Vol. 118,
Issue. 4,
p.
942.
Guo, Shaoming
and
Zhang, Ruixiang
2019.
On integer solutions of Parsell–Vinogradov systems.
Inventiones mathematicae,
Vol. 218,
Issue. 1,
p.
1.
Kuo, Wentang
Liu, Yu-Ru
and
Zhao, Xiaomei
2019.
The asymptotic estimates and Hasse principle for multidimensional Waring's problem.
Advances in Mathematics,
Vol. 353,
Issue. ,
p.
1.
Brandes, Julia
and
Parsell, Scott T.
2021.
The Hasse principle for diagonal forms restricted to lower-degree hypersurfaces.
Algebra & Number Theory,
Vol. 15,
Issue. 9,
p.
2289.
Heath-Brown, D. R.
2022.
The cubic case of Vinogradov’s mean value
theorem.
Essential Number Theory,
Vol. 1,
Issue. 1,
p.
1.