Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T05:09:29.604Z Has data issue: false hasContentIssue false
  • English
  • Français

Some Abelian results for Dirichlet series

Published online by Cambridge University Press:  26 February 2010

W. E. Briggs
W. E. Briggs
Affiliation:
University of Colorado. University College, London
Get access

Extract

If f(s) is the analytic function defined by the Dirichlet series 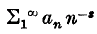and if 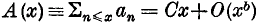where 0 ≤ b < 1, then the series converges for Re s > 1 and f(s) is regular in the half plane Re s > b except for a simple pole with residue C ≠ 0 at a s = 1. Thus f(s) has a Laurent expansion at s = 1 and it has been shown [1] that under these conditions

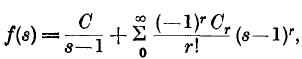
where

Type
Research Article
Information
Mathematika , Volume 9 , Issue 1 , June 1962 , pp. 49 - 53
Copyright
Copyright © University College London 1962

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Briggs, W. E. and Buschman, R. G., “The power series coefficients of functions defined by Dirichlet series”, Illinois J. of Math., 5 (1961), 43–44.CrossRefGoogle Scholar