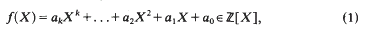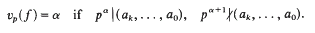Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kudryavtsev, M. V.
1993.
Estimation of a complete rational trigonometric sum. I.
Mathematical Notes,
Vol. 53,
Issue. 1,
p.
43.
Loh, W.K.A.
1994.
On Hau's lemma.
Bulletin of the Australian Mathematical Society,
Vol. 50,
Issue. 3,
p.
451.
Ding, Ping
1997.
On a Conjecture of Chalk.
Journal of Number Theory,
Vol. 65,
Issue. 1,
p.
116.
Cochrane, Todd
and
Zheng, Zhiyong
2001.
On upper bounds of Chalk and Hua for exponential sums.
Proceedings of the American Mathematical Society,
Vol. 129,
Issue. 9,
p.
2505.
Cochrane, Todd
and
Zheng, Zhiyong
2001.
Upper bounds on a two-term exponential sum*.
Science in China Series A: Mathematics,
Vol. 44,
Issue. 8,
p.
1003.
Cochrane, Todd
Liu, Chun Lei
and
Zheng, Zhi Yong
2003.
Upper Bounds on Character Sums with Rational Function Entries.
Acta Mathematica Sinica, English Series,
Vol. 19,
Issue. 2,
p.
327.
Ding, P.
2006.
On an exponential sum.
Journal of Mathematical Sciences,
Vol. 137,
Issue. 2,
p.
4645.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
195.
Cluckers, Raf
and
Nguyen, Kien Huu
2024.
Combining Igusa’s conjectures on exponential
sums and monodromy with semicontinuity of the minimal exponent.
Algebra & Number Theory,
Vol. 18,
Issue. 7,
p.
1275.
Liu, Huaning
and
Liu, Zehua
2024.
The sum-of-digits function in rings of residue classes.
Periodica Mathematica Hungarica,
Vol. 89,
Issue. 2,
p.
335.
Liu, H.
and
Liu, Z.
2024.
Truncated polynomials with restricted digits.
Acta Mathematica Hungarica,
Vol. 174,
Issue. 2,
p.
462.