Article contents
LARGE BIAS FOR INTEGERS WITH PRIME FACTORS IN ARITHMETIC PROGRESSIONS
Published online by Cambridge University Press: 15 February 2018
Abstract
We prove asymptotic formulas for the number of integers at most  $x$ that can be written as the product of
$x$ that can be written as the product of 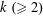 $k~({\geqslant}2)$ distinct primes
$k~({\geqslant}2)$ distinct primes 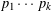 $p_{1}\cdots p_{k}$ with each prime factor in an arithmetic progression
$p_{1}\cdots p_{k}$ with each prime factor in an arithmetic progression 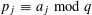 $p_{j}\equiv a_{j}\hspace{0.2em}{\rm mod}\hspace{0.2em}q$,
$p_{j}\equiv a_{j}\hspace{0.2em}{\rm mod}\hspace{0.2em}q$, 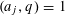 $(a_{j},q)=1$
$(a_{j},q)=1$ 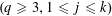 $(q\geqslant 3,1\leqslant j\leqslant k)$. For any
$(q\geqslant 3,1\leqslant j\leqslant k)$. For any 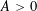 $A>0$, our result is uniform for
$A>0$, our result is uniform for 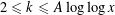 $2\leqslant k\leqslant A\log \log x$. Moreover, we show that there are large biases toward certain arithmetic progressions
$2\leqslant k\leqslant A\log \log x$. Moreover, we show that there are large biases toward certain arithmetic progressions 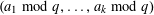 $(a_{1}\hspace{0.2em}{\rm mod}\hspace{0.2em}q,\ldots ,a_{k}\hspace{0.2em}{\rm mod}\hspace{0.2em}q)$, and such biases have connections with Mertens’ theorem and the least prime in arithmetic progressions.
$(a_{1}\hspace{0.2em}{\rm mod}\hspace{0.2em}q,\ldots ,a_{k}\hspace{0.2em}{\rm mod}\hspace{0.2em}q)$, and such biases have connections with Mertens’ theorem and the least prime in arithmetic progressions.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2018
References
- 4
- Cited by


