Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Loxton, J. H.
1983.
The graphs of exponential sums.
Mathematika,
Vol. 30,
Issue. 2,
p.
153.
Loxton, J. H.
1985.
The distribution of exponential sums.
Mathematika,
Vol. 32,
Issue. 1,
p.
16.
Coutsias, Evangelos A.
and
Kazarinoff, Nicholas D.
1987.
Disorder, renormalizability, theta functions and Cornu spirals.
Physica D: Nonlinear Phenomena,
Vol. 26,
Issue. 1-3,
p.
295.
Berry, M V
and
Goldberg, J
1988.
Renormalisation of curlicues.
Nonlinearity,
Vol. 1,
Issue. 1,
p.
1.
Mendès France, Michel
1991.
Fractal Geometry and Analysis.
p.
325.
Coutsias, E.
and
Kazarinoff, N.
1998.
The approximate functional formula for the theta function and Diophantine Gauss sums.
Transactions of the American Mathematical Society,
Vol. 350,
Issue. 2,
p.
615.
Evans, Ronald
Minei, Marvin
and
Yee, Bennet
2003.
Incomplete higher-order Gauss sums.
Journal of Mathematical Analysis and Applications,
Vol. 281,
Issue. 2,
p.
454.
Paris, R.B.
2003.
On the asymptotic connection between two exponential sums.
Journal of Computational and Applied Mathematics,
Vol. 157,
Issue. 2,
p.
297.
Paris, R.B.
2005.
An asymptotic approximation for incomplete Gauss sums.
Journal of Computational and Applied Mathematics,
Vol. 180,
Issue. 2,
p.
461.
Patterson, Samuel James
2007.
The Shaping of Arithmetic after C. F. Gauss’s Disquisitiones Arithmeticae.
p.
505.
Paris, R.B.
2008.
An asymptotic approximation for incomplete Gauss sums. II.
Journal of Computational and Applied Mathematics,
Vol. 212,
Issue. 1,
p.
16.
Paris, R.B.
2009.
The asymptotics of a new exponential sum.
Journal of Computational and Applied Mathematics,
Vol. 223,
Issue. 1,
p.
314.
Demirci Akarsu, Emek
and
Marklof, Jens
2013.
THE VALUE DISTRIBUTION OF INCOMPLETE GAUSS SUMS.
Mathematika,
Vol. 59,
Issue. 2,
p.
381.
Li, Kezhi
Gan, Lu
and
Ling, Cong
2013.
Convolutional Compressed Sensing Using Deterministic Sequences.
IEEE Transactions on Signal Processing,
Vol. 61,
Issue. 3,
p.
740.
Demirci Akarsu, Emek
2014.
Short incomplete Gauss sums and rational points on metaplectic horocycles.
International Journal of Number Theory,
Vol. 10,
Issue. 06,
p.
1553.
Korolev, M. A.
2015.
On incomplete Gaussian sums.
Proceedings of the Steklov Institute of Mathematics,
Vol. 290,
Issue. 1,
p.
52.
Chamizo, Fernando
and
Raboso, Dulcinea
2015.
Van der Corput method and optical illusions.
Indagationes Mathematicae,
Vol. 26,
Issue. 5,
p.
723.
Kowalski, Emmanuel
and
Sawin, William F.
2016.
Kloosterman paths and the shape of exponential sums.
Compositio Mathematica,
Vol. 152,
Issue. 7,
p.
1489.
Vogt, Trenton
and
Ulness, Darin J.
2019.
Cornu Spirals and the Triangular Lacunary Trigonometric System.
Fractal and Fractional,
Vol. 3,
Issue. 3,
p.
40.
Mork, L.K.
Vogt, Trenton
Sullivan, Keith
Rutherford, Drew
and
Ulness, Darin J.
2019.
Exploration of Filled-In Julia Sets Arising from Centered Polygonal Lacunary Functions.
Fractal and Fractional,
Vol. 3,
Issue. 3,
p.
42.
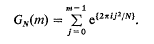
 . A sharp estimate is given for max |GN(m)|, depending on the residue of N modulo 4. The results were suggested by graphs of GN(m) made for N near 1000. The analysis employs the Fresnel integrals and the Cornu spiral whose curvature is proportional to its arc length.
. A sharp estimate is given for max |GN(m)|, depending on the residue of N modulo 4. The results were suggested by graphs of GN(m) made for N near 1000. The analysis employs the Fresnel integrals and the Cornu spiral whose curvature is proportional to its arc length.

