Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T05:46:19.380Z Has data issue: false hasContentIssue false
  • English
  • Français

Generic well-posedness of optimization problems in topological spaces

Part of: Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  26 February 2010

M. M. Čoban ,
P. S. Kenderov  and
J. P. Revalski
M. M. Čoban
Affiliation:
Moldavian SSR, 90 Odesskaia Str. kv 50, 278 000 Tiraspol, U.S.S.R.
P. S. Kenderov
Affiliation:
Institute of Mathematics, Bulgarian Academy of Sciences, “Acad. G. Bonchev” Str, Block 8, 1113 Sofia, Bulgaria.
J. P. Revalski
Affiliation:
Institute of Mathematics, Bulgarian Academy of Sciences, “Acad. G. Bonchev” Str, Block 8, 1113 Sofia, Bulgaria.
Get access

Abstract

Let X be a completely regular Hausdorff topological space and let C(X) (the set of all real-valued bounded and continuous in X functions) be endowed with the sup-norm. Let ßX, as usual, denotes the Stone-Čech compactification of X. We give a characterization of those X for which the set

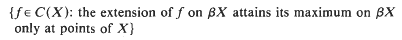
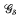contains a dense -subset of C(X). These are just the spaces X which contain a dense Čech complete subspace. We call such spaces almost Čech complete. We also prove that X contains a dense completely metrizable subspace, if, and only if, C(X) contains a dense 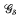-subset of functions which determine Tykhonov well-posed optimization problems over X. For a compact Hausdorff topological space X the latter result was proved by Čoban and Kenderov [CK1.CK2]. Relations between the well-posedness and Gâteaux and Fréchet differentiability of convex functionals in C(X) are investigated. In particular it is shown that the sup-norm in C(X) is Frechet differentiable at the points of a dense 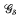-subset of C(X), if, and only if, the set of isolated points of X is dense in X. Conditions and examples are given when the set of points of Gateaux differentiability of the sup-norm in C(X) is a dense and Baire subspace of C(X) but does not contain a dense -subset of C(X).

MSC classification

Secondary: 46B20: Geometry and structure of normed linear spaces
Type
Research Article
Information
Mathematika , Volume 36 , Issue 2 , December 1989 , pp. 301 - 324
Copyright
Copyright © University College London 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

A.Arhangel'skiǐ, A. V.. Compact sets and topology of spaces. Trud. Moscow Math. Soc, 13 (1965), 3–55 (in Russian).Google Scholar
AP.Arhangel'skiǐ, A. V. and Ponomarev, V. I.. Foundations of General Topology in Problems and Exercises. Moscow, “Nauka” (1974) (in Russian).Google Scholar
As.Asplund, E.. Fréchet differentiability of convex functions. ActaMatk, 121 (1968), 31–47.Google Scholar
B.Beer, G.. On a generic optimization theorem of Petar Kenderov. Nonlinear Analysis, 12 (1988), 647–655.CrossRefGoogle Scholar
Cr.Christensen, J. P. R.. Theorems of I. Namioka and R. E. Jonson type for upper semicontinuous and compact-valued set-valued mappings. Proc. Amer. Math. Soc, 86 (1982), 649–655.Google Scholar
CrK.Christensen, J. P. R. and Kenderov, P. S.. Dense strong continuity of mappings and the Radon-Nikodym property. Math. Scand., 54 (1984), 70–78.CrossRefGoogle Scholar
CK1.Čoban, M. M. and Kenderov, P. S.. Dense Gâteaux differentiability of the sup-norm in C(T) and the topological properties of T. Compt. rend. Acad. bulg. Sci., 38 (1985), 1603–1604.Google Scholar
CK2.Čoban, M. M. and Kenderov, P. S.. Generic Gâteaux differentiability of convex functionals in C(T) and the topological properties of T. Math, and Education in Math., Proc. of the XV-th Spring Conf. of the Union of Bulg. Mathematicians (Sunny Beach, April, 1986), 141–149.Google Scholar
DM.De Blasi, F. S. and Myjak, J.. Some generic properties in convex and nonconvex optimization theory. Ann. Soc. Math. Polonae, Ser. I: Comm. Math., 24 (1984), 1–14.Google Scholar
DZ.Dontchev, A. and Zolezzi, T.. Well-Posed Optimization Problems. To appear in Lecture Notes in Mathematics (Springer).Google Scholar
En.Engelking, R.. General Topology (Warsaw, PWN, 1977).Google Scholar
FV.Furi, M. and Vignoli, A.. About well-posed minimization problems for functionals in metric spaces. J. Opt. Theory and Appl., 5 (1970), 225–229.CrossRefGoogle Scholar
K1.Kenderov, P. S.. Most of optimization problems have unique solution. Compt. rend. Acad. bulg. Sci., 37 (1984), 297–299.Google Scholar
K2.Kenderov, P. S.. Most of optimization problems have unique solution. International Series of Numerical Mathematics, 72 (Birkhauser, Basel, 1984), 203–216.CrossRefGoogle Scholar
LPh.Larman, D. G. and Phelps, R. R.. Gâteaux differentiability of convex functions on Banach spaces. j. London Math. Soc, 20 (1979), 115–127.CrossRefGoogle Scholar
LP.Lucchetti, R. and Patrone, F.. Sulla densita e genericita di alcuni problemi di minimo ben posti. Boll. U.M.I. (5), 15-B (1978), 225–240.Google Scholar
NPh.Namioka, I. and Phelps, R. R.. Banach spaces which are Asplund spaces. Duke Math. J., 42 (1975), 735–749.CrossRefGoogle Scholar
P.Patrone, F.. Well-posedness as an ordinal property. Rivista di Mat. pura ed appl., 1 (1987), 95–104.Google Scholar
Rl.Revalski, J. P.. Generic properties concerning well-posed optimization problems. Compt. rend. Acad. bulg. Sci., 38 (1985), 1431–1434.Google Scholar
R2.Revalski, J. P.. Generic well-posedness in some classes of optimization problems. Acta Univ. Carolinae-Math. et Phys., 28 (1987), 117–125.Google Scholar
R3.Revalski, J. P.. Well-posedness almost everywhere in a class of constrained convex optimization problems. Math, and Education in Math., Proc. of the 17th Spring Conf. of the Union of the Bulgarian Math. (Sunny Beach, April 1988), 348–353.Google Scholar
S1.Stegall, C.. A class of topological spaces and differentiation of functions in Banach spaces. Proc. of the Conf. on Vector Measure and Integral representations of operators and on Functional Analysis (Banach space theory) (Esen 1982) Vorlesungen Fachbereich Math. Univ. Essen, 10 (1983), 63–67.Google Scholar
S2.Stegall, C.. Topological spaces with dense subspaces that are homeomorphic to complete metric spaces and the classification of C(K) Banach spaces. Mathematika, 34 (1987), 101–107.CrossRefGoogle Scholar
Tl.Talagrand, M.. Deux example de fonctions convexes. C.R. Acad. Sci. Paris, 288, A, (1979), 461–464.Google Scholar
T.Tykhonov, A. N.. On the stability of the functional optimization problem. USSR J. of Comp. Math, and Math. Physics, 6 (1966), 631–634 (in Russian).Google Scholar