No CrossRef data available.
Published online by Cambridge University Press: 05 February 2023
In this paper, we mainly study the function spaces related to H-sober spaces. For an irreducible subset system H and
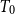 $T_{0}$
spaces X and Y, it is proved that the following three conditions are equivalent: (1) the Scott space
$T_{0}$
spaces X and Y, it is proved that the following three conditions are equivalent: (1) the Scott space
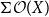 $\Sigma \mathcal O(X)$
of the lattice of all open sets of X is H-sober; (2) for every H-sober space Y, the function space
$\Sigma \mathcal O(X)$
of the lattice of all open sets of X is H-sober; (2) for every H-sober space Y, the function space
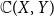 $\mathbb{C}(X, Y)$
of all continuous mappings from X to Y equipped with the Isbell topology is H-sober; (3) for every H-sober space Y, the Isbell topology on
$\mathbb{C}(X, Y)$
of all continuous mappings from X to Y equipped with the Isbell topology is H-sober; (3) for every H-sober space Y, the Isbell topology on
 $\mathbb{C}(X, Y)$
has property S with respect to H. One immediate corollary is that for a
$\mathbb{C}(X, Y)$
has property S with respect to H. One immediate corollary is that for a
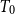 $T_{0}$
space X, Y is a d-space (resp., well-filtered space) iff the function space
$T_{0}$
space X, Y is a d-space (resp., well-filtered space) iff the function space
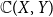 $\mathbb{C}(X, Y)$
equipped with the Isbell topology is a d-space (resp., well-filtered space). It is shown that for any
$\mathbb{C}(X, Y)$
equipped with the Isbell topology is a d-space (resp., well-filtered space). It is shown that for any
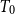 $T_0$
space X for which the Scott space
$T_0$
space X for which the Scott space
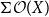 $\Sigma \mathcal O(X)$
is non-sober, the function space
$\Sigma \mathcal O(X)$
is non-sober, the function space
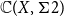 $\mathbb{C}(X, \Sigma 2)$
equipped with the Isbell topology is not sober. The function spaces
$\mathbb{C}(X, \Sigma 2)$
equipped with the Isbell topology is not sober. The function spaces
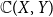 $\mathbb{C}(X, Y)$
equipped with the Scott topology, the compact-open topology and the pointwise convergence topology are also discussed. Our study also leads to a number of questions, whose answers will deepen our understanding of the function spaces related to H-sober spaces.
$\mathbb{C}(X, Y)$
equipped with the Scott topology, the compact-open topology and the pointwise convergence topology are also discussed. Our study also leads to a number of questions, whose answers will deepen our understanding of the function spaces related to H-sober spaces.
This research was supported by the National Natural Science Foundation of China (Nos. 12071199, 11661057), and the Science and Technology Project of Education Bureau of Hebei Province, China (No. QN2023023).