Let d be a square-free positive integer and let O (= Od) be the ring of integers of the imaginary quadratic number field ℚ(-d). The groups PSL2(O) are called the Bianchi groups after Luigi Bianchi who made the first important contribution 1 to their study in 1892. Since then they have attracted considerable attention particularly during the last thirty years. Their importance stems primarily from their action as discrete groups of isometries on hyperbolic 3-space, H3. As a consequence they play an important role in hyperbolic geometry, low-dimensional topology together with the theory of discontinuous groups and automorphic forms. In addition they are of particular significance in the class of linear groups over Dedekind rings of arithmetic type. Serre9 has proved that in this class the Bianchi groups (along with, for example, the modular group, PSL2(z), where z is the ring of rational integers) have an exceptionally complicated (non-congruence) subgroup structure.

An arithmetic Fuchsian group is necessarily of finite covolume and so of the first kind. From the structure theorem for finitely generated Fuchsian groups those of the first kind which can be generated by two elements are triangle groups, groups of signature (1;q;0) or (1; ; 1) or groups of signature (0;2,2,2,e;0) where e is odd 6. It is known that there are finitely many conjugacy classes of arithmetic groups with these signatures or indeed with any fixed signature 3, 15. In the case of non-cocompact groups, the arithmetic groups are conjugate to groups commensurable with the classical modular group and are easily determined. For the other groups described above the space of conjugacy classes of all such Fuchsian groups of fixed signature can be described in terms of the traces of a pair of generating elements and their product. In the case of triangle groups this space is a single point. This description has been utilized to determine all classes of triangle groups 13 and groups of signature (1;q;0) which are arithmetic 15. In this paper we determine all classes of groups of signature (0;2,2,2,e;0) with e odd which are arithmetic. The techniques involving traces used so profitably in 15 are not so fruitful in this case. Consequently we have in the main resorted to a quite different method which does not rely on having a precise description of the space of conjugacy classes and hence could be applicable to groups other than those which have rank 2. Extensive use is made of results of Borell on the structure of arithmetic Fuchsian groups which require detailed information on the number fields defining the quaternion algebras. Consequently, the results are given in terms of the quaternion algebra which is determined by its defining field and ramification set, and maximal orders in that quaternion algebra.

In 5, I provided a method whereby the study of an Artinian module A over a commutative ring R (throughout the paper, R will denote a commutative ring with identity) can, for some purposes at least, be reduced to the study of an Artinian module A' over a complete (Noetherian) local ring; in the latter situation, Matlis' duality 1 (alternatively, see 6, ch. 5) is available, and this means that the investigation can often be converted into a dual one about a finitely generated module over a complete (Noetherian) local ring.

This note is a brief excursion into a new theory of modules over a commutative ring R modulo a Serre subcategory S of the category of R-modules, in the sense that the modules of S are regarded as trivial. As a demonstration of the theory we have chosen to extend the primary decomposition theorem for submodules of a Noetherian .R-module from the familiar case of S trivial (i.e. the only R-module of S is the zero module) to the general case.

Throughout, (R, m) will denote a d-dimensional CohenMacaulay (CM for short) local ring having an infinite residue field and I an m-primary ideal in R. Recall that an ideal JI is said to be a reduction of I if Ir+1 = JIr for some r0, and a reduction J of I is called a minimal reduction of I if J is generated by a system of parameters. The concepts of reduction and minimal reduction were first introduced by Northcott and Rees12. If J is a reduction of I, define the reduction number of I with respect to J, denoted by rj(I), to be min {r0 Ir+1 = JIr}. The reduction number of I is defined as r(I) = min {rj(I)J is a minimal reduction of I}. The reduction number r(I) is said to be independent if r(I) = rj(I) for every minimal reduction J of I.

Intuitively, transfinite iteration is a repetitive process, which eventually reaches completion, but might need to progress through an infinite chain of steps before finally doing so. But whereas such a chain is always readily at hand in classical set theory in the form of ordinals, iterative arguments involving sets (i.e. objects) in a general topos have to depend on some intrinsic or naturally available inductive structure, say algebraic, which might not be associated with a (well-ordered) chain.

We introduce a constructively weak variant of compactness for locales as a supplement to weak closure, a notion which has featured in the constructive formulation of some recent results in locale theory.

In this paper we are concerned with questions about the knottedness of a closed curve of given length embedded in Z3. What is the probability that such a randomly chosen embedding is knotted? What is the probability that the embedding contains a particular knot? What is the expected complexity of the knot? To what extent can these questions also be answered for a graph of a given homeomorphism type?

We use a pattern theorem due to Kesten 12 to prove that almost all embeddings in Z3 of a sufficiently long closed curve contain any given knot. We introduce the idea of a good measure of knot complexity. This is a function F which maps the set of equivalence classes of embeddings into 0, ). The F measure of the unknot is zero, and, generally speaking, the more complex the prime knot decomposition of a given knot type, the greater its F measure. We prove that the average value of F diverges to infinity as the length (n) of the embedding goes to infinity, at least linearly in n. One example of a good measure of knot complexity is crossing number.

Finally we consider similar questions for embeddings of graphs. We show that for a fixed homeomorphism type, as the number of edges n goes to infinity, almost all embeddings are knotted if the homeomorphism type does not contain a cut edge. We prove a weaker result in the case that the homeomorphism type contains at least one cut edge and at least one cycle.

In this paper we extend ideas developed in 2, 4 to study certain minimal immersions of S2 and ℝP2 into ℂPn. Here S2 denotes the unit sphere in ℝ3 with its standard conformal structure and ℝP2 is S2 factored out by the antipodal map, while ℂPn denotes complex projective n-space equipped with the FubiniStudy metric of constant holomorphic sectional curvature 4. Since ℝPn with its standard metric of constant curvature 1 is included in ℂPn as a totally geodesic submanifold, this includes the case of minimal immersions into the unit sphere Sn(1) with its standard metric.

In this paper, we classify generic isotropic map-germs arising from the symplectic reduction process relative to a hypersurface (i.e. one-dimensional reduction), up to symplectic equivalence in the C category. These models are open Whitney umbrellas of arbitrary dimension and their suspensions. These singularities appear in the generalized Cauchy problem for HamiltonJacobi equations.

Let G denote a compact connected abelian group with character groupand normalized Haar measure . As a consequence of the duality theorems (11, theorem 2518),is torsion-free and hence can be ordered. That is, there is a sub-semigroup P ofsuch that

In recent years many mathematicians have studied the semigroups S, the Stone-ech compactification of the discrete semigroup S, and more particularly ℕ, where ℕ is the usual semigroup of positive integers with addition (see the surveys by Hindman and Pym 6). An important role in this theory is played by sequences in S which have distinct finite sums, for these sequences are closely linked to idempotents in S (see the survey by Hindman 6). Pym 8 introduced the concept of an oid in order to show that the structure of ℕ in 5 could be obtained in a simple way for a large class of semigroups. Papazyan 7 pointed out that the theories of sequences with distinct finite sums and of oids are the same. Thus oids have a central position in the theory of semigroup compactifications.

Let K be a compact Hausdorif space, X a Banach space and C(K, X) the Banach space of all continuous functions : KX equipped with the supremum norm. A subset H of C(K, X) is pointwise weakly precompact if, for each t in K, the set Ht) = {(t):H} is weakly precompact. In this note we study the images of a bounded pointwise weakly precompact subset H of C(K, X) under several classes of linear operators on C(K, X).

Given vectors x and y in a Hilbert space, an interpolating operator is a bounded operator T such that Tx = y. An interpolating operator for n vectors satisfies the equation Txt = yt, for i = 1, 2,, n. In this article, we continue the investigation of the one-vector interpolation problem for nest algebras that was begun by Lance. In particular, we require the interpolating operator to belong to certain ideals which have proved to be of importance in the study of nest algebras, namely, the compact operators, the radical, Larson's ideal, and certain other ideals. We obtain necessary and sufficient conditions for interpolation in each of these cases.

Suppose that A is a Banach algebra and let A be the second dual algebra of A equipped with the first Arens product 3. In this paper we characterize compact and weakly compact multipliers of A, when A possesses a bounded approximate identity and is a two sided ideal in A. We use this to study the isomorphisms between second duals of various classes of Banach algebras satisfying the above properties.

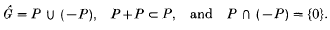
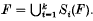A family {S1, ,Sk} of contracting affine transformations on Rn defines a unique non-empty compact set F satisfying . We obtain estimates for the Hausdorff and box-counting dimensions of such sets, and in particular derive an exact expression for the box-counting dimension in certain cases. These estimates are given in terms of the singular value functions of affine transformations associated with the Si. This paper is a sequel to 4, which presented a formula for the dimensions that was valid in almost all cases.

An amenable semigroup of positive linear unital mappings on a W*-algebra is considered. Two main questions are dealt with: the existence of a normal faithful state invariant with respect to this semigroup and the description of ergodicity conditions. An explicit form of the ergodic projection, useful in treating the ergodicity problems, is also derived.