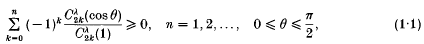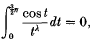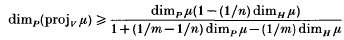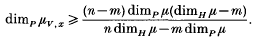Research Article
Marked homeomorphisms and the realization problem
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 575-596
-
- Article
- Export citation
A natural invariant algebra for the Harada-Norton group
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 597-614
-
- Article
- Export citation
All automorphisms of free groups with maximal rank fixed subgroups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 615-630
-
- Article
- Export citation
Betweenness relations and cycle-free partial orders
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 631-643
-
- Article
- Export citation
Fundamental groups of 4-manifolds with circle actions†
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 645-655
-
- Article
- Export citation
Isomorphisms between the second duals of group algebras of locally compact groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 657-663
-
- Article
- Export citation
Towards a higher-dimensional MacLane class
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 665-671
-
- Article
- Export citation
Harmonic typically real mappings
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 673-680
-
- Article
- Export citation
Positivity of more Jacobi polynomial sums
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 681-694
-
- Article
- Export citation
The packing dimension of projections and sections of measures
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 695-713
-
- Article
- Export citation
On Hausdorff and packing dimension of product spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 715-727
-
- Article
- Export citation
Beurling's projection theorem via one-dimensional Brownian motion
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 729-738
-
- Article
- Export citation
Cosmological solutions of the Vlasov-Einstein system with spherical, plane, and hyperbolic symmetry
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 739-762
-
- Article
- Export citation
Front matter
PSP volume 119 issue 4 Cover and Front matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
PSP volume 119 issue 4 Cover and Back matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. b1-b7
-
- Article
-
- You have access
- Export citation

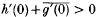 and is not typically real. Furthermore, we give a geometric characterization for univalent, harmonic and typically real mappings.
and is not typically real. Furthermore, we give a geometric characterization for univalent, harmonic and typically real mappings.