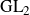Hostname: page-component-5cf477f64f-2wr7h Total loading time: 0 Render date: 2025-04-02T06:24:04.297Z Has data issue: false hasContentIssue false
  • English
  • Français

A zero density estimate and fractional imaginary parts of zeros for $\textrm{GL}_2$ L-functions

Part of: Zeta and $L$-functions: analytic theory

Published online by Cambridge University Press:  28 December 2022

OLIVIA BECKWITH ,
DI LIU ,
JESSE THORNER  and
ALEXANDRU ZAHARESCU
OLIVIA BECKWITH
Affiliation:
Mathematics Department, Tulane University, New Orleans, 6823 St. Charles Ave., LA 70118, U.S.A. e-mail: [email protected]
DI LIU
Affiliation:
Department of Mathematics, University of Illinois at Urbana–Champaign, 1409 West Green Street, Urbana, IL 61801, U.S.A. e-mails: [email protected], [email protected]
JESSE THORNER
Affiliation:
Department of Mathematics, University of Illinois at Urbana–Champaign, 1409 West Green Street, Urbana, IL 61801, U.S.A. e-mails: [email protected], [email protected]
ALEXANDRU ZAHARESCU
Affiliation:
Department of Mathematics, University of Illinois at Urbana–Champaign, 1409 West Green Street, Urbana, IL 61801, U.S.A. and Simion Stoilow Institute of Mathematics of the Romanian Academy, P.O. Box 1-764, RO-014700 Bucharest, Romania. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove an analogue of Selberg’s zero density estimate for 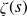$\zeta(s)$ that holds for any 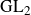$\textrm{GL}_2$ L-function. We use this estimate to study the distribution of the vector of fractional parts of 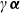$\gamma\boldsymbol{\alpha}$ , where 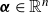$\boldsymbol{\alpha}\in\mathbb{R}^n$ is fixed and 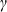$\gamma$ varies over the imaginary parts of the nontrivial zeros of a 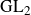$\textrm{GL}_2$ L-function.

MSC classification

Primary: 11M41: Other Dirichlet series and zeta functions
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 174 , Issue 3 , May 2023 , pp. 605 - 630
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Cambridge Philosophical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andersen, N. and Thorner, J.. Zeros of $\textrm{GL}_2$ L-functions on the critical line. Forum Math. 33 (2021), no. 2, 477–491.Google Scholar
Alan, S. Baluyot, C.. On the Zeros of Riemann’s Zeta-function. (ProQuest LLC, Ann Arbor, MI, 2017). PhD. thesis. University of Rochester.Google Scholar
Blomer, V. and Brumley, F.. On the Ramanujan conjecture over number fields. Ann. of Math. (2) 174 (2011), no. 1, 581–605.Google Scholar
Bernard, D.. Modular case of Levinson’s theorem. Acta Arith. 167 (2015), no. 3, 201–237.Google Scholar
Bombieri, E. and Hejhal, D. A.. On the distribution of zeros of linear combinations of Euler products. Duke Math. J. 80 (1995), no. 3, 821–862.Google Scholar
Barnet-Lamb, T., Geraghty, D., Harris, M. and Taylor, R.. A family of Calabi-Yau varieties and potential automorphy II. Publ. Res. Inst. Math. Sci. 47 (2011), no. 1, 29–98.Google Scholar
Das, M.. Selberg’s Central Limit Theorem for L-functions of level aspect. (2020), arXiv:2012.10766.Google Scholar
Ford, K., Harper, A. J. and Lamzouri, Y.. Extreme biases in prime number races with many contestants. Math. Ann. 374 (2019), no. 1-2, 517–551.Google Scholar
Ford, K. and Konyagin, S.. The prime number race and zeros of L-functions off the critical line. Duke Math. J. 113 (2002), no. 2, 313–330.Google Scholar
Ford, K., Lamzouri, Y. and Konyagin, S.. The prime number race and zeros of Dirichlet L-functions off the critical line: Part III. Q. J. Math. 64 (2013), no. 4, 1091–1098.Google Scholar
Fiorilli, D. and Martin, G.. Inequities in the Shanks–Rényi prime number race: an asymptotic formula for the densities. J. Reine Angew. Math. 676 (2013), 121–212.Google Scholar
Ford, K., Meng, X. and Zaharescu, A.. Simultaneous distribution of the fractional parts of Riemann zeta zeros. Bull. Lond. Math. Soc. 49 (2017), no. 1, 1–9.Google Scholar
Ford, K., Soundararajan, K. and Zaharescu, A.. On the distribution of imaginary parts of zeros of the Riemann zeta function. II. Math. Ann. 343 (2009), no. 3, 487–505.Google Scholar
Ford, K. and Zaharescu, A.. On the distribution of imaginary parts of zeros of the Riemann zeta function. J. Reine Angew. Math. 579 (2005), 145–158.Google Scholar
Ford, K. and Zaharescu, A.. Unnormalized differences between zeros of L-functions. Compositio. Math. 151 (2015), no. 2, 230–252.Google Scholar
Harris, M., Potential automorphy of odd-dimensional symmetric powers of elliptic curves and applications, Algebra, arithmetic and geometry: in honor of Yu. I. Manin. Vol. II. Progr. Math., vol. 270 (Birkhäuser Boston, Boston, MA, 2009), pp. 1–21.Google Scholar
Hlawka, E.. Über die Gleichverteilung gewisser Folgen, welche mit den Nullstellen der Zetafunktion zusammenhängen. Österreich. Akad. Wiss. Math.-Naturwiss. Kl. S.-B. II 184 (1975), no. 8-10, 459–471.Google Scholar
Iwaniec, H. and Kowalski, E.. Analytic Number Theory . (American Mathematical Society Colloquium Publications, American Mathematical Society, 2004).Google Scholar
Jiang, Y., Lü, G. and Wang, Z.. Exponential sums with multiplicative coefficients without the Ramanujan conjecture. Math. Ann. 379 (2021), no. 1-2, 589–632.Google Scholar
Jutila, M.. Zeros of the Zeta-function Near the Critical Line. Stud. Pure Math., (Birkhäuser, Basel, 1983), pp. 385–394.CrossRefGoogle Scholar
Kemble, R. S.. A Groshev theorem for small linear forms. Mathematika 52 (2005), no. 1-2, 79–85 (2006).Google Scholar
Khintchine, A.. Einige Sätze über Kettenbrüche, mit Anwendungen auf die Theorie der Diophantischen Approximationen. Math. Ann. 92 (1924), no. 1-2, 115–125.Google Scholar
Kim, H. H.. Functoriality for the exterior square of $\textrm{GL}_4$ and the symmetric fourth of $\textrm{GL}_2$ . J. Amer. Math. Soc. 16 (2003), no. 1, 139–183, With appendix 1 by Dinakar Ramakrishnan and appendix 2 by Kim and Peter Sarnak.Google Scholar
Luo, W. Z.. Zeros of Hecke L-functions associated with cusp forms. Acta Arith. 71 (1995), no. 2, 139–158.Google Scholar
Liu, D. and Zaharescu, A.. Races with imaginary parts of zeros of the Riemann zeta function and Dirichlet L-functions. J. Math. Anal. Appl. 494 (2021), no. 1, 124591.Google Scholar
Montgomery, H. L. and Vaughan, R. C.. Hilbert’s inequality. J. London Math. Soc. (2) 8 (1974), 73–82.CrossRefGoogle Scholar
Ono, K., The web of modularity: arithmetic of the coefficients of modular forms and q-series, CBMS Regional Conference Series in Mathematics, vol. 102. Published for the Conference Board of the Mathematical Sciences, Washington, DC (American Mathematical Society, Providence, RI, 2004).CrossRefGoogle Scholar
Rubinstein, M. and Sarnak, P.. Chebyshev’s bias. Experiment. Math. 3 (1994), no. 3, 173–197.Google Scholar
Radziwiłł, M. and Soundararajan, K.. Selberg’s central limit theorem for $\log{|\zeta(1/2+it)|}$ . Enseign. Math. 63 (2017), no. 1-2, 1–19.Google Scholar
Rubinstein, M.. L-function c++ class library and the command line program lcalc. https://github.com/agrawroh/l-calc (2014).Google Scholar
Selberg, A., Old and new conjectures and results about a class of Dirichlet series. Proceedings of the Amalfi Conference on Analytic Number Theory (Maiori, 1989), University of Salerno (1992), pp. 367–385.Google Scholar
Thorner, J., Effective forms of the Sato-Tate conjecture, Res. Math. Sci. 8 (2021), no. 1, 4.Google Scholar
Titchmarsh, E. C., The Theory of Functions (Oxford University Press, Oxford, 1958). Reprint of the second (1939) edition.Google Scholar
Titchmarsh, E. C., The Theory of the Riemann Zeta-function, second ed. (The Clarendon Press, Oxford University Press, New York, 1986). Edited and with a preface by D. R. Heath–Brown.Google Scholar
Wong, P.-J., On the Chebotarev–Sato–Tate phenomenon , J. Number Theory 196 (2019), 272–290.CrossRefGoogle Scholar