No CrossRef data available.
Article contents
Sparseness for the symmetric hit problem at all primes
Published online by Cambridge University Press: 11 December 2014
Abstract
The hit problem for a module over the Steenrod algebra 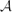 $\mathcal{A}$ seeks a minimal set of
$\mathcal{A}$ seeks a minimal set of 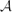 $\mathcal{A}$-generators (“non-hit elements,” those not in the image of positive degree elements of
$\mathcal{A}$-generators (“non-hit elements,” those not in the image of positive degree elements of 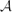 $\mathcal{A}$). This problem has been studied for 25 years in a variety of contexts and, although general or complete results have been difficult to obtain, partial results have been obtained in many cases.
$\mathcal{A}$). This problem has been studied for 25 years in a variety of contexts and, although general or complete results have been difficult to obtain, partial results have been obtained in many cases.
For any prime p ⩾ 2, consider the algebra of symmetric polynomials in l variables over  $\mathbb{F}$p (the cohomology of BU(l) [BO(l) for p = 2]). We prove a general sparseness result: For any l, the
$\mathbb{F}$p (the cohomology of BU(l) [BO(l) for p = 2]). We prove a general sparseness result: For any l, the 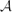 $\mathcal{A}$-generators can be concentrated in complex [real for p = 2] degrees τ such that α((p - 1) (τ + l)) ⩽(p - 1)l, where α(m) denotes the sum of the digits in the p-ary expansion of m.
$\mathcal{A}$-generators can be concentrated in complex [real for p = 2] degrees τ such that α((p - 1) (τ + l)) ⩽(p - 1)l, where α(m) denotes the sum of the digits in the p-ary expansion of m.
The specialisation of this result to p = 2 was proved by Janfada and Wood using different methods. Key ingredients of our approach are an action of the Kudo–Araki–May algebra on the 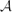 $\mathcal{A}$-primitives in homology, and a symmetric homology adaptation of the concept behind the χ-trick in cohomology.
$\mathcal{A}$-primitives in homology, and a symmetric homology adaptation of the concept behind the χ-trick in cohomology.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 158 , Issue 2 , March 2015 , pp. 269 - 274
- Copyright
- Copyright © Cambridge Philosophical Society 2014


