Some arithmetic properties of the Legendre polynomials
Published online by Cambridge University Press: 24 October 2008
Extract
1. Good (4) has proved the formula
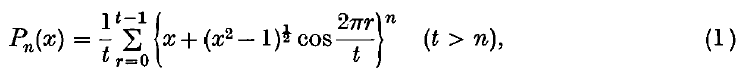
where Pn(x) is the Legendre polynomial of degree n and t is any integer greater than n. The form of the right-hand side suggests that (1) may be of use in deriving arithmetic properties of Pn(x).
Elsewhere (1) the writer indicated a connexion between divisibility properties of Pm(a) for special values of a and the complex multiplication of elliptic functions. If p = 2m + 1 is an odd prime, put

- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 53 , Issue 2 , April 1957 , pp. 265 - 268
- Copyright
- Copyright © Cambridge Philosophical Society 1957
References
REFERENCES
- 3
- Cited by


