Article contents
Regularity of Hausdorff measure function for conformal dynamical systems
Published online by Cambridge University Press: 17 February 2016
Abstract
We deal with the question of continuity of numerical values of Hausdorff measures in parametrised families of linear (similarity) and conformal dynamical systems by developing the pioneering work of Lars Olsen and the work [SUZ]. We prove Hölder continuity of the function ascribing to a parameter the numerical value of the Hausdorff measure of either the corresponding limit set or the corresponding Julia set. We consider three cases. Firstly, we consider the case of parametrised families of conformal iterated function systems in 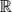 $\mathbb{R}$k with k ⩾ 3. Secondly, we consider all linear iterated function systems consisting of similarities in
$\mathbb{R}$k with k ⩾ 3. Secondly, we consider all linear iterated function systems consisting of similarities in 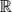 $\mathbb{R}$k with k ⩾ 1. In either of these two cases, the strong separation condition is assumed. In the latter case the Hölder exponent obtained is equal to 1/2. Thirdly, we prove such Hölder continuity for analytic families of conformal expanding repellers in the complex plane
$\mathbb{R}$k with k ⩾ 1. In either of these two cases, the strong separation condition is assumed. In the latter case the Hölder exponent obtained is equal to 1/2. Thirdly, we prove such Hölder continuity for analytic families of conformal expanding repellers in the complex plane  $\mathbb{C}$. Furthermore, we prove the Hausdorff measure function to be piecewise real–analytic for families of naturally parametrised linear IFSs in
$\mathbb{C}$. Furthermore, we prove the Hausdorff measure function to be piecewise real–analytic for families of naturally parametrised linear IFSs in 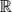 $\mathbb{R}$ satisfying the strong separation condition. On the other hand, we also give an example of a family of linear IFSs in
$\mathbb{R}$ satisfying the strong separation condition. On the other hand, we also give an example of a family of linear IFSs in 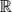 $\mathbb{R}$ for which this function is not even differentiable at some parameters.
$\mathbb{R}$ for which this function is not even differentiable at some parameters.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 160 , Issue 3 , May 2016 , pp. 537 - 563
- Copyright
- Copyright © Cambridge Philosophical Society 2016
References
REFERENCES
- 3
- Cited by


