Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-25T06:00:31.373Z Has data issue: false hasContentIssue false
  • English
  • Français

Ramanujan's Continued Fraction and the Bauer-Muir Transformation

Published online by Cambridge University Press:  24 October 2008

V. N. Singh
V. N. Singh
Affiliation:
Ramanujan Institute of MathematicsMadras-5, India
Get access Rights & Permissions [Opens in a new window]

Extract

Ramanujan's Continued Fraction may be stated as follows: Let where there are eight gamma functions in each product and the ambiguous signs are so chosen that the argument of each gamma function contains one of the specified number of minus signs. Then 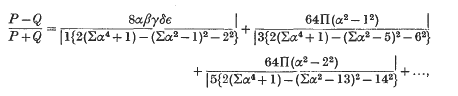where the products and the sums on the right range over the numbers α, β, γ, δ, ε: provided that one of the numbers β, γ, δ, ε is equal to ± ±n, where n is a positive integer. In 1935, Watson (3) proved the theorem by induction and also gave a basic analogue. In this paper we give a new proof of Ramanujan's Continued Fraction by using the transformation of Bauer and Muir in the theory of continued fractions (Perron (1), §7;(2), §2).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 57 , Issue 1 , January 1961 , pp. 76 - 79
Copyright
Copyright © Cambridge Philosophical Society 1961

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Perron, O., Die Lehre von den Kettenbrüchen, 3. Aufl., Band II, Teubners (Stuttgart), 1957.Google Scholar
(2)Perron, O., Über die Preece'schen Kettenbrüche. S.B. Akad. Wiss. Math. Wien, 1953, Nr. 3, 21–56.Google Scholar
(3)Watson, G. N., Ramanujan's Continued Fraction. Proc. Camb. Phil. Soc. 31 (1935), 7–17.CrossRefGoogle Scholar
(4)Watson, G. N., A theorem on continued fractions. Proc. Edin. Math. Soc. 11 (1959), 167–74.CrossRefGoogle Scholar